This paper is divided into four sections – A, B, C and D.
Answer all questions.
Section A consists of one question having sub-parts of one mark each.
Section B consists of seven questions of two marks each.
Section C consists of nine questions of three marks each, and
Section D consists of three questions of five marks each
.
Internal choices have been provided in two questions each in Section B,
Section C and Section D.
The intended marks for questions are given in brackets [ ].
All working, including rough work, should be done on the same sheet as and
adjacent to the rest of the answer.
Answers to sub parts of the same question must be given in one place only.
A list of useful physical constants is given at the end of this paper.
A simple scientific calculator without a programmable memory may be used for
calculations.
Section-A
This section comprises multiple choice questions (MCQs) of 1 mark each
Question
1
If the sum of zeroes of the polynomial p(x) = 2x2 - k√2x + 1 is √2,
then value of k is :
(a) √2
(b) 2
(c) 2√2
(d) 1/2
Solution
View Solution
Question
2
If the probability of a player winning a game is 0.79, then the
probability of his losing the same game is :
(a) 1.79
(b) 0.31
(c) 0.21%
(d) 0.21
Solution
View Solution
Question
3
If the roots of equation ax2 + bx + c = 0, a ≠ 0 are real and equal, then
which of the following relation is true ?
(a) a = b2/c
(b) b2 = ac
(c) ac = b2/4
(d) c = b2/a
Solution
View Solution
Question
4
In an A.P., if the first term a = 7, nth term an = 84 and the sum of first
n terms sn = 2093/2 then n is equal to :
(a) 22
(b) 24
(c) 23
(d) 26
Solution
View Solution
Question
5
If two positive integers p and q can be expressed as p = 18 a2b4 and
q =20 a3b2, where a and b are prime numbers, then LCM (p, q) is
(a) 2a2b2
(b) 180a2b2
(c) 12a2b2
(d) 180a3b4
Solution
View Solution
Question
6
AD is a median of ▵ABC with vertices A(5,-6), B(6, 4) and C(0, 0)
Length AD is equal to
(a) √68 units
(b) 2√15 units
(c) √101 units
(d) 10 units
Solution
View Solution
Question
7
If sec θ - tan θ = m, then the value of sec θ + tan θ is
(a) 1-(1/m)
(b) m2-1
(c) 1/m
(d) -m
Solution
View Solution
Question
8
From the data 1, 4, 7, 9, 16, 21, 25, if all the even numbers are
removed, then the probability of getting at random a prime number
from the remaining is :
(a) 2/5
(b) 1/5
(c) 1/7
(d) 2/7
Solution
View Solution
Question
9
For some data x1, x2, ... xn with respective frequencies f1,f2,...fn the value of 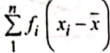
(a) nx
(b) 1
(c) ∑fi
(d) 0
Solution
View Solution
Question
10
The zeroes of a polynomial x2 + px + q are twice the zeroes of the
polynomial 4x2 - 5x - 6. The value of p is :
(a) -5/2
(b) 5/2
(c) -5
(d) 10
Solution
View Solution
Question
11
If the distance between the points (3, - 5) and (x,-5) is 15 units, then
the values of x are :
(a) 12,-18
(b) -12,18
(c) 18,5
(d) -9,-12
Solution
View Solution
Question
12
If cos(α+ β) = 0, then value of cos((α + β)/2) is equal to :
(a) 1/√2
(b) 1/2
(c) 0
(d) √2
Solution
View Solution
Question
13
A solid sphere is cut into two hemispheres. The ratio of the surface
areas of sphere to that of two hemispheres taken together, is :
(a) 1:1
(b) 1:4
(c) 2:3
(d) 3:2
Solution
View Solution
Question
14
The middle most observation of every data arranged in order is called :
(a) mode
(b) median
(c) mean
(d) deviation
Solution
View Solution
Question
15
The volume of the largest right circular cone that can be carved out
from a solid cube of edge 2 cm is :
(a) 4π/3 cu cm
(b) 5π/3 cu cm
(c) 8π/3 cu cm
(d) 2π/3 cu cm
Solution
View Solution
Question
16
Two dice are rolled together, The probabilities of getting sum of
numbers on the two dice as 2,3 or 5 is
(a) 7/36
(b) 11/36
(c) 5/36
(d) 4/9
Solution
View Solution
Question
17
The centre of a circle is at (2, 0). If one end of a diameter is at (6, 0),
then the other end is at :
(a) (0,0)
(b) (4,0)
(c) (-2,0)
(d) (-6,0)
Solution
View Solution
Question
18
In the given figure, graphs of two linear equations are
shown. The pair of these linear equations is :
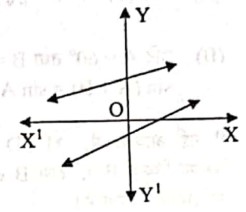
(a) consistent with unique solution,
(b) consistent with infinitely many-r solutions.
(c) inconsistent.
(d) inconsistent but can be made consistent
by extending these lines,
Solution
View Solution
Questions number 19 and 20 are Assertion and Reason based questions carrying
1 mark each. Two statements are given, one labelled as Assertion (A) and the
other is labelled as Reason (R). Select the correct answer to these questons from the codes (a), (b),
(c) and (d) as given below.
(a) both Assertion (A) and Reason (R) are true and Reason (R) is the
correct explanation of the Assertion (A).
(b) Both Assertion (A) and Reason (R) are true, but Reason (R) is not
the correct explanation of the Assertion (A).
(c) Assertion (A) is true, but Reason (R) is false.
(d) Assertion (A) is false, but Reason (R) is true.
Question
19
Assertion (A) : The tangents drawn at the end points of a diameter
of a circle, are parallel.
Reason (R): Diameter of a circle is the longest chord.
Solution
View Solution
Question
20
Assertion (A) : If the graph of a polynomial touches x-axis at only
one point, then the polynomial cannot be a quadratic
polynomial.
Reason (R): A polynomial of degree n(n >1) can have at most n
zeroes.
Solution
View Solution
Section-B
This section comprises very short answer (VSA) type questions of 2 marks each.
Question
21
Solve the following System of linear, equations
7x-2y = 5 and 8x + 7y = 15 verify your answer.
Solution
View Solution
Question
22
In pack of 52 playing cards one card is lost. From the remaining
cards, a card is drawn at random. Find the probability that the drawn card is queen of heart, if the lost card is a black card.
Solution
View Solution
Question
23
(A) Evaluate 2√2 cos 45o sin 30o + 2√3 cos 30o
OR
(B) If A= 60° and B = 30°, verify that
sin(A+B) = sinA cosB + cosA sinB
Solution
View Solution
Question
24
In the given figure. ABCD is a quadilateral
Diagonal BD bisects ∠B and ∠D both.
Prove that
(i) ▵ABD ~ ▵CBD
(ii) AB=BC
Solution
View Solution
Question
25
(A) Prove that 5 - 2√3 is an irrational number. It is given that √3
is an irrational number.
OR
(B) Show that the number 5 x 11 x 17 + 3 x 11 is a composite number.
Solution
View Solution
Section-C
This section comprises short answer (SA) type questions of 3 marks each.
Question
26
(A) Find the ratio in which the point (8/5, y) divides the line
segment joining the points (1, 2) and (2, 3). Also, find the value
of y.
OR
(B) ABCD is a rectangle formed by the points A(-1,-1),B(-1,6),
C(3,6)and D (3,-1).P,Q, R and S are mid-points of sides
AB, BC, CD and DA respectively. Show that diagonals of the
quadrilateral PORS bisect each other.
Solution
View Solution
Question
27
In a teachers' workshop, the number of teachers teaching French, Hindi and English are 48, 80 and 144 respectively. Find the minimum number of rooms required if in each room the same number of teachers are seated and all of them are of the same subject.
Solution
View Solution
Question
28
Question
29
Three years ago, Rashmi was thrice as old as Nazma. Ten years later,
Rashmi will be twice as old as Nazma. How old are Rashmi and Nazma now ?
Solution
View Solution
Question
30
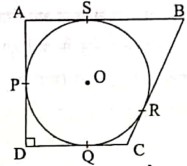
(A) In the given figure, AB is a diameter of the circle with centre O. AQ, BP and PQ are tangents to the circle. Prove that ∠POQ = 90°.
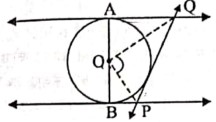
OR
(B) A circle with centre O and radius 8 cm
is inscribed in a quadrilateral ABCD in which P, Q, R, S are the points of contact as shown. If AD is perpendicular to DC, BC = 30 cm and BS=24 cm, then find the length DC.
Solution
View Solution
Question
31
The difference between the outer and inner radii of a hollow right
circular cylinder of length 14 cm is 1 cm. If the volume of the metal
used in making the cylinder is 176 cm3, find the outer and inner radii
of the cylinder.
Solution
View Solution
Section-D
This section comprises long answer (LA) type questions of 5 marks eac h.
Question
32
An arc of a circle of radius 21 cm subtends an angle of 60° at the
centre. Find :
(i) the length of the arc.
(ii) the area of the minor segment of the circle made by the
corresponding chord.
Solution
View Solution
Question
33
(A) The sum of first and eighth terms of an A.P. is 32 and their
product is 60. Find the first term and common difference of the
A.P. Hence, also find the sum of its first 20 terms.
OR
(B) In an A.P. of 40 terms, the sum of first 9 terms is 153 and the
sum of last 6 terms is 687. Determine the first term and
common difference of A.P. Also, find the sum of all the terms of the A.P.
Solution
View Solution
Question
34
(A) If a line is drawn parallel to one side of a triangle to intersect the other two sides in distinct points, then prove that the other two sides are divided in the same ratio.
OR
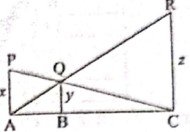
(B) In the given figure PA, QB and RC are each perpendicular to AC. If AP = x, BQ = y and CR = z
Prove that :
1/x + 1/z = 1/y then
Solution
View Solution
Question
35
A pole 6m high is fixed on the top of a tower. The angle of elevation
of the top of the pole observed from a point P on the ground is 60°
and the angle depression of the point P from the top of the tower is
45°. Find the height of the tower and the distance of point P from the
foot of the tower. (Use √3 =1.73)
Solution
View Solution
Section-E
This section comprises 3 case study based questions of 4 marks each.
Question
36
Case Study-I
A rectangular floor area can be
completely tiled with 200 square
tiles. If the side length of each tile is
increased by 1 unit, it would take
only 128 tiles to cover the floor.
(i) Assuming the original length of each side of a ule be x units, make a quadratic equation from the above information.
(ii) Write the corresponding quadratic equation in standard form.
(iii) (a) Find the value of x, the length of side of a tile by factorisation.
OR
(b) Solve the quadratic equation for x, using quadratic formula.
Solution
View Solution
Question
37
Case Study -2
The
BINGO is game of chance. host has 75 balls numbered 1 through 75. Each
player has a BINGO card with
some numbers written on it.
The participant cancels the number on the card when called out a number written on the ball selected at random. Whosoever cancels all the numbers on his/her card, says BINGO and wins the game. 48 balls were used before Tara said "BINGO".
The table given below, shows the data of one such game where
Based on the above information, answer the following
(i) Write the median class
(ii) When first ball was picked up, what was the probability of calling out an even number?
(iii) (a) Find median of the given data.
OR
(b) Find mode of the given data.
Solution
View Solution
Question
38
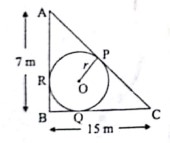
A backyard is in the shape of a triangle ABC with right angle at B.
AB = 7m and BC = 15 m. A circular pit was dug inside 1t such that it
touches the walls AC, BC and AB at P, Q and R respectively such
that AP = xm

Based on the above information, answer the following questions
(i) Find the length of AR in terms of x.
(ii) Write the type of quadrilateral BQOR.
(iii) (a) Find the length PC in terms of x and hence find the value
of x.
OR
(b) Find x and hence find the radius r of circle.
Solution
View Solution
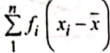

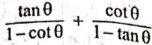 =
1+ secθ cosecθ
=
1+ secθ cosecθ