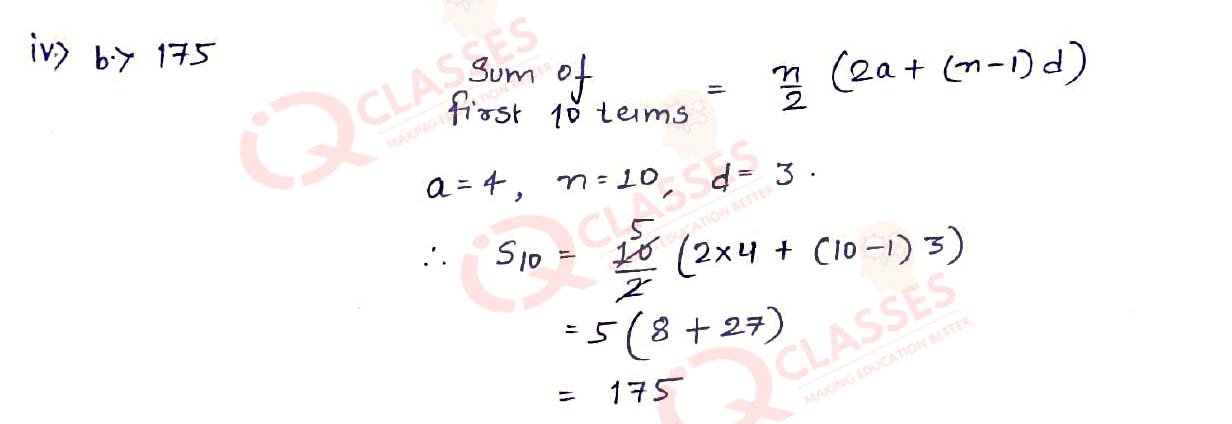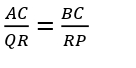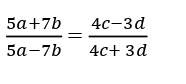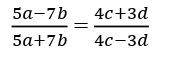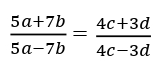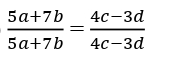Class 10 ICSE Term1 Maths Specimen 2022

SECTION-A

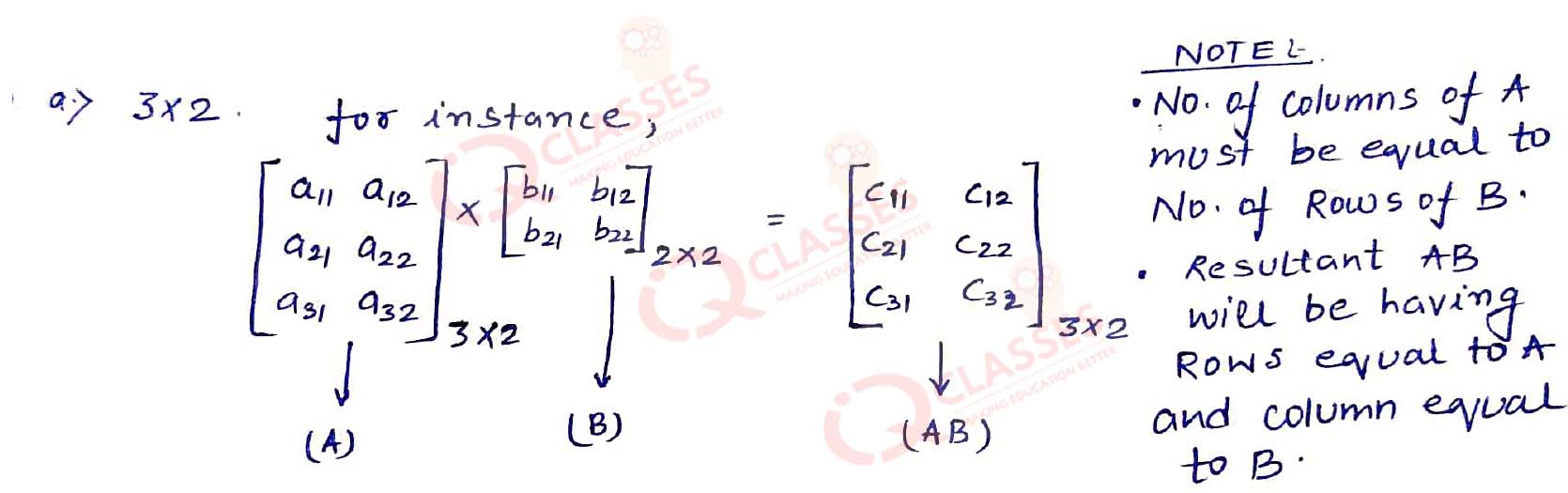
Q.1 If matrix A is of order 3 x 2 and matrix B is of order 2 x 2 then the matrix AB is of order

1. 3 x 2
2. 3 x 1
3. 2 x 3
4. 1 x 3

Solution

Q2 The percentage share of SGST of total GST for an Intra-State sale of an article is

1. 25%
2. 50%
3. 75%
4. 100%

Solution

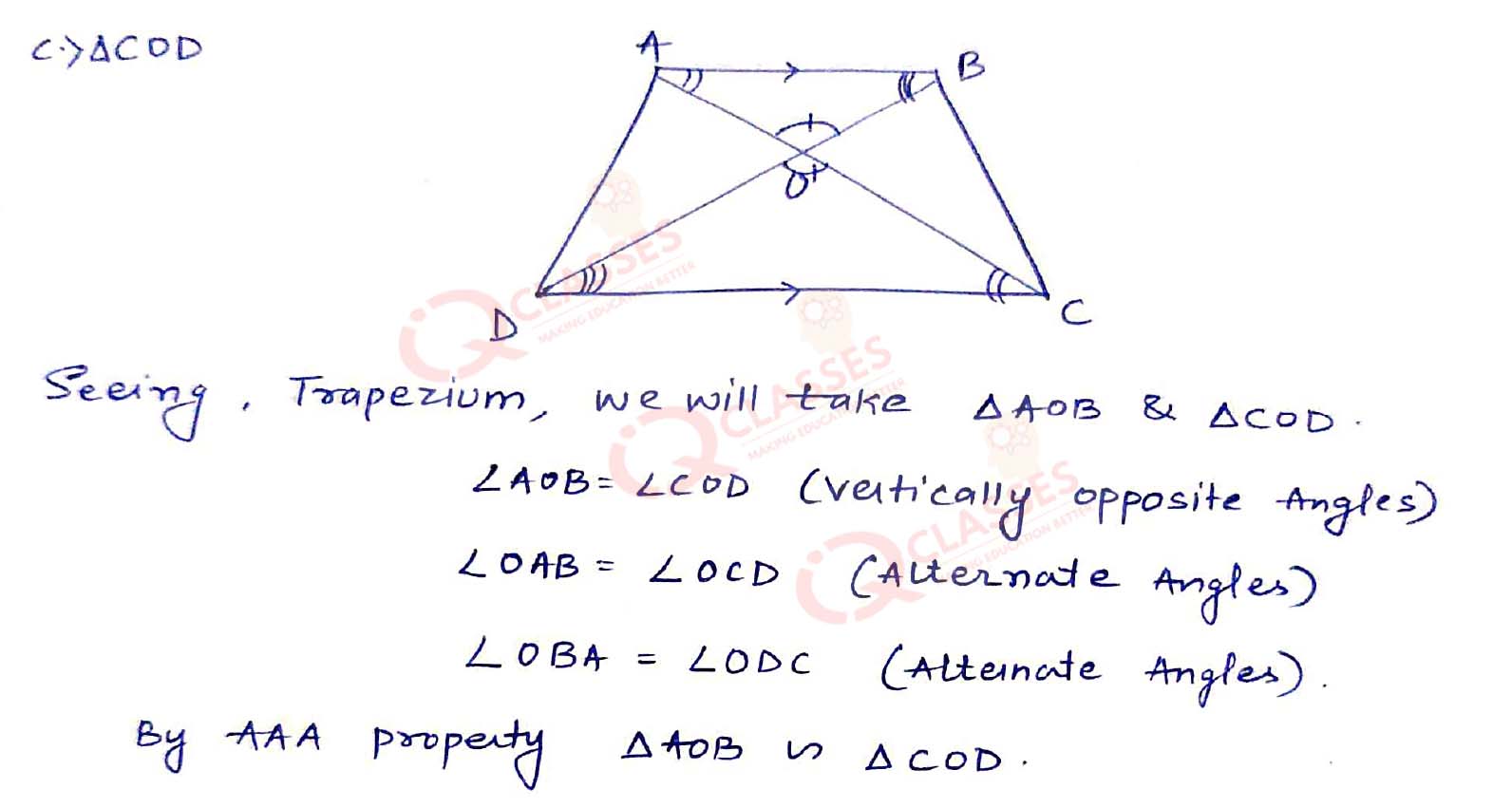
Q3 ABCD is a trapezium with AB parallel to DC. Then the triangle similar to triangle AOB is

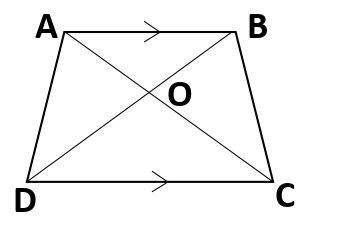
1. △ADB
2. △ACB
3. △COD
4. △COB

Solution

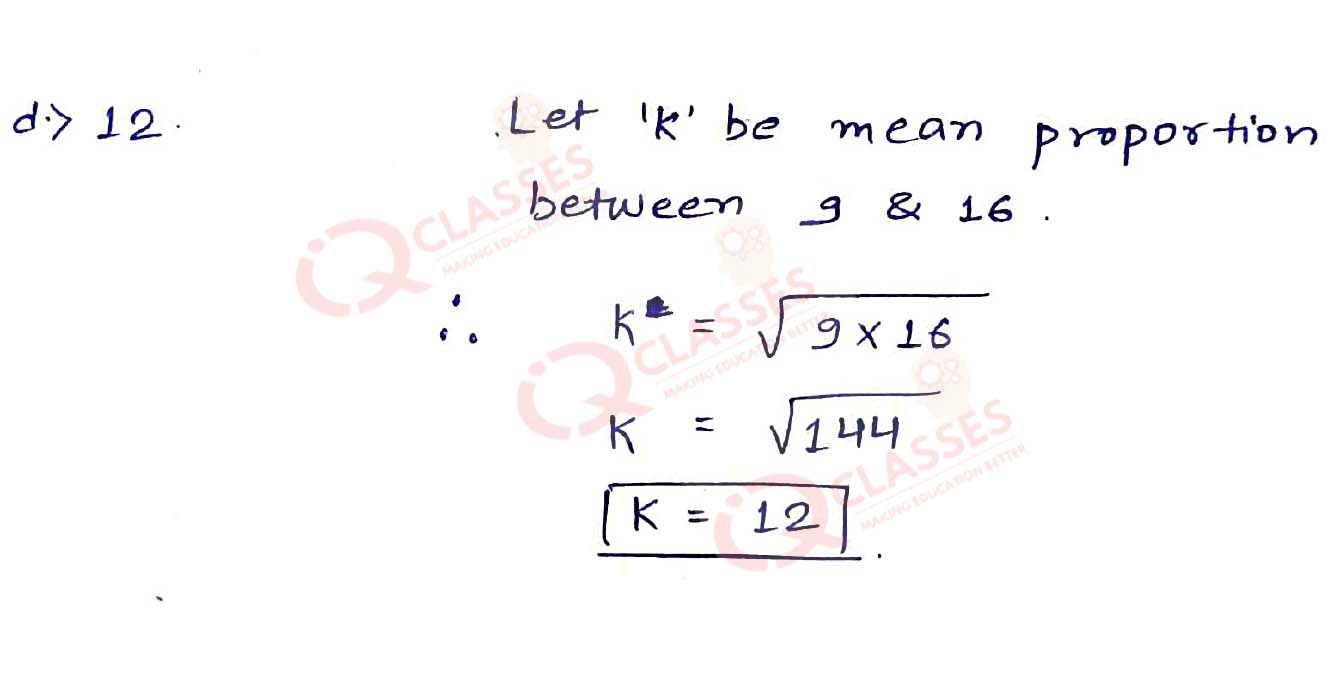
Q4 The mean proportion between 9 and 16 is

1. 25
2. 144
3. 7
4. 12

Solution

Q5 A man deposited Rs 500 per month for 6 months and received Rs 3300 as the maturity value. The interest received by him is:

1. 1950
2. 300
3. 2800
4. none of these

Solution

Q6 The solution set representing the following number line is

1. {x: x∈ R, -3 ≤ x < 2 }
2. {x: x∈ R, -3 < x < 2 }
3. {x: x∈ R, -3 < x ≤ 2 }
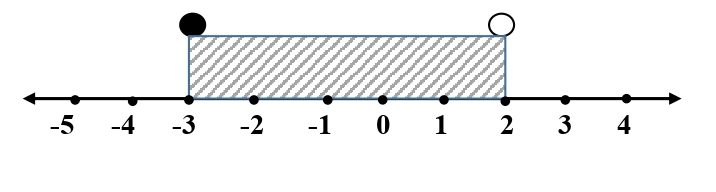
4. {x: x∈ R, -3 ≤ x ≤ 2 }

Solution

Q7 The first three terms of an arithmetic progression (A. P.) are 1, 9, 17, then the next two terms are

1. 25 and 35
2. 27 and 37
3. 25 and 33
4. none of these

Solution

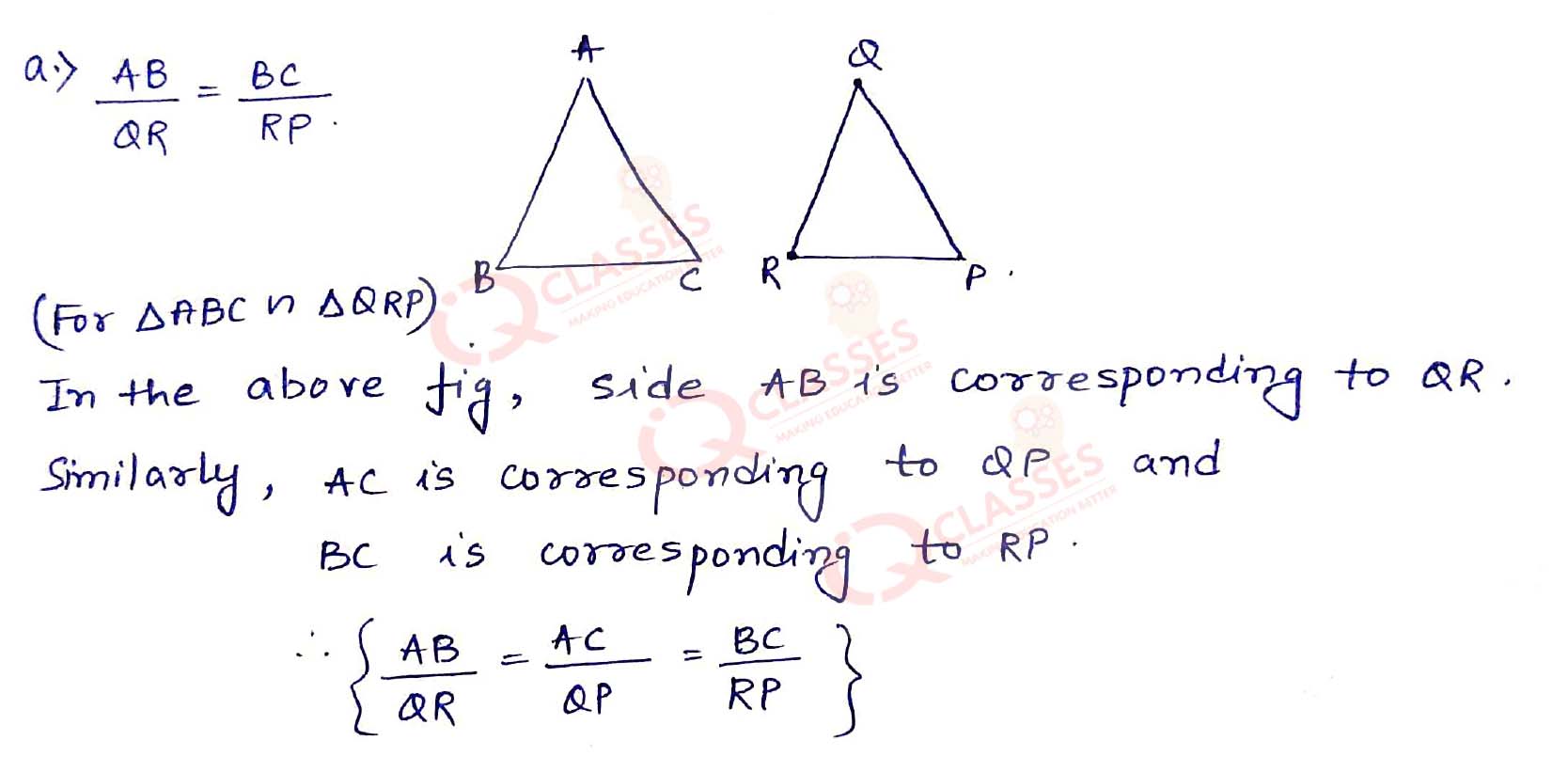
Q8 If △ABC∽△QRP then the corresponding proportional sides are

Solution

Q9 If x ∈W, then the solution set of the inequation -x > -7, is

1. {8,9,10.....}
2. {0,1,2,3,4,5,6}
3. {0,1,2,3.....}
4. {-8,-9,-10...}

Solution

Q10 The roots of the quadratic equation 4x² - 7x + 2 = 0 are 1.390, 0. 359.The roots correct to 2 significant figures are

1. 1.39 and 0.36
2. 1.3 and 0.35
3. 1.4 and 0.36
4. 1.390 and 0.360

Solution

Q11 1.5, 3, x and 8 are in proportion, then x is equal to

1. 6
2. 4
3. 4.5
4. 16

Solution

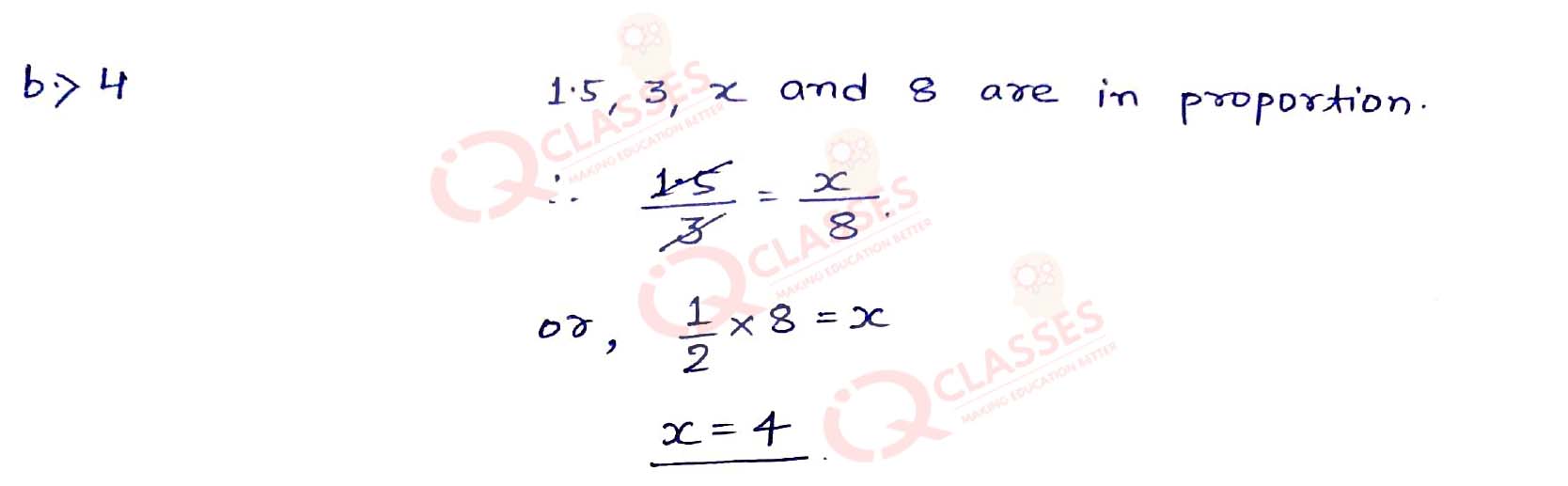
Q12 If a polynomial 2x² – 7x – 1 is divided by (x + 3), then the remainder is

1. -4
2. 38
3. -3
4. 2

Solution

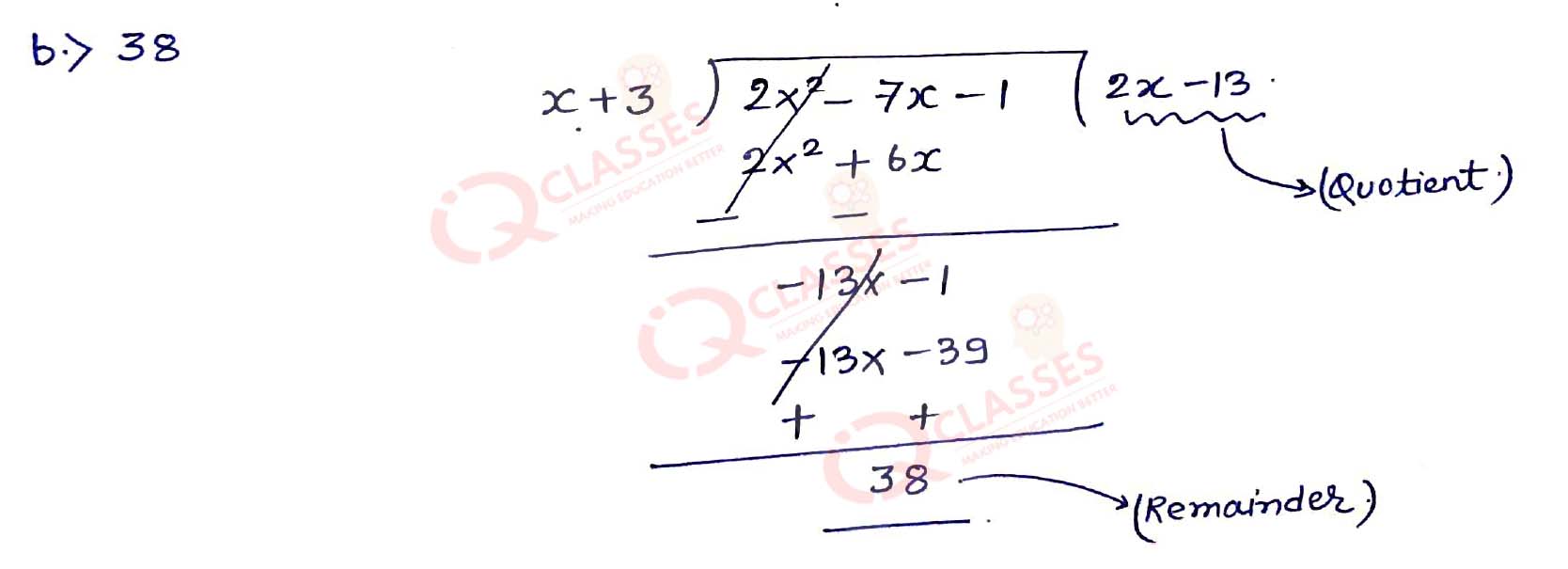
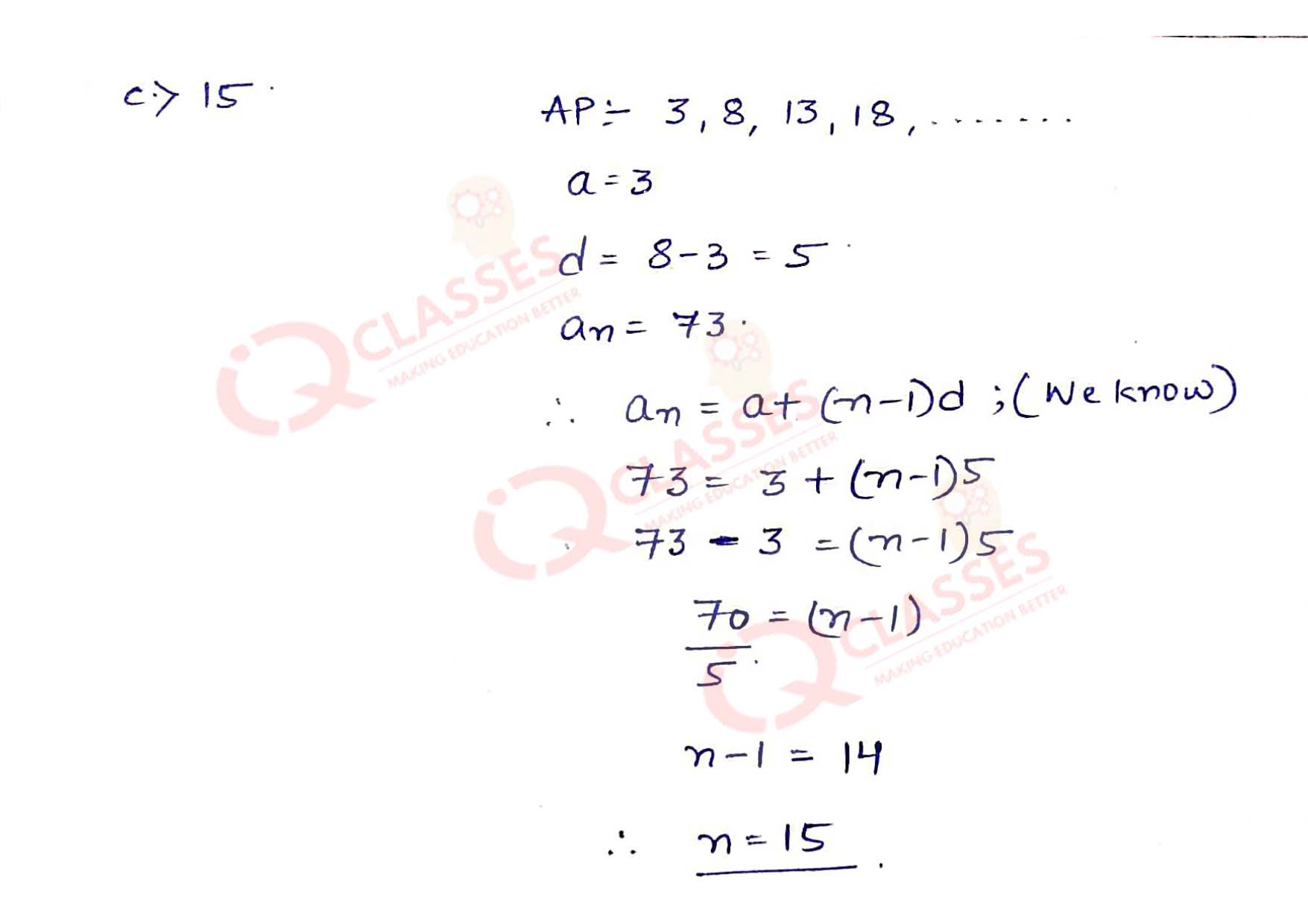
Q13 If 73 is the nth term of the arithmetic progression 3, 8, 13, 18..., then `n' is

1. 13
2. 14
3. 15
4. 16

Solution

Q14 The roots of the quadratic equation x² + 2x + 1 = 0 are

1. Real and distinct
2. Real and equal
3. Distinct
4. Not real/ imaginary

Solution

Q15 Which of the following statement is not true?

1. All identity matrices are square matrix
2. All null matrices are square matrix
3. For a square matrix number of rows is equal to the number of columns
4. A square matrix all of whose elements except those in the leading diagonal are zero is the diagonal matrix

Solution

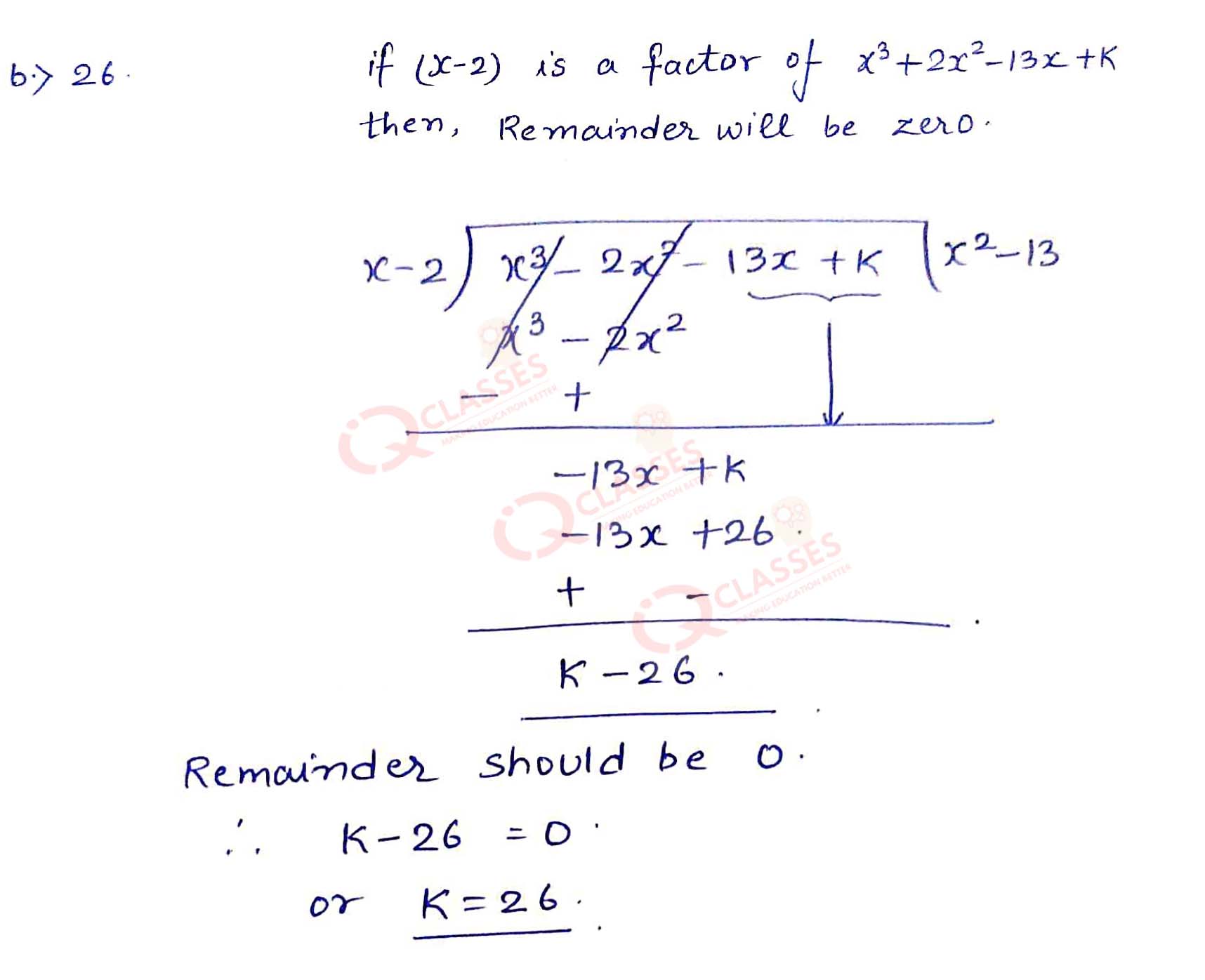
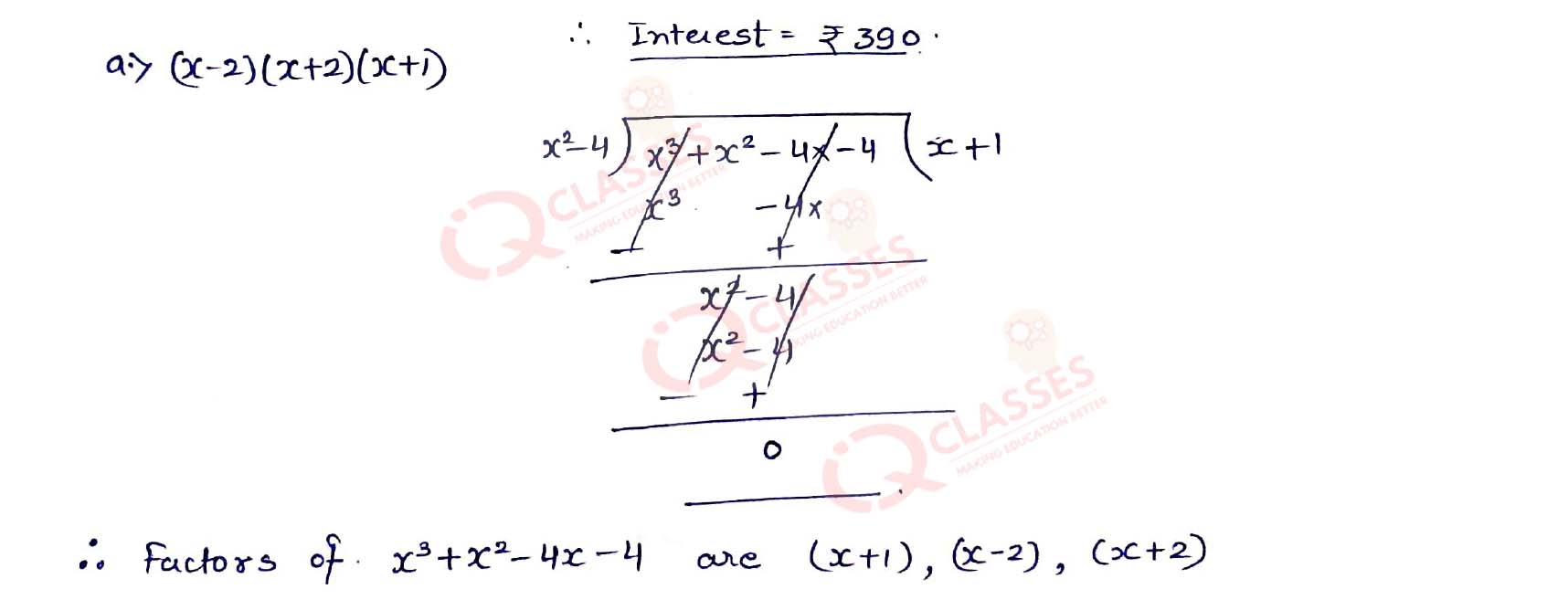
Q16 If (x — 2) is a factor of the polynomial x³ + 2x² — 13 x + k, then 'k' is equal to

1. -10
2. 26
3. -26
4. 10

Solution

SECTION-B

Q17 A man deposited 21200 in a recurring deposit account for 1 year at 5% per annum simple interest. The interest earned by him on maturity is

1. 14790
2. 390
3. 4680
4. 780

Solution

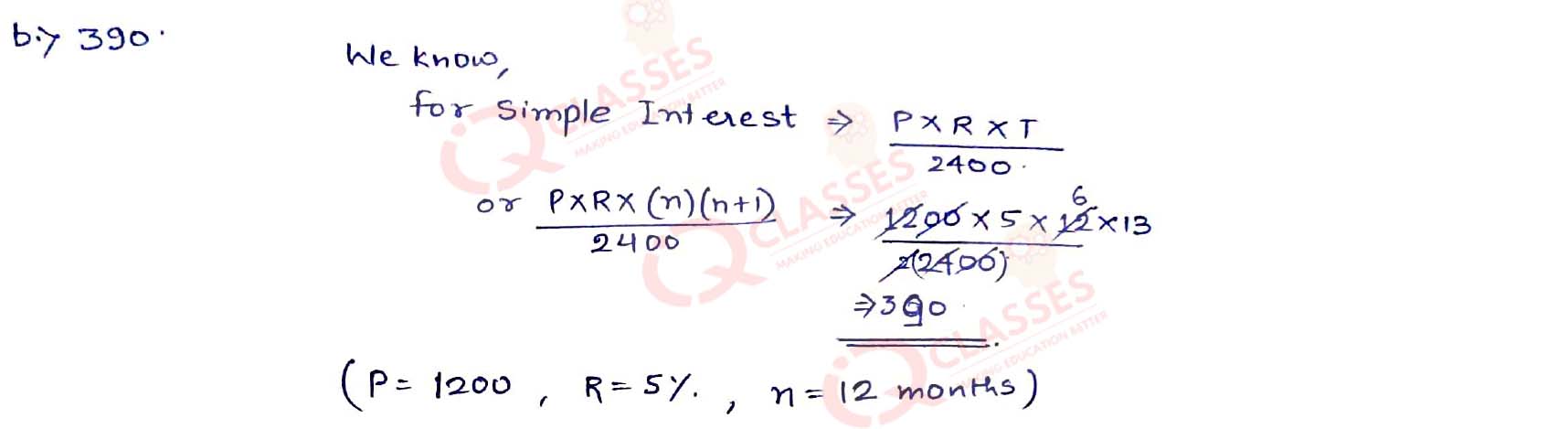
Q18 If x² — 4 is a factor of polynomial x³+ x² - 4x- 4, then its factors are

1. (x-2) (x+2) (x+1)
2. (x-2) (x+2) (x-1)
3. (x-2) (x-2) (x+1)
4. (x-2) (x-2) (x-1)

Solution

Q19 The following bill shows the GST rates and the marked price of articles A and B:

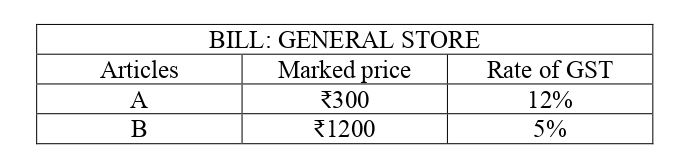
1. 1548
2. 1596
3. 1560
4. 1536

Solution

Q20 The solution set for the linear inequation -8 ≤ x — 7 < - 4, x ∈ I is

1. {x : x ∈ R, -1≤ x < 3
2. {0,1,2,3}
3. {-1,0,1,2,3}
4. {-1,0,1,2}

Solution

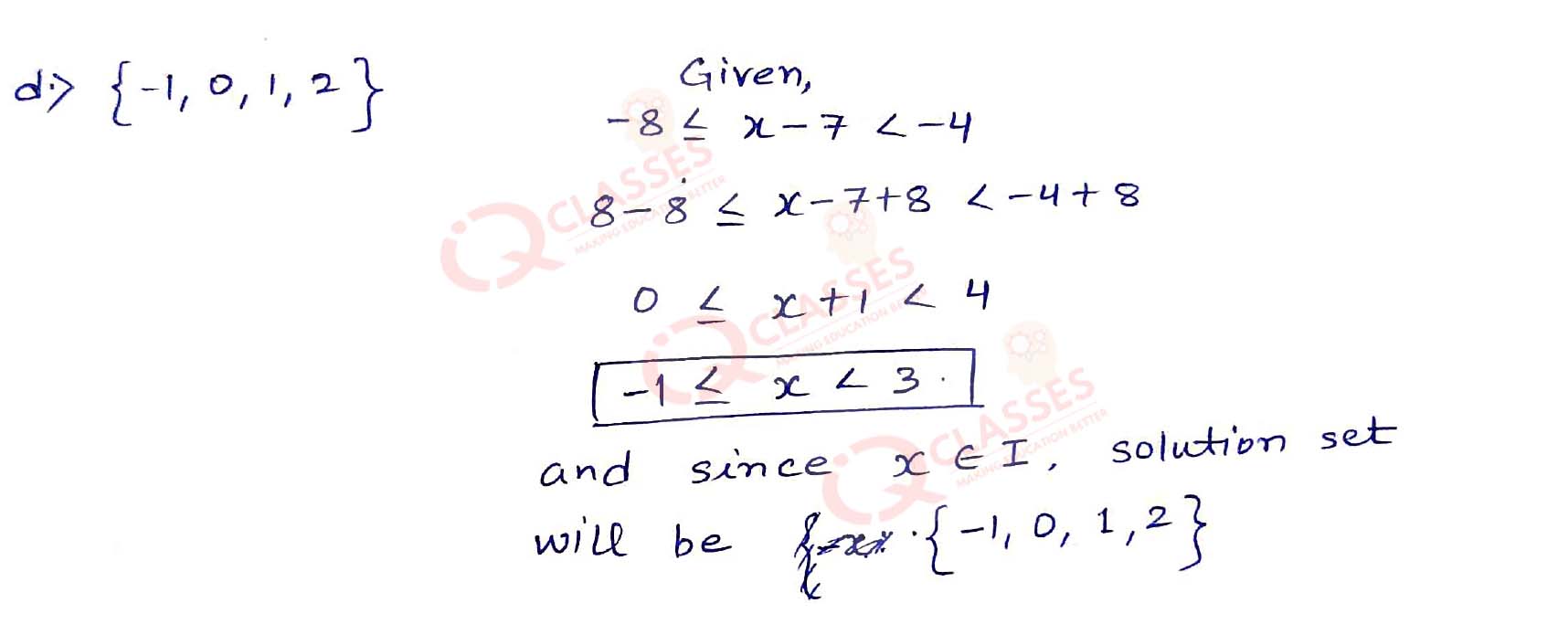
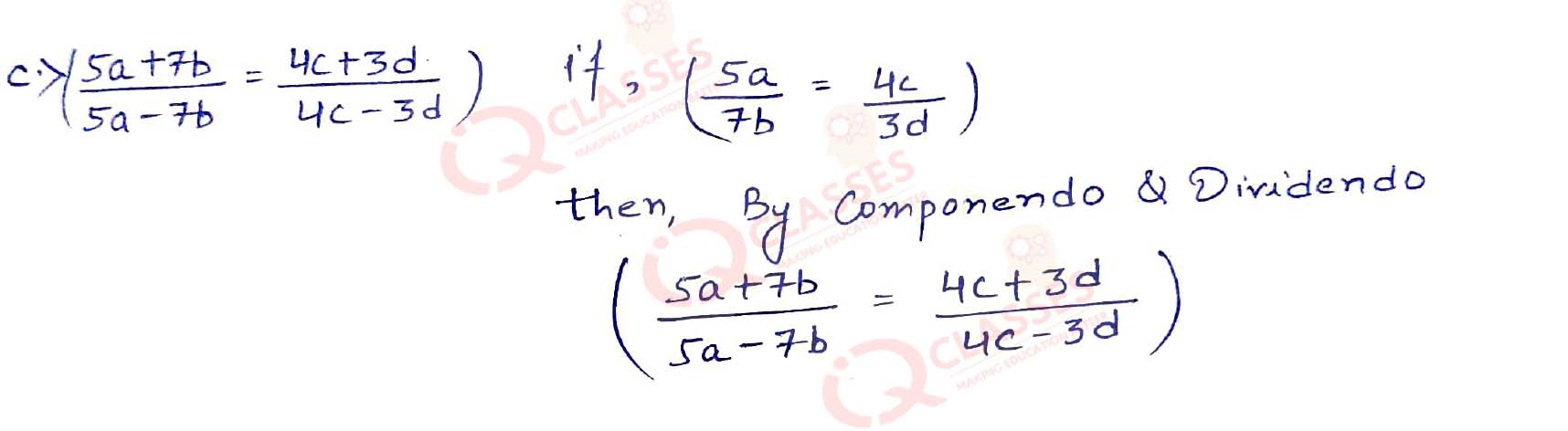
Q21 If 5a/7b = 4c/3d, then by componendo and dividendo

Solution

Q22 If A =

2	4
-1	7

then A² is

1. 4	0
   1	49

2. 4	0
   -9	49

3. 4	0
   9	49

4. 1	9
   -9	48

Solution

SECTION-C

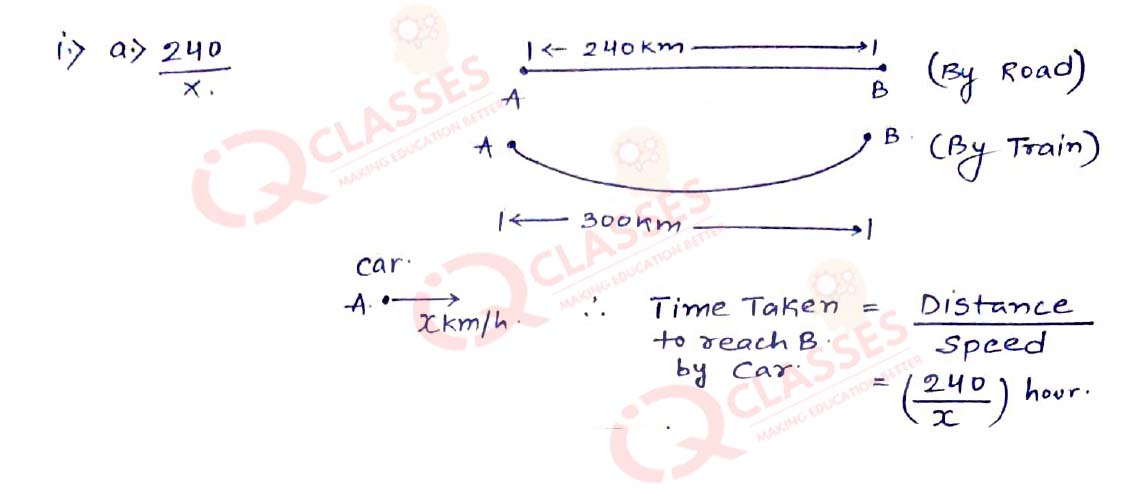
Q23 The distance between station A and B by road is 240 km and by train it is 300 km. A car starts from station A with a speed x km/hr whereas a train starts from station B with a speed 20km/hr more than the speed of the car.
(i) The time taken by car to reach station B is

1. 240 / x
2. 300 /x
3. 20 / x
4. 300 / (x+20)

Solution

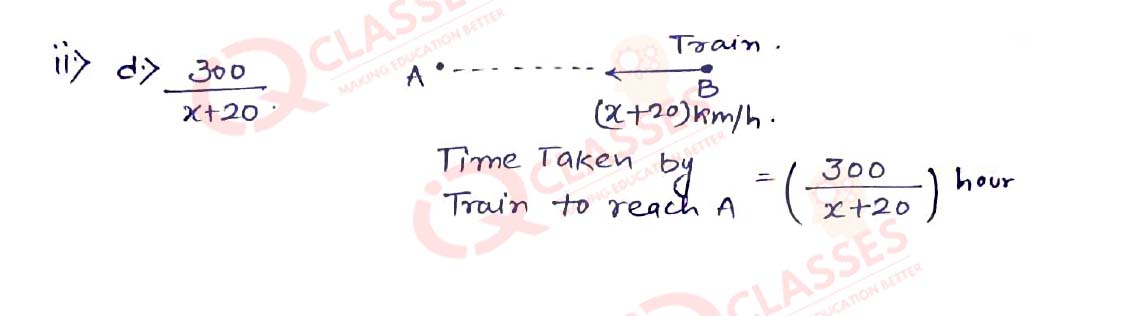
(ii) The time taken by train to reach station A

1. 240 / x
2. 300 /x
3. 20 / x
4. 300 / (x+20)

Solution

(iii)) If the time taken by train is 1 hour less than that taken by the car, then the quadratic equation formed is

1. x² + 80x – 6000=0
2. x² + 80x –4800=0
3. x²+ 240x - 1600 =0
4. x²-80x +4800 = 0

Solution

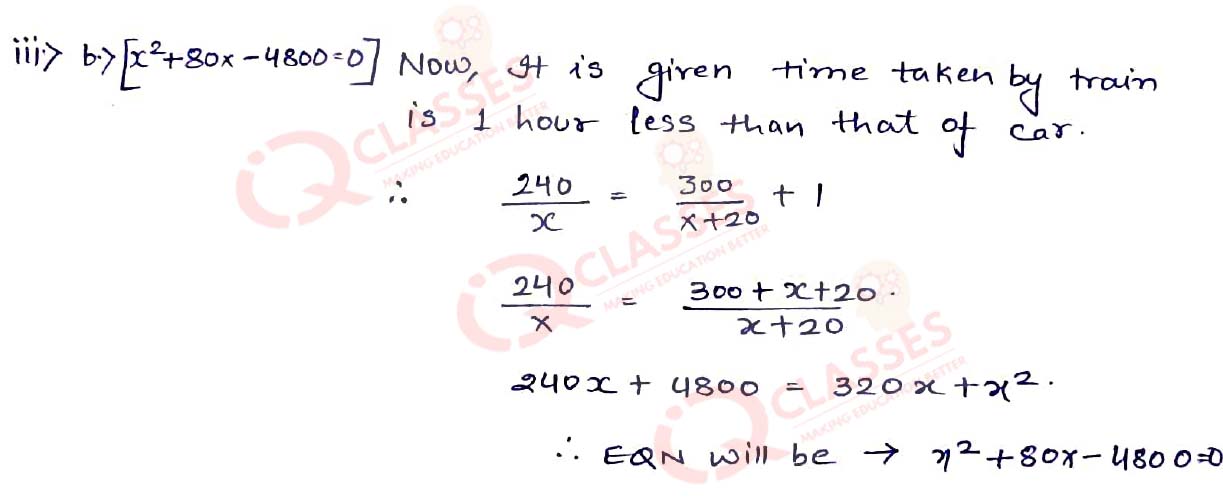
(iv) The speed of the car is

1. 60km/hr
2. 120km/hr
3. 40km/hr
4. 80km/hr

Solution

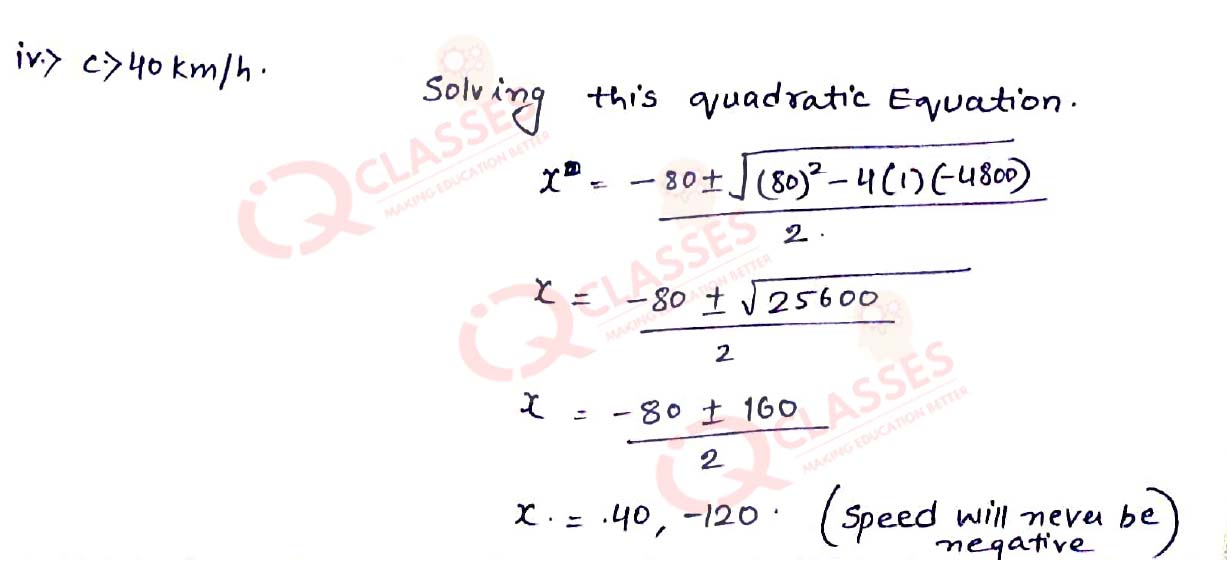
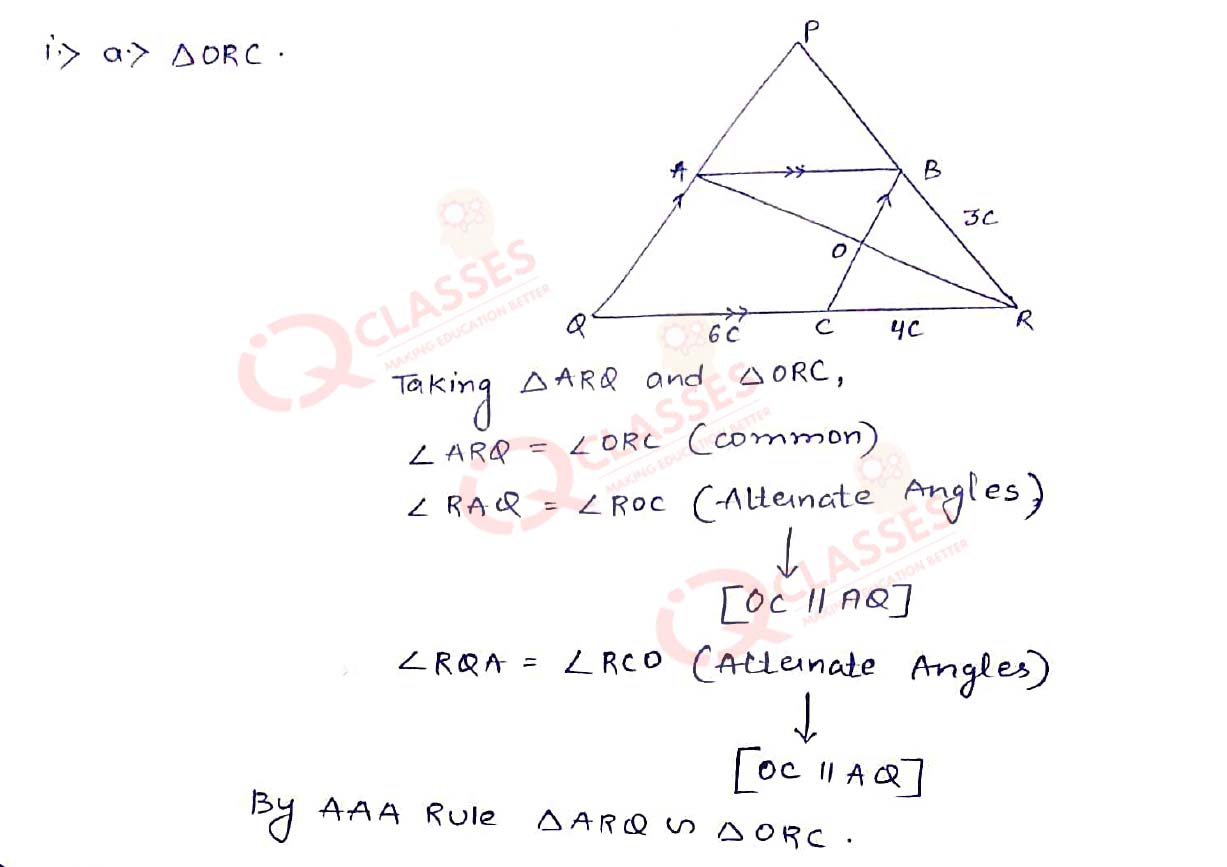
Q24 In the given triangle PQR, AB || QR, QP || CB and AR intersects CB at 0. P 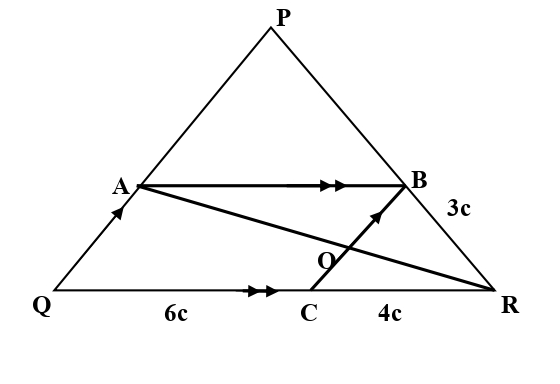Using the given diagram answer the following question:
(i) The triangle similar to △ARQ is

1. △ORC
2. △ARP
3. △OBR
4. △QRP

Solution

(ii) △PQR ∽ △BCR by axiom

1. SAS
2. AAA
3. SSS
4. AAS

Solution

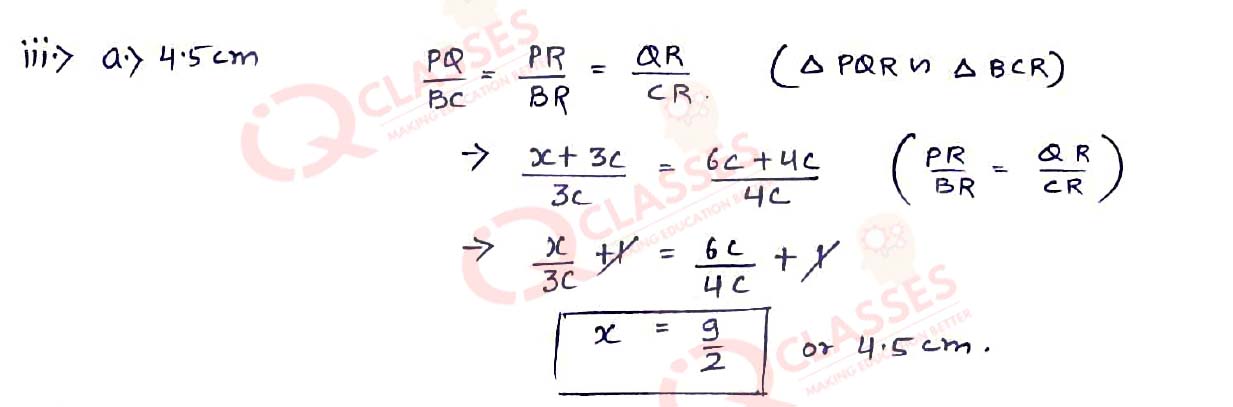
(iii) If QC =6 cm, CR = 4 cm, BR = 3 cm. The length of RP is

1. 4.5 cm
2. 8cm
3. 7.5cm
4. 5cm

Solution

(iv) The ratio PQ: BC is 25

1. 2:3
2. 3:2
3. 5:2
4. 2:5

Solution

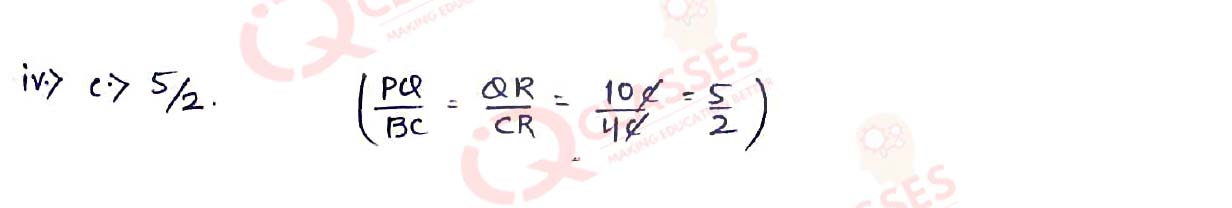
Q25 The nth term of an arithmetic progression (A.P.) is (3n + 1)
(i)The first three terms of this A. P. are

1. 5, 6, 7
2. 3, 6, 9
3. 1, 4, 7
4. 4, 7, 10

Solution

(ii) The common difference of the A.P. is

1. 3
2. 1
3. -3
4. 2

Solution

(iii) Which of the following is not a term of this A.P

1. 25
2. 27
3. 28
4. 31

Solution

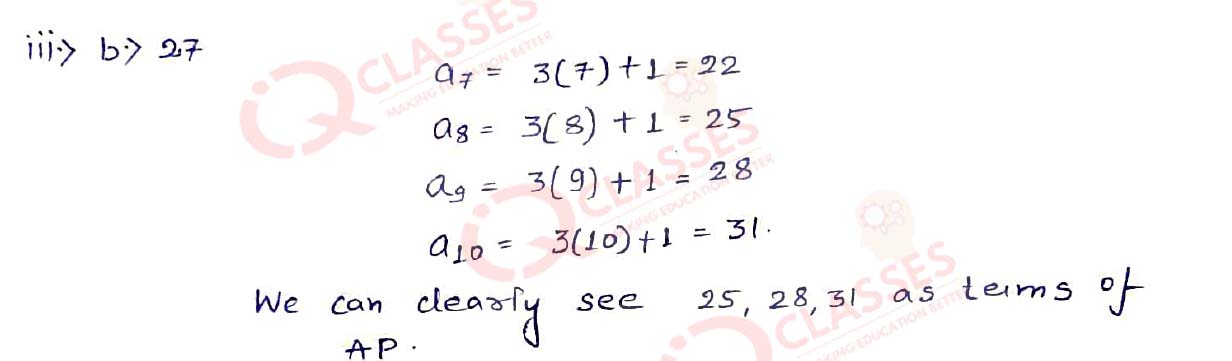
(iv) Sum of the first 10 terms of this A.P. is

1. 350
2. 175
3. -95
4. 2 : 5

Solution