Q1
State the condition when on applying a force, a body has:
(a) translational motion
(b) rotational motion.
solutions
(a) translational motion
(b) rotational motion.
solutions

Q2
Define moment of force and state its S.I. unit.
solutions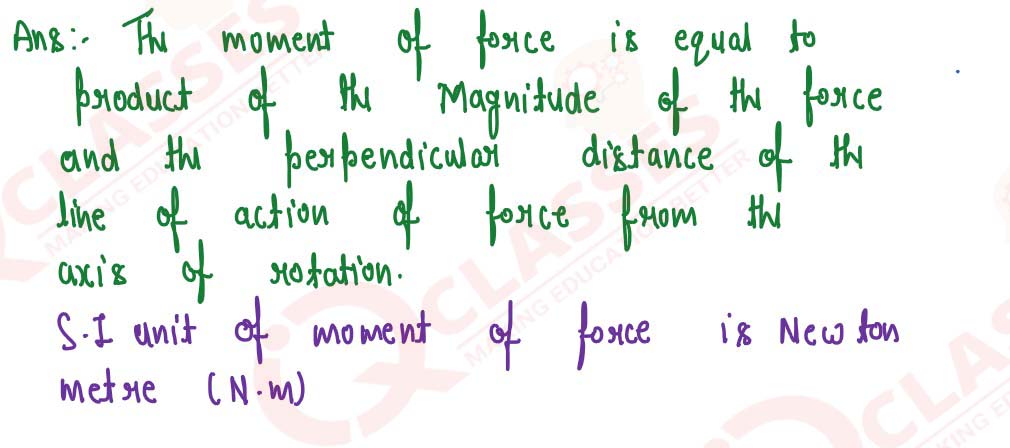
solutions

Q3
State whether the moment of force is a scalar or vector quantity?
solutions
solutions

Q4
State two factors affecting the turning effect of a force.
solutions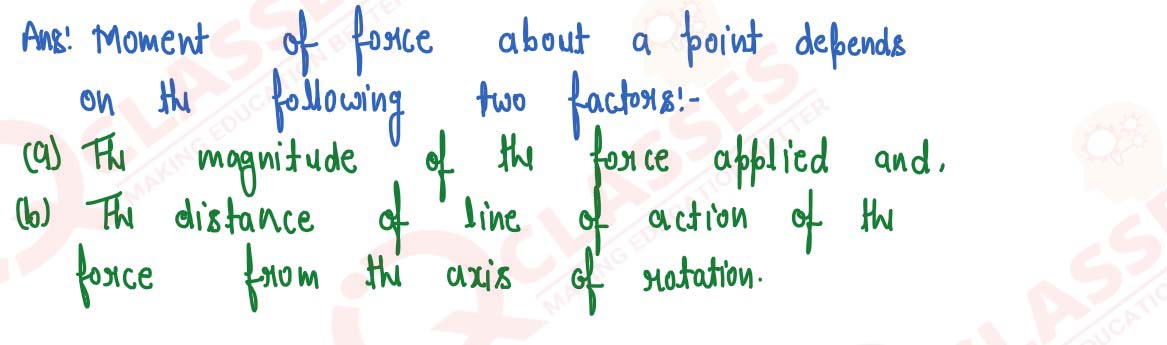
solutions

Q5
When does a body rotate? State one way to change the direction of rotation of a body. Give a suitable example to explain your answer.
solutions

solutions


Q6
Write the expression for the moment of force about a given axis
solutions
solutions

Q7
What do you understand by the clockwise and anticlockwise moment of force? When is it taken positive?
solutions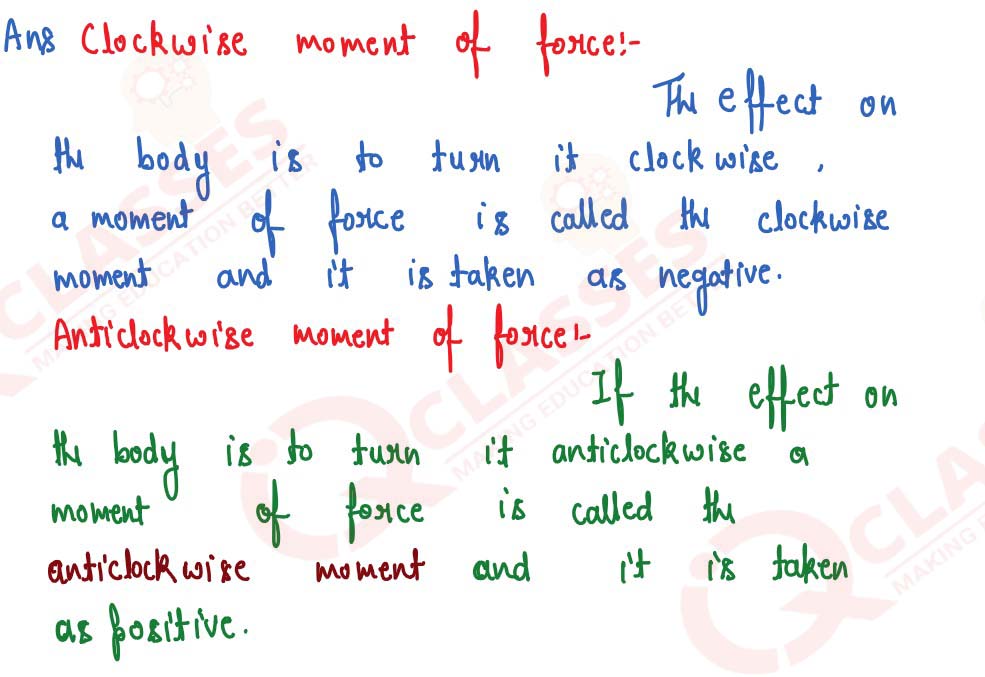
solutions

Q8
State one way to reduce the moment of a given force about a given axis of rotation
solutions
solutions

Q9
State one way to obtain a greater moment of a force about a given axis of rotation.
solutions
solutions

Q10
Why is it easier to open a door by applying the force at the free end of it?
solutions
solutions

Q11
The stone of hand flour grinder is provided with a handle near its rim. Give a reason
solutions
solutions

Q12
It is easier to turn the steering wheel of a large diameter than that of a small diameter. Give reason.
solutions
solutions

Q13
A spanner (or wrench) has a long handle. Why?
solutions
solutions

Q14
A jack screw is provided with a long arm. Explain why?
solutions
solutions

Q15
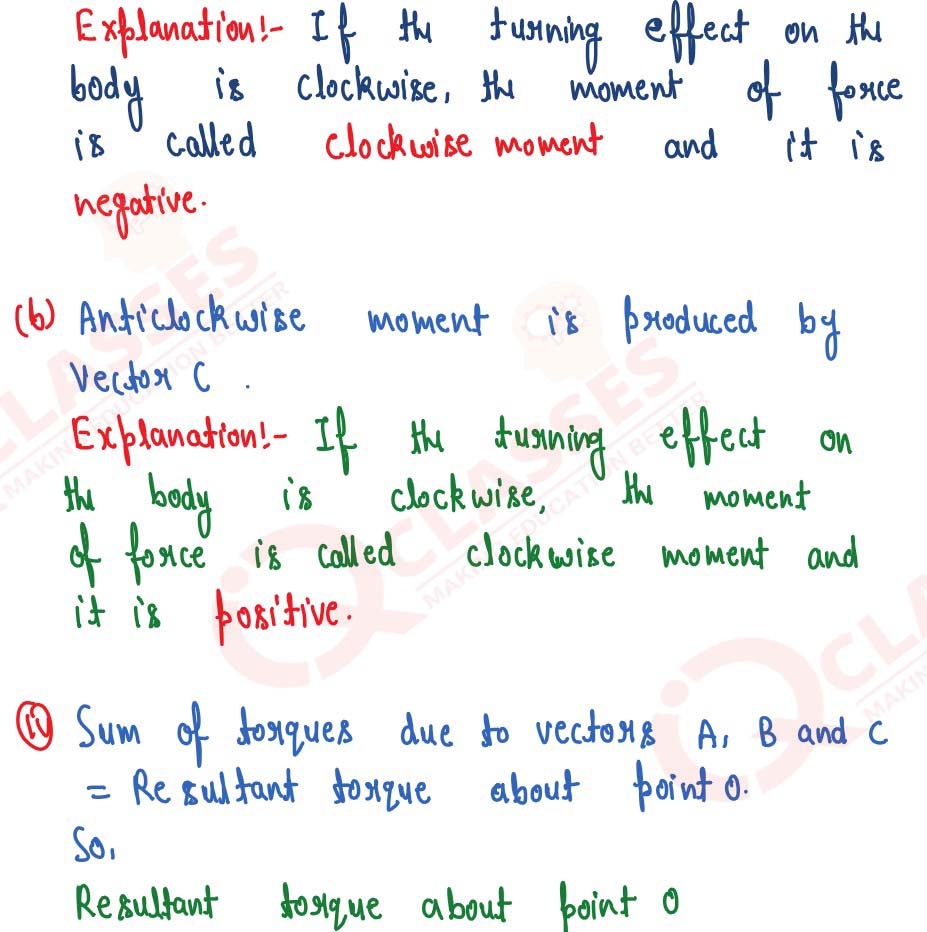
A, B and C are the three forces each of magnitude 4N acting in the plane of paper as shown in figure. The point O lies in the same plane.
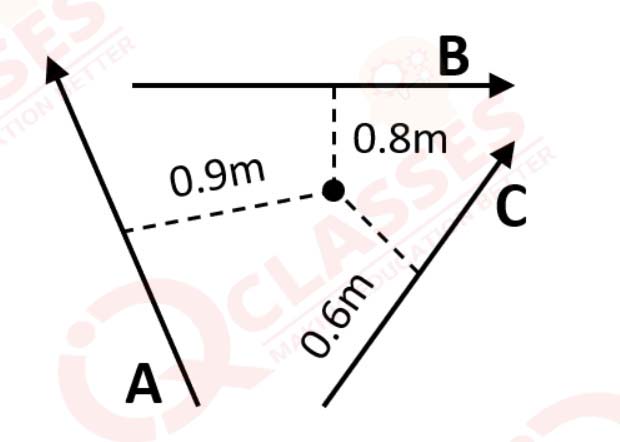
(i) Which force has the least moment about O? Give reason.
(ii) Which force has the greatest moment about O? Give reason.
(iii) Name the forces producing (a) clockwise, (b) anticlockwise moments.
(iv) What is the resultant torque about the point O?
solutions


(i) Which force has the least moment about O? Give reason.
(ii) Which force has the greatest moment about O? Give reason.
(iii) Name the forces producing (a) clockwise, (b) anticlockwise moments.
(iv) What is the resultant torque about the point O?
solutions



Q16
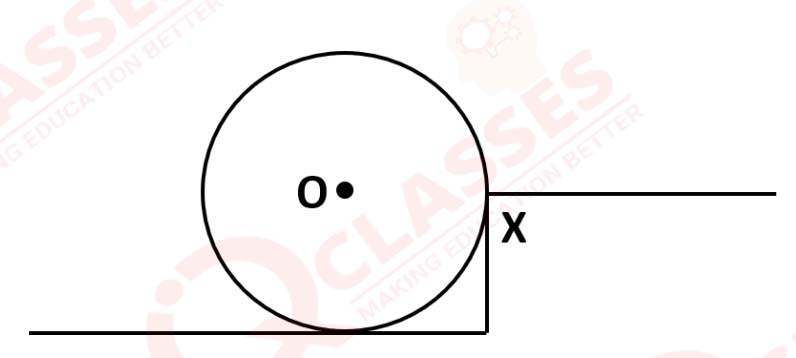
The adjacent diagram shows a heavy roller, with its axle at O, which is to be raised on a pavement XY. If there is friction between the roller and pavement, show by an arrow on the diagram the point of application and the direction of force to be applied. If pivoted at 0, now will it go up?
solutions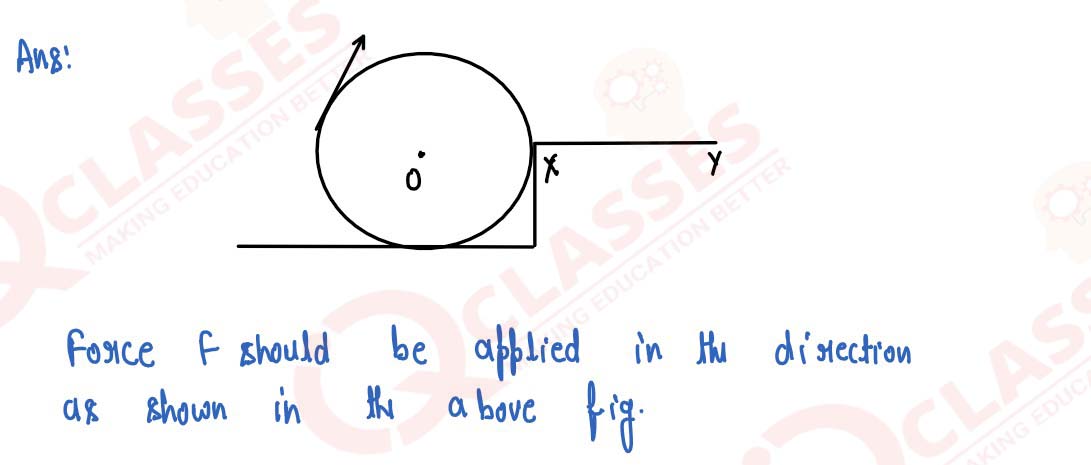

solutions

Q17
A body is acted upon by two forces each of magnitude F, but in opposite directions. State the effect of the forces if
(a) both forces act at the same point of the body.
(b) the two forces act at two different points of the body at a separation r.
solutions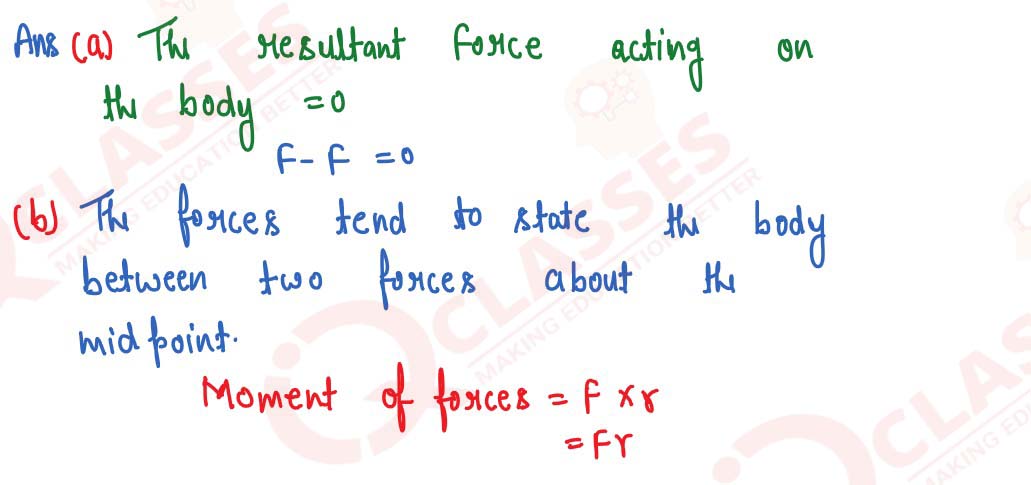
(a) both forces act at the same point of the body.
(b) the two forces act at two different points of the body at a separation r.
solutions

Q18
Draw a neat labelled diagram to show the direction of two forces acting on a body to produce rotation in it. Also mark the point O about which the rotation takes place.
solutions
solutions

Q19
What do you understand by the term couple? State its effect on a body. Give two examples in our daily life where couple is applied to turn a body.
solutions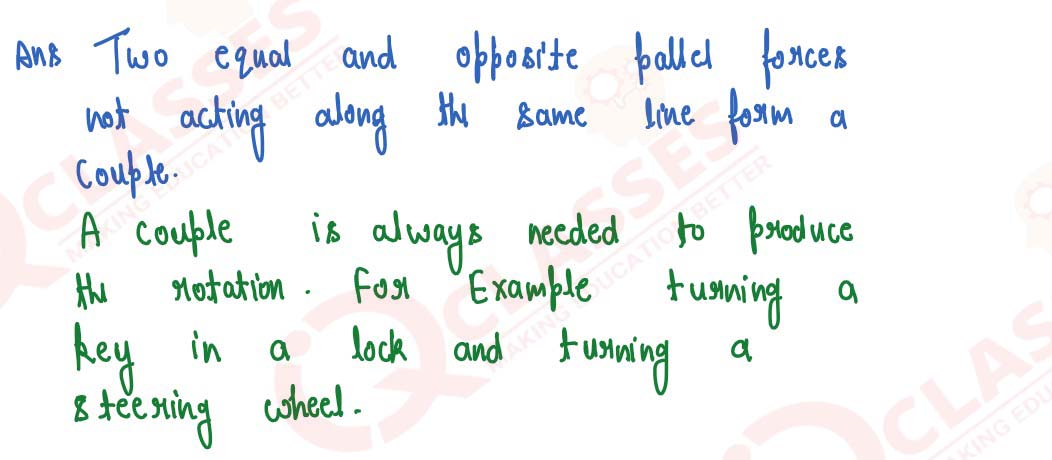
solutions

Q20
Define the moment of a couple. Write its S.I unit.
solutions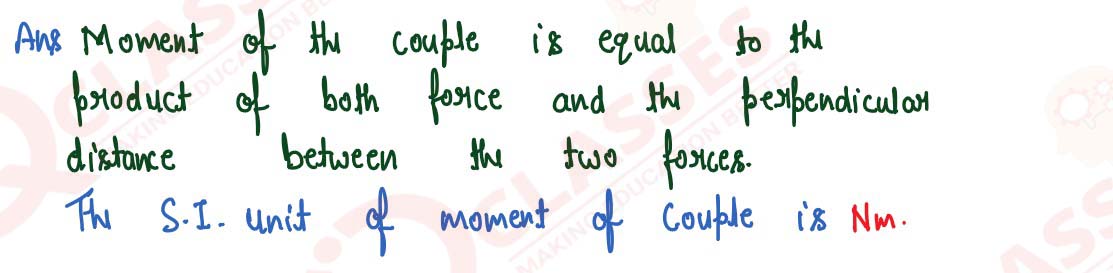
solutions

Q21
Prove that
Moment of couple = Force x Couple arm.
solutions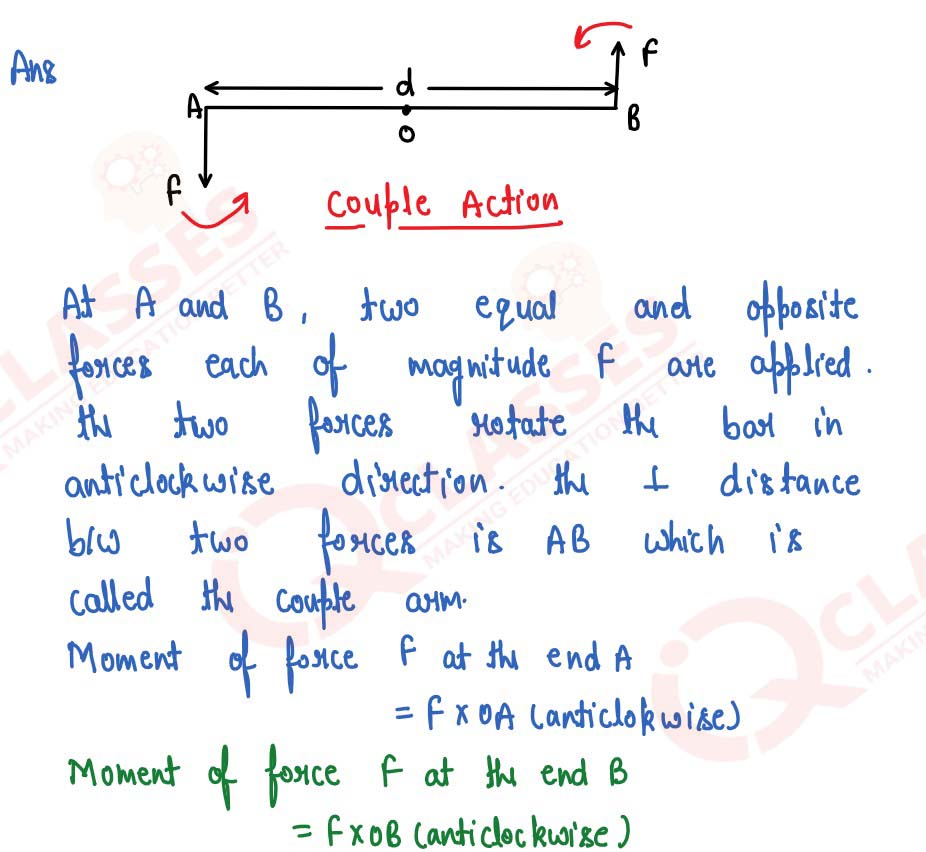
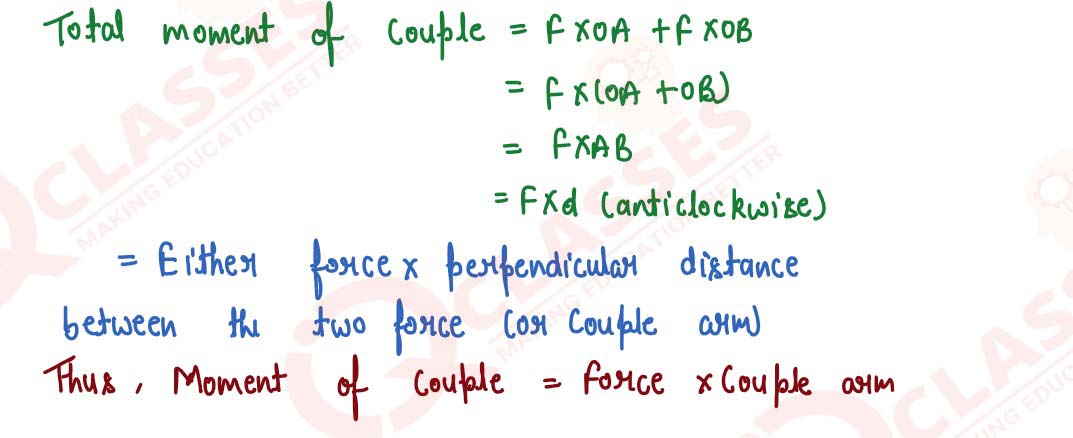
Moment of couple = Force x Couple arm.
solutions


Q22
What do you mean by equilibrium of a body?
solutions
solutions

Q23
State the condition when a body is in
(i) static equilibrium.
(ii) dynamic equilibrium.
Give one example each of static and dynamic equilibrium.
solutions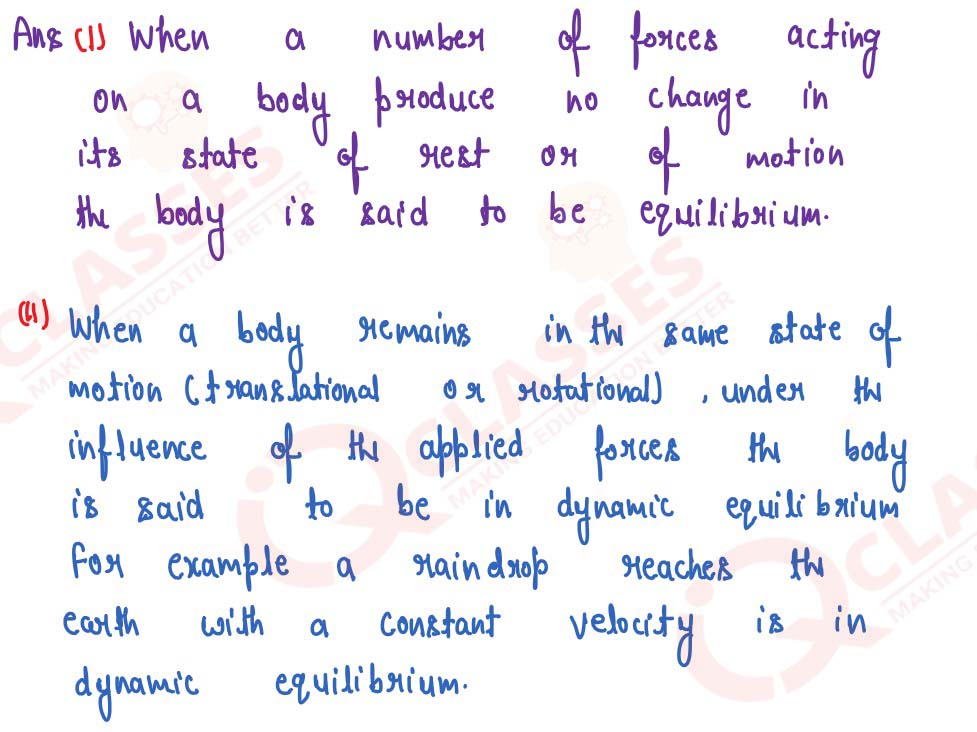
(i) static equilibrium.
(ii) dynamic equilibrium.
Give one example each of static and dynamic equilibrium.
solutions

Q24
State two conditions for a body, acted upon by several forces to be in equilibrium.
solutions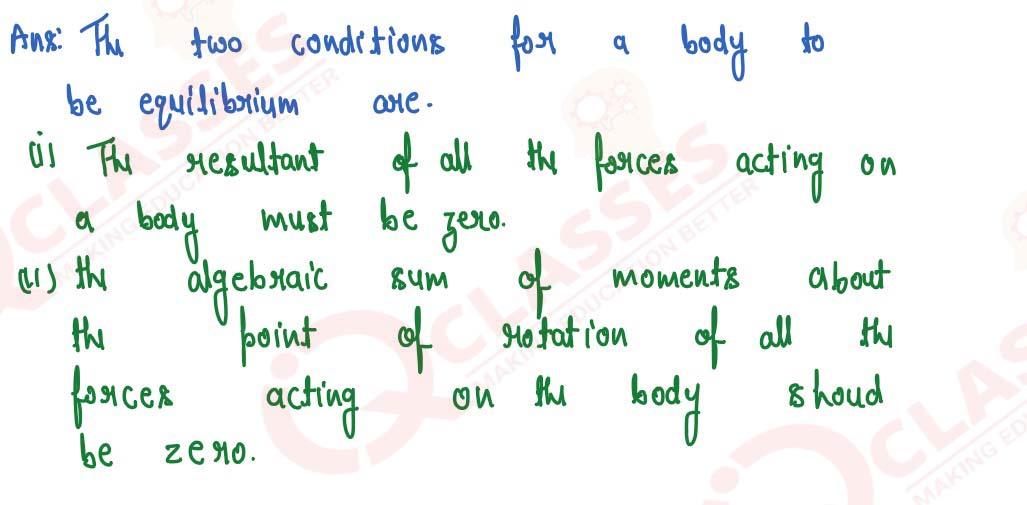
solutions

Q25
State the principle of moments. Name one device based on it.
solutions
solutions

Q26
Describe a simple experiment to verify the principle of moments, if you are supplied with a metre rule, a fulcrum and two springs with slotted weights.
solutions
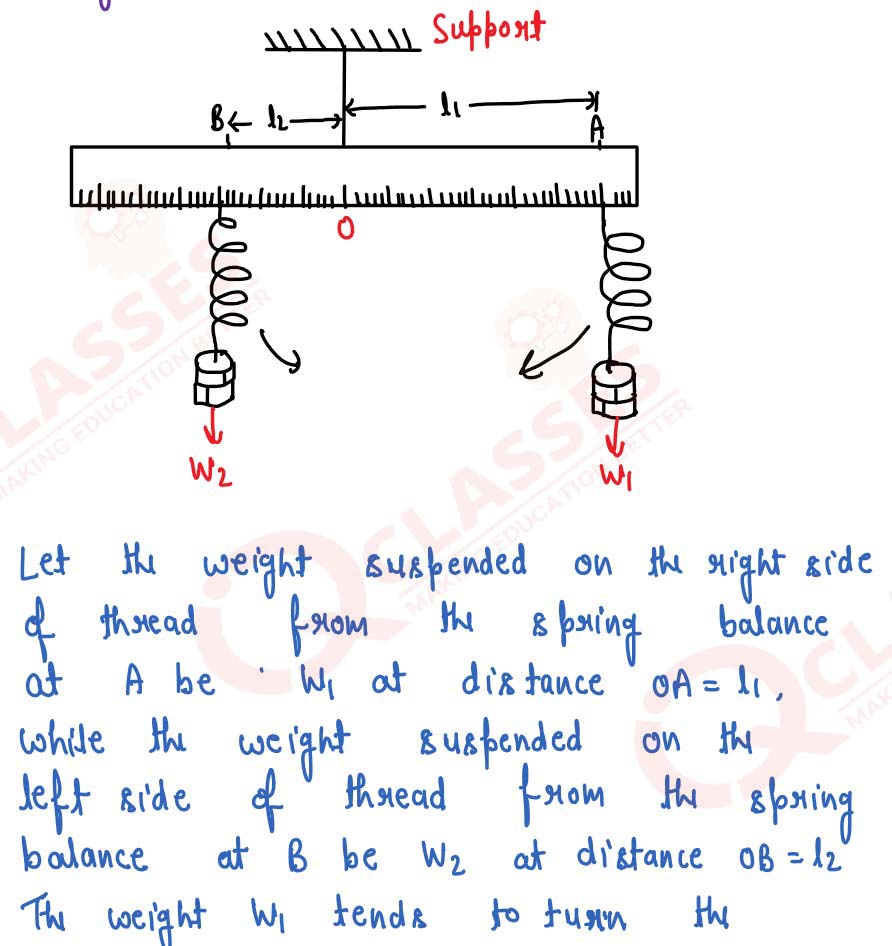

solutions



Q27
Complete the following sentences:
(i) The S.I. unit of moment of force is ________
(ii) In equilibrium algebraic sum of moments of all forces about the point of rotation is _________.
(iii) In beam balance when the beam is balanced in a horizontal position, it is in ________ equilibrium.
(iv) The moon revolving around the earth is in _________ equilibrium.
solutions
(i) The S.I. unit of moment of force is ________
(ii) In equilibrium algebraic sum of moments of all forces about the point of rotation is _________.
(iii) In beam balance when the beam is balanced in a horizontal position, it is in ________ equilibrium.
(iv) The moon revolving around the earth is in _________ equilibrium.
solutions

Multiple Choice type Questions :
Q1
The moment of a force about a given axis depends:
1. only on the magnitude of force
2. only on the perpendicular distance of force from the axis
3. neither on the force nor on the perpendicular distance of force from the axis
4. both, on the force and its perpendicular distance from the axis.
solutions
1. only on the magnitude of force
2. only on the perpendicular distance of force from the axis
3. neither on the force nor on the perpendicular distance of force from the axis
4. both, on the force and its perpendicular distance from the axis.
solutions

Q2
A body is acted upon by two unequal forces in opposite directions, but not in the same line. The effect is that:
1. the body will have only the rotational motion
2. the body will have only the translational motion
3. the body will have neither the rotational motion nor the translational motion
4. the body will have rotational as well as translational motion.
solutions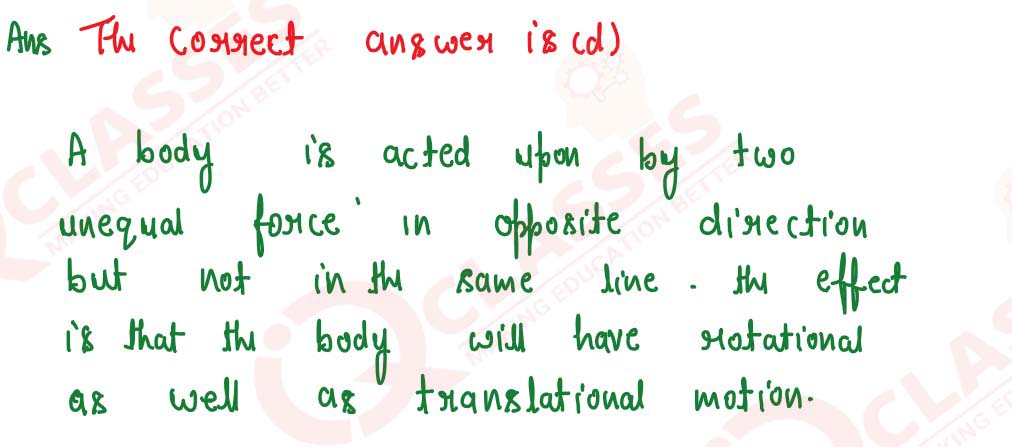
1. the body will have only the rotational motion
2. the body will have only the translational motion
3. the body will have neither the rotational motion nor the translational motion
4. the body will have rotational as well as translational motion.
solutions

Numericals
Q1
The moment of a force of 10N about a fixed point O is 5Nm. Calculate the distance of the point O from the line of action of the force
solutions
solutions

Q2
A nut is opened by a wrench of length 10cm. If the least force required is 5.0N, find the moment of force needed to turn the nut.
solutions
solutions

Q3
A wheel of diameter 2m is shown in figure with axle at O. A force F = 2N is applied at B in the direction shown in figure
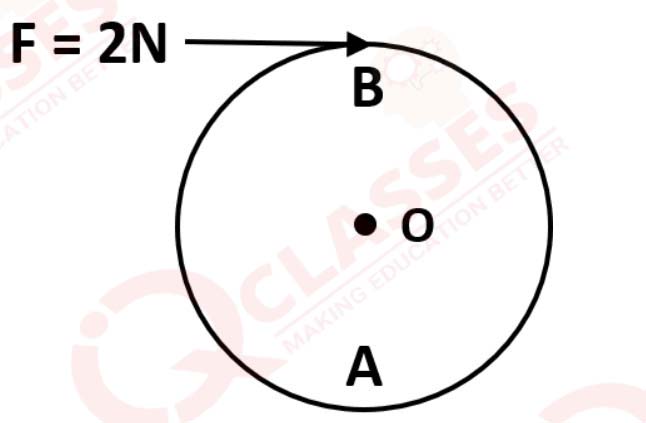
Calculate the moment of force about
(i) the centre O, and
(ii) the point A.
solutions

Calculate the moment of force about
(i) the centre O, and
(ii) the point A.
solutions

Q4
The diagram shows two forces F1 = 5N and F2 = 3N acting at points A and B of a rod pivoted at a point O, such that OA = 2m and OB = 4m

Calculate:
(i) the moment of force F1 about O.
(ii) the moment of force F2 about O.
(iii) total moment of the two forces about O.
solutions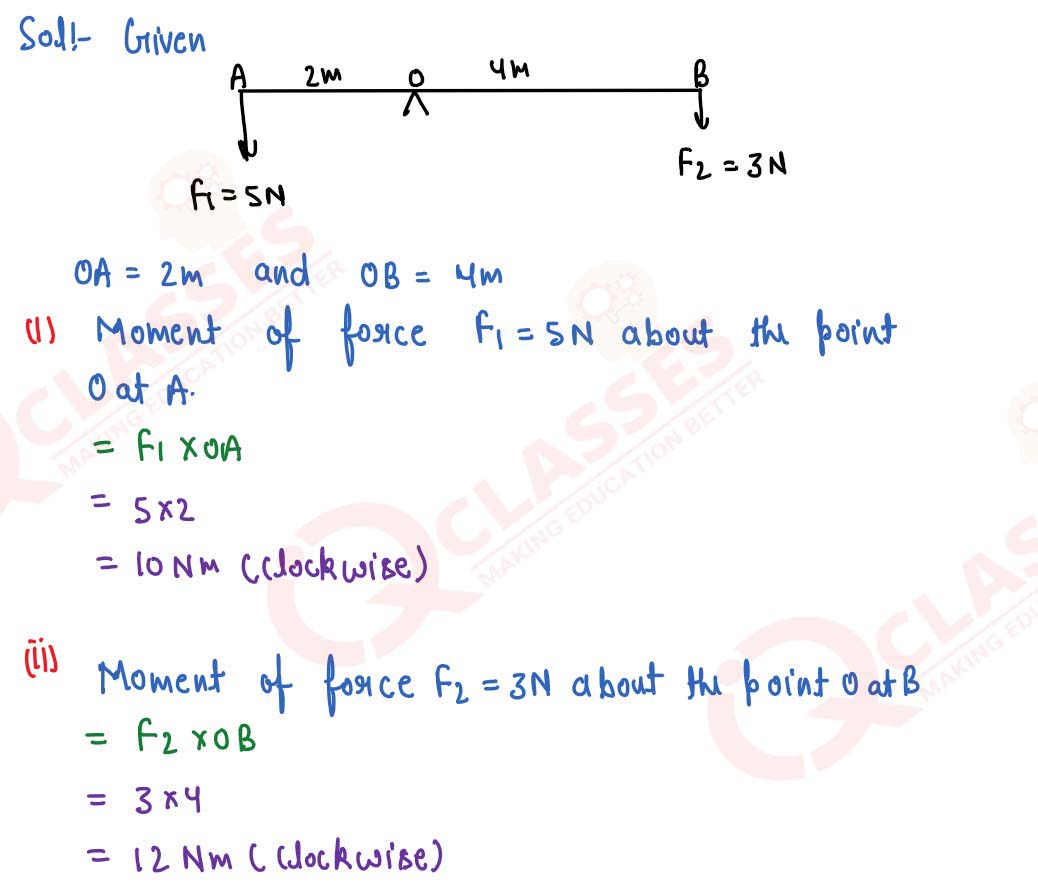

Calculate:
(i) the moment of force F1 about O.
(ii) the moment of force F2 about O.
(iii) total moment of the two forces about O.
solutions

Q5
Two forces each of magnitude 10N act vertically upwards and downwards respectively at the two ends A and B of a uniform rod of length 4m which is pivoted at its mid-point O as shown. Determine the magnitude of resultant moment of forces about the pivot O.
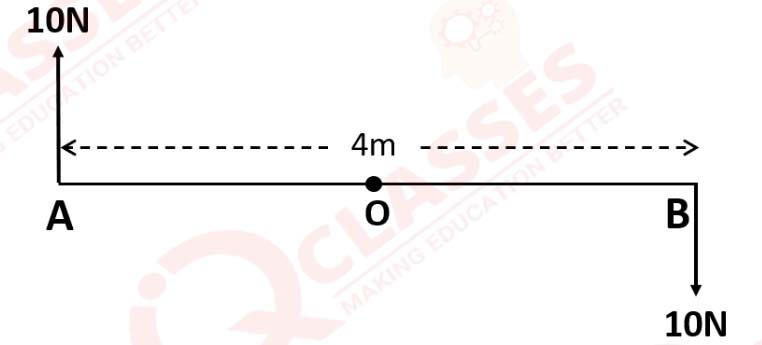
solutions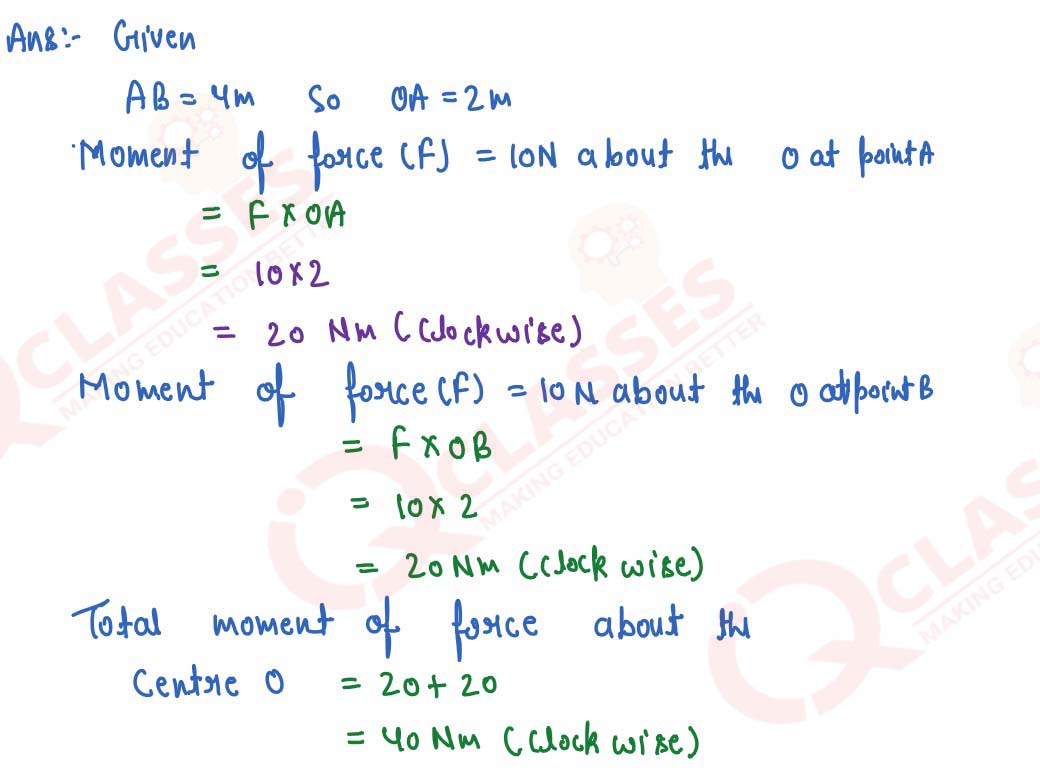

solutions

Q6
Figure shows two forces each of magnitude 10N acting at the points A and B at a separation of 50 cm, in opposite directions. Calculate the resultant moment of the two forces about the point (i) A, (ii) B and (iii) O, situated exactly at the middle of the two forces.
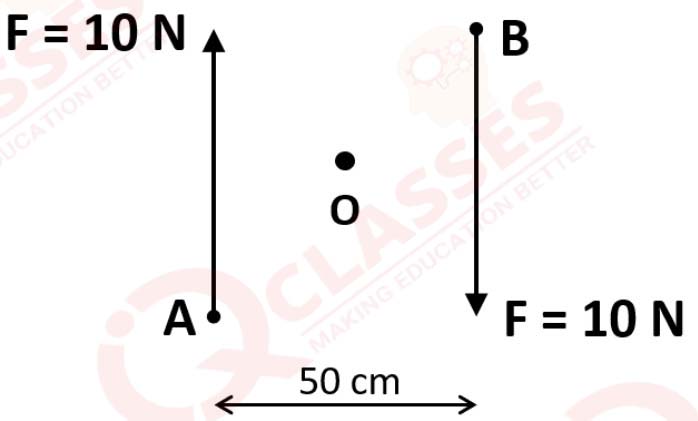
solutions


solutions


Q7
A steering wheel of diameter 0.5m is rotated anti-clockwise by applying two forces each of magnitude 5N. Draw a diagram to show the application of forces and calculate the moment of forces applied.
solutions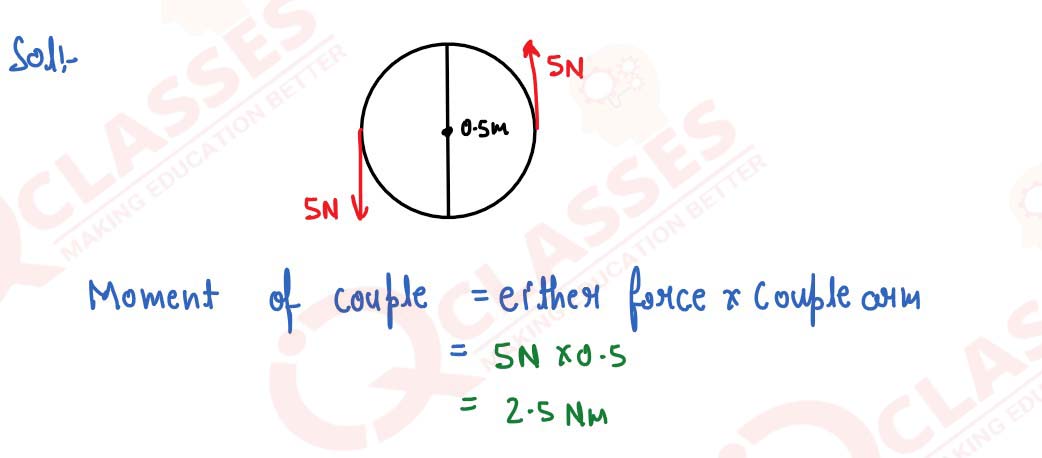
solutions

Q8
A uniform metre rule is pivoted at its mid-point. A weight of 50 gf is suspended at one end of it. Where should a weight of 100gf be suspended to keep the rule horizontal?
solutions
solutions

Q9
A uniform metre rule balances horizontally on a knife edge placed at the 58 cm mark when a weight of 20gf is suspended from one end. (i) Draw a diagram of the arrangement. (ii) What is the weight of the rule?
solutions
solutions

Q10
The diagram shows a uniform bar supported at the middle point O. A weight of 40 gf is placed at a distance 40cm to the left of the point O. How can you balance the bar with a weight of 80 gf?
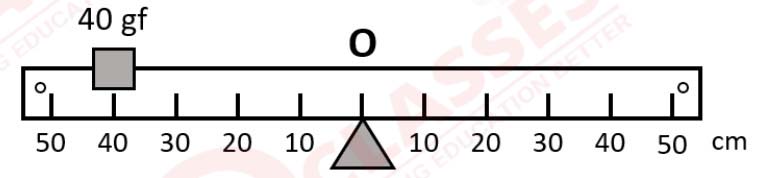
solutions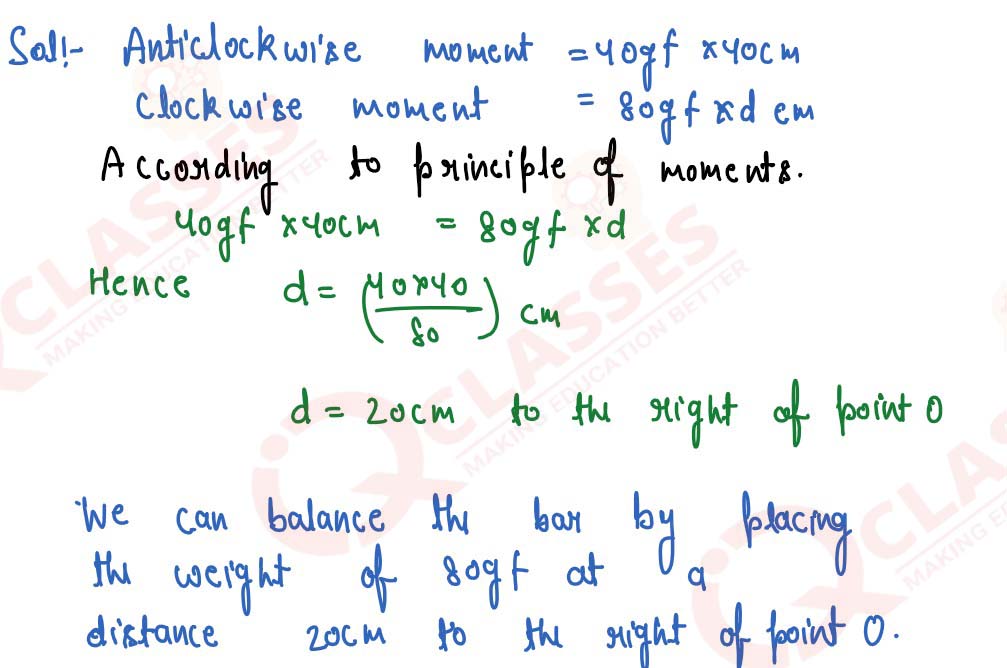

solutions

Q11
Figure shows a uniform metre rule placed on a fulcrum at its mid-point O and having a weight 40gf at the 10 cm mark and a weight of 20 gf at the 90 cm mark.
(i) Is the metre rule in equilibrium? If not, how will the rule turn?
(ii) How can the rule be brought in equilibrium by using an additional weight of 40gf?
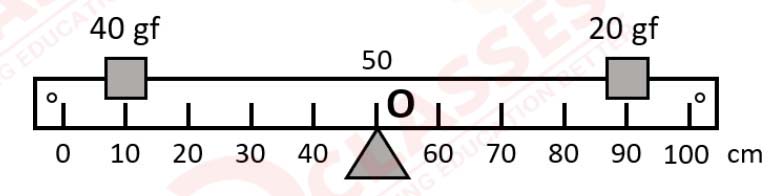
solutions

(i) Is the metre rule in equilibrium? If not, how will the rule turn?
(ii) How can the rule be brought in equilibrium by using an additional weight of 40gf?

solutions


Q12
When a boy weighing 20 kgf sits at one end of a 4m long see-saw, it gets depressed at its end. How can it be brought to the horizontal position by a man weighing 40 kgf.
solutions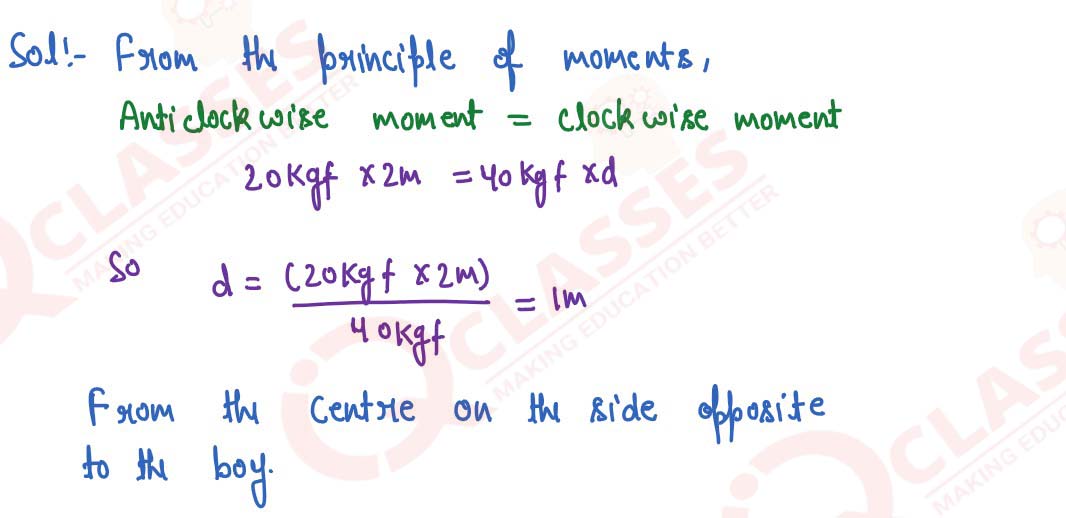
solutions

Q13
A physical balance has its arms of length 60 cm and 40 cm. What weight kept on a pan of longer arm will balance an object of weight 100 gf kept on other pan?
solutions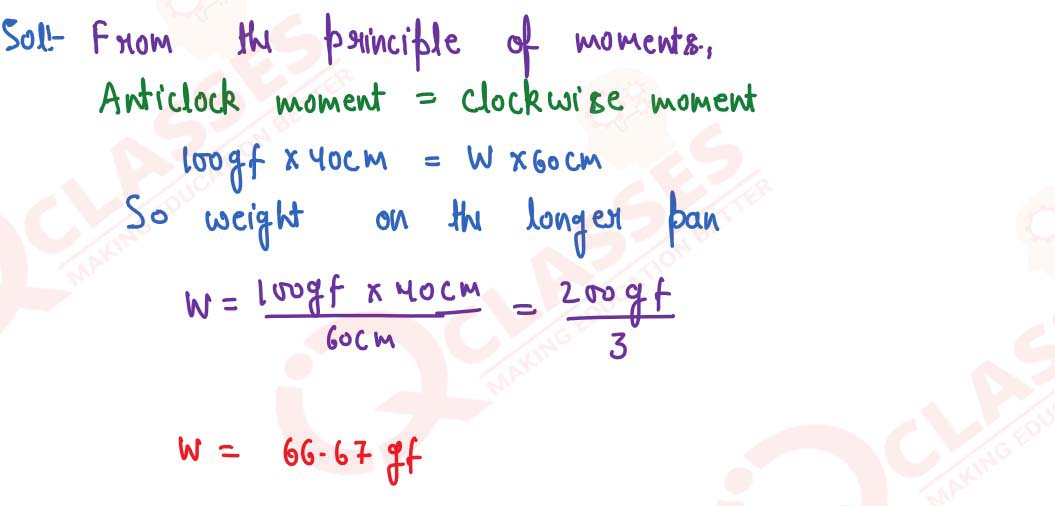
solutions

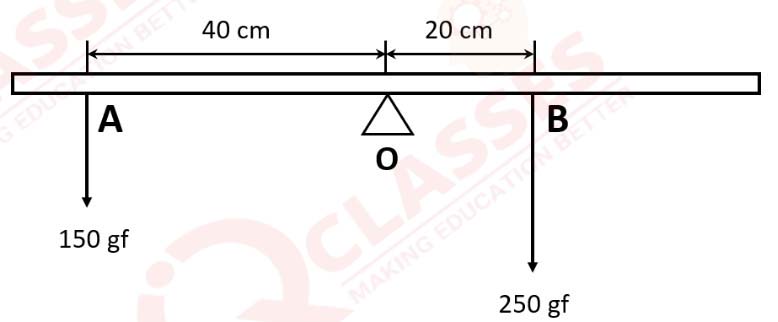
Q14
The diagram shows a uniform meter rule weighing 100 gf, pivoted at its centre O. Two weights 150gf and 250gf hang from the point A and B respectively of the metre rule such that OA = 40 cm and OB = 20 cm.
Calculate:
(i) the total anticlockwise moment about O,
(ii) the total clockwise moment about O,
(iii) the difference of anticlockwise and clockwise moment, and
(iv) the distance from O where a 100gf weight should be placed to balance the metre rule.
solutions
Calculate:
(i) the total anticlockwise moment about O,
(ii) the total clockwise moment about O,
(iii) the difference of anticlockwise and clockwise moment, and
(iv) the distance from O where a 100gf weight should be placed to balance the metre rule.

solutions

Q15
A uniform metre rule of weight 10 gf is pivoted at its 0 mark.
(i) What moment of force depresses the rule?
(ii) How can it be made horizontal by applying a least force?
solutions

(i) What moment of force depresses the rule?
(ii) How can it be made horizontal by applying a least force?
solutions


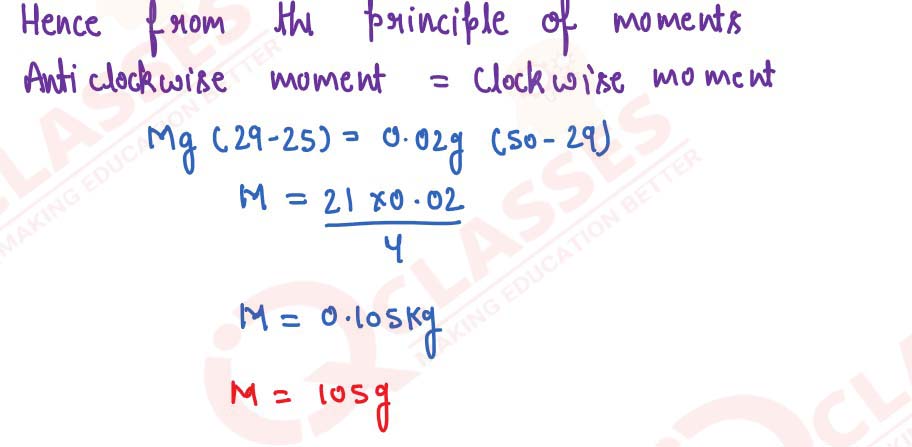
Q16
A uniform half metre rule can be balanced at the 29.0 cm mark when a mass 20g is hung from its one end.
(a) Draw a diagram of the arrangement.
(b) Find the mass of the half metre rule.
(c) In which direction would the balancing point shift if 20g mass is shifted inside from its one end?
solutions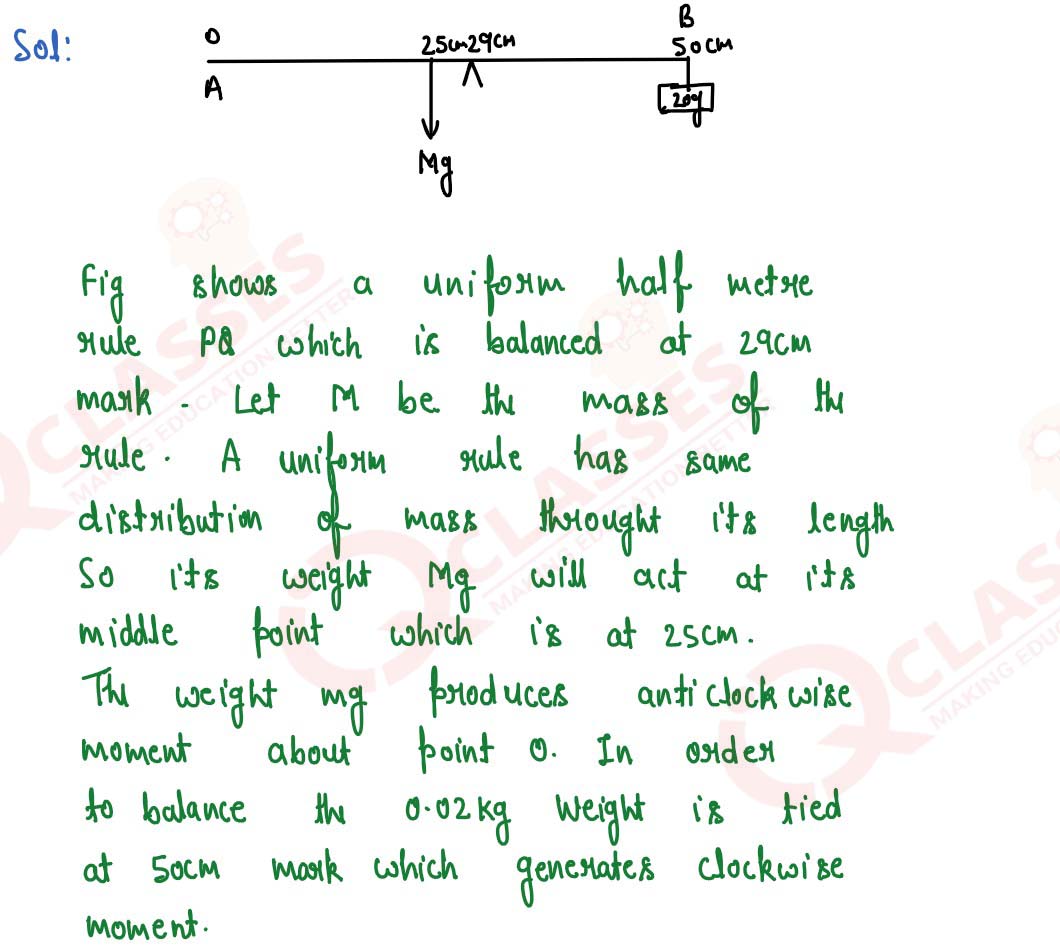
(a) Draw a diagram of the arrangement.
(b) Find the mass of the half metre rule.
(c) In which direction would the balancing point shift if 20g mass is shifted inside from its one end?
solutions


Q17
A uniform metre rule of mass 100 g is balanced on a fulcrum at mark 40 cm by suspending an unknown mass m at the mark 20 cm.
(i) Find the value of m.
(ii) To which side the rule will tilt if the mass m is moved to the mark 10 cm?
(iii) What is the resultant moment now?
(iv) How can it be balanced by another mass 50 g?
solutions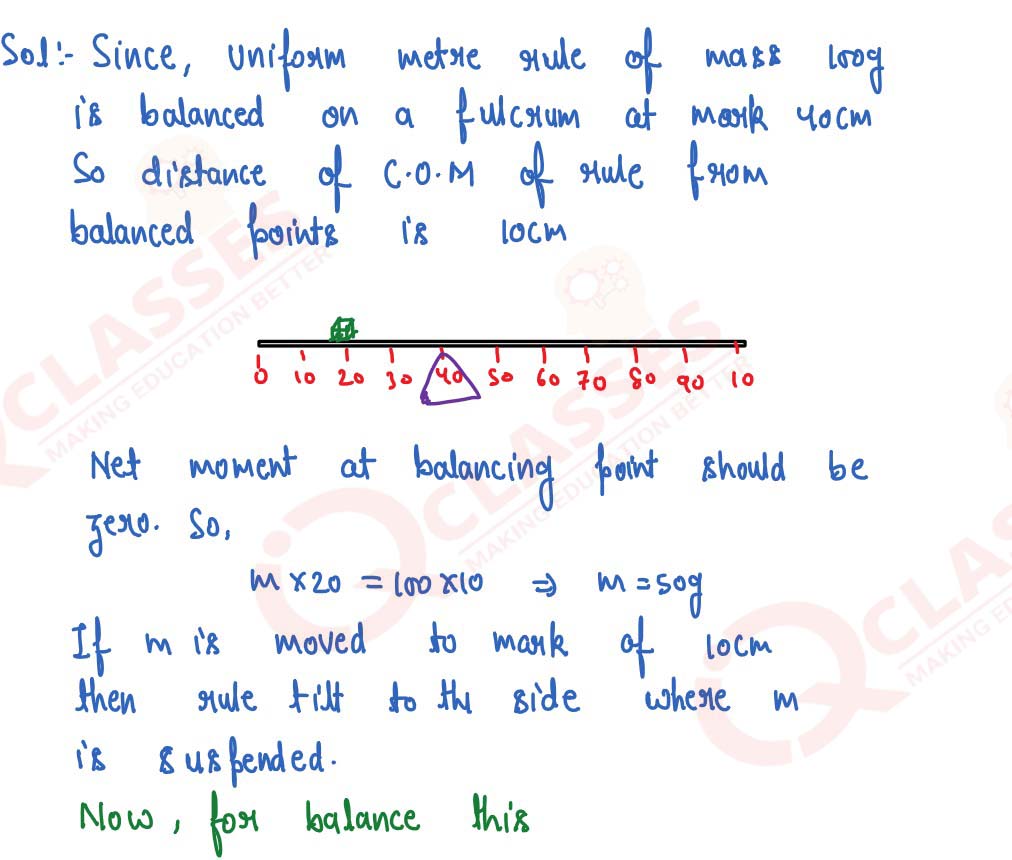

(i) Find the value of m.
(ii) To which side the rule will tilt if the mass m is moved to the mark 10 cm?
(iii) What is the resultant moment now?
(iv) How can it be balanced by another mass 50 g?
solutions


Q18
In figure below, a uniform bar of length l m is supported at its ends and loaded by a weight W kgf at its middle. In equilibrium, find the reactions R1 and R2 at the ends.
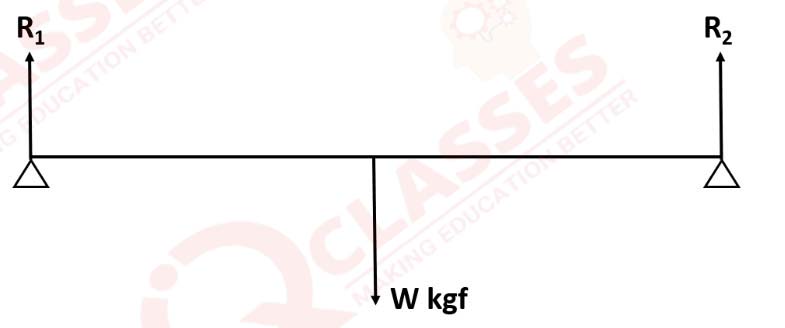
solutions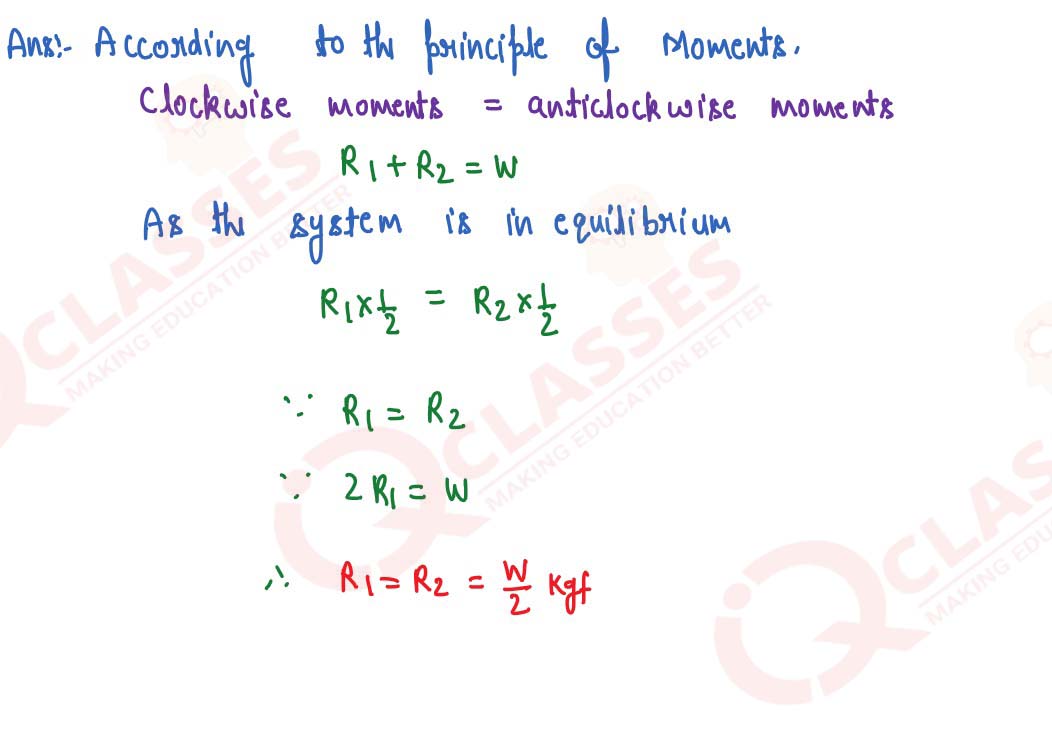

solutions
