Class XII ISC Maths Chapter Relation and Functions Exercise 1.3
Explore our wide range of questions on "Relations & Functions," designed to help you master this fundamental topic in mathematics. Our question bank covers essential concepts, including types of relations, domain and range, types of functions, and their properties, as well as real-world applications. These practice questions are perfect for students looking to strengthen their understanding and problem-solving skills in this topic. Whether you're preparing for exams or enhancing your knowledge, our collection provides the ideal resource to help you excel in "Relations & Functions."
class 12 relation&function exercise1-1
Relations & Functions
Select Exercise
Q1 Let A= {1, 2} B= {3, 6}, function f: A -> B be defined by f(x) = 3x and function g: A → B be defined by g(x) = x2 + 2. Show that f = g
Q2 Check the injectivity and surjectivity of the following functions
(i) f: N→N defined by f(x) = x2
(ii) f: Z →Z defined by f(x) = x2
(iii) f: R→R defined by f(x) = x2
(iv) f: N→Q defined by f(x) = x3
(v) f:Z →Z defined by f(x) = x3
(vi) f:R→R defined by f(x) = x3
Q3 Let I be the set of all integers, Show that the function f: I→I defined by f(x)=|x| is neither one-one nor onto.
Q5 let A= {1, 2, 3},B= (4, 5, 6, 7} and f: {(1, 4), (2, 5), (3, 6)} be a function from A to B. Show that f is one-one but not onto.
Q6 In each of the following cases state whether the function is bijective or not. Justify your answer
(i) f: R →R defined by f(x) = 2x + 1
(ii) f: R → R defined by f(x) = 3 - 4x
(iii) f : R→R defined by f(x) =1 + x2
Q8 Let A = R — {2} and B= R - {1}. Show that the function fi A → B defined by f(x)= x-1 / x-2 is bijective.
Q9 Show that the function f : R -> R defined by f(x) = cos x iS neither one-one nor onto
Q10 Show that the function f: I→I defined by f(x) = x2 + x is neither one-one nor onto .
Q11 If the function f: I -> I is defined by
 find,
find,
(i) f (3)
(ii) f (4)
(iii) x, if f(x) = -9.
Is this function one-one?
Q17 Is the function f : N —> N defined by f(m) = m2 + m + 2 one-one? Justify your answer.
Q21 Find the range of the following functions :
(i) f (x) = sin x + cos x
(ii) f(x) = 3 sin x + 4 cos x.
Q22 The function f: R -> R is defined by f(x) = |2x -3|.
(i) Is f one-one?
(ii) Is f onto?
Q24 If A = {a, b, c} and B = {1, 2, 3, 4}, then find the number of functions from A to B.
Q25 If A = {a, b} and B = {1, 3, 5}, then find the number of one-one functions from A to B.
Q26 If A = {a, b, c} and B = {p, q}, then find the number of onto functions from A to B.
Q27 If A = {1, 2, 3, 4} and B = {-1, 3}, then find the number of onto functions from A to B.
Q28 If A = {1, 2, 3} and B = {a, B, c}, then find the number of one-one onto functions from A to B.
Q29 If A = {1, 2, 3, 4} and B = {a, b, c}, then find the number of bijections from A to B.

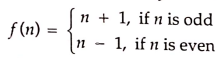 find,
find,

Add a comment