Class XII ISC Maths Chapter Linear Programming Exercise 3.2
Explore our extensive collection of Linear Programming questions, tailored for class 12 students following the ISC curriculum. This topic is vital for understanding how to optimize a particular outcome given certain constraints. Our practice questions cover various aspects of Linear Programming, including formulating linear inequalities, graphical methods, and finding optimal solutions. Whether you're looking to strengthen your problem-solving skills or prepare for your exams, these questions provide the perfect resource to master Linear Programming concepts with ease.
class 12 ITF exercise2-1
Linear Programming
Select Exercise
Q(6) Minimize Z = 200x + 500y, subject to the constraints
x + 2y≥10, 3x + 4y≤24,x≥0,y≥0
Q(7) Minimize Z = x + 2y subject to
2x +y≥3,x+2y≥6,x≥0,y≥0
Show that the minimum value of Z occurs at more than two points.
Q(8) Minimize and maximize Z = 3x + 9y subject to the constraints
x+y≥10,x+3y≤60,x≤ y,x≥0,y≥0
Q(11) Minimize and maximize Z = x + 2y subject to the constraints
x + 2y≥100,2x -y≤0, 2x + y≤200,x≥0,y≥0
Q(13) A manufacturer considers that men and women workers are equally efficient and so he pays them at the same rate. He has 30 and 17 units of workers (male and female) and capital respectively, which he uses to produce two types of goods A and B. To produce one unit of A, 2 workers and 3 units of capital are required while 3 workers and 1 unit of capital is required to produce one unit of B. If A and B are priced at ₹100 and ₹120 per unit respectively, how should he use his resources to maximise the total revenue? Form the above as an L.P.P. and solve graphically.
Do you agree with this view of the manufacturer that men and women workers are equally efficient and so should be paid at the same rate?
Q(14) A company produces two types of items P and Q. Manufacturing of both items requires the metals gold and copper. Each unit of item P requires 3 gms of gold and 1 gm of copper while that of item Q requires 1 gm of gold and 2 gms of copper. The company has 9 gms of gold and 8 gms of copper in store. If each unit of item P makes a profit of ₹50 and each unit of item Q makes a profit of ₹60, determine the number of units of each item that the company should produce to maximize profit.What is the maximum profit?
Q(15) A cooperative society of farmers has 50 hactare of land to grow two crops X and Y. The profit from crop X and Y per hectare are estimated as ₹10500 and ₹9000 respectively. To control weeds, a liquid herbicide has to be used for crops X and Y at the rate of 20 litres and 10 litres per hectare. Further, no more than 800 litres of herbicide should be used in order to protect fish and wild life using a pond which collects drainage from this land.
Keeping in mind that the protection of fish and other wildlife is more important than earning profit, how much land be allocated to each crop so as to maximize the total profit? Form an L.P.P. from the above and solve it graphically. Do you agree with the message that the protection of wildlife is utmost necessary to preserve the balance in environment
Q(16) One kind of cake requires 200 g of flour and 25 g of fat, and another kind of cake requires 100 g of flour and 50 g of fat. Find the maximum number of cakes which can be made from 5 kg of flour and 1 kg of fat assuming that there is no shortage of other ingredients used in making the cakes.Formulate the above as a linear programming problem and solve graphically.
Q(17) A company manufactures two types of toys A and B. A toy of type A requires 5 minutes for cutting and 10 minutes for assembling. A toy of type B requires 8 minutes for cutting and 8 minutes for assembling. There are 3 hours available for cutting and 4 hours available for assembling the toys in a day. The profit is ₹50 each on a toy of Type A and ₹60 each on a toy of type B. How many toys of each type should the company manufacture in a day to maximize the profit? Use linear programming to find the solution.
Q(18) A manufacturer manufactures two types of plastic bags A and B using two machines M1 and M2 .To manufacture a bag of type A, machine M1 is operated for 2 minutes and machine M2 for 1 minute; to manufacture a bag of type B, machine M1 is operated for 3 minutes and machine M2 for 4 minutes. Each machine can be operated for atmost 8 hours per day. A bag of type A is sold at a profit of ₹0.50 and a bag of type B is sold at a profit of ₹0.75. How many bags of each type should be manufactured and sold per day so as to maximise his profit. Form an L.P.P. and solve it graphically. Why plastic bags should not be used? What values are being promoted?
Q(19) A small firm manufactures gold rings and chains. The combined number of rings and chains manufactured per day is atmost 24. It takes one hour to make a ring and half an hour for a chain. The maximum number of hours available per day is 16. If the profit on a ring is ₹300 and on a chain is ₹190, how many of each should be manufactured daily so as to maximize the profit?
Q(20) A factory manufactures two types of screws, A and B, each type requiring the use of two machines —an automatic and a hand-operated. It takes 4 minutes on the automatic and 6 minutes on the hand-operated machine to manufacture a package of screws ‘A’, while it takes 6 minutes on the automatic and 3 minutes on the hand-operated machine to manufacture a package of screws ‘B’. Each machine is available for atmost 4 hours on any day. The manufacturer can sell a package of screws ‘A’ at a profit of ₹7 and of screws ‘B’ at a profit of ₹10. Assuming that he can sell all the screws he can manufacture, how many packages of each type should the factory owner produce in a day in order to maximize his profit? Determine the maximum profit.
Q(21) A dealer in rural area wishes to purchase a number of sewing machines. He has only ₹57600 to invest and has space for atmost 20 items. An electronic sewing machine costs him ₹3600 and a manually operated sewing machine ₹2400. He can sell an electronic sewing machine at a profit of ₹220 and a manually operated sewing machine at a profit of ₹180. Assuming that he can sell all the items that he can buy, how should he invest his money in order to maximize his profit. Make it as an L.P.P. and solve it graphically.
Q(22) A cottage industry manufactures pedestal lamps and wooden shades, each requiring the use of a grinding /cutting machine and a sprayer. It takes 2 hours on the grinding /cutting machine and 3 hours on the sprayer to manufacture a pedestal lamp while it takes 1 hour on the grinding / cutting machine and 2 hours on the sprayer to manufacture a shade.
To keep the noise and dust pollution under prescribed limits, a sprayer can be used for a maximum of 20 hours per day while cutting and grinding machine can be used for a maximum of 12 hours per day. The profit from the sale of a lamp is ₹5 and that from sale of a shade is ₹3. Assuming that manufacturer can sell all the pedestal lamps and shades that are made, how should he schedule his daily production to maximise profit? Make it as an L.P.P. and solve it graphically. Which value is indicated in the above question?
Q(23) A company manufactures two types of novelty souvenirs made of plywood. Souvenirs of type A require 5 minutes each for cutting and 10 minutes each for assembling. Souvenirs of type B require 8 minutes each for cutting and 8 minutes each for assembling. There are 3 hours 20 minutes available for cutting and 4 hours available for assembling. The profit is ₹5 each for type A and ₹6 each for type B souvenirs. How many souvenirs of each type should the company manufacture in order to maximize the profit?
Q(24) A small scale factory makes two types of dolls. One doll of type I takes 1.5 hours of electronic machine and 3 hours of hand operated machine; one doll of type II takes 3 hours of electronic machine and 1 hour of hand operated machine. In a day, the factory has the ability of atmost 42 hours of electronic machines and 24 hours of hand operated machines. If the profit on one doll of type I is ₹20 and on one doll of type II is ₹30, find the number of dolls of each type that the factory should manufacture to earn maximum profit. Make it as an L.P.P. and solve graphically. Why are small scale industries important in India? What values are being promoted by establishing small scale industry?
Q(25) An aeroplane can carry a maximum of 200 passengers. Baggage allowed for a first class ticket is 30 kg and for an economy class ticket is 20 kg. Maximum capacity for the baggage is 4500 kg. The profit on each first class ticket is ₹500 and on each economy class ticket is ₹300. Determine how many tickets of each type must be sold to maximize the profit of the airline. Also find the maximum profit.
Q(26) Two tailors P and Q earn ₹150 and ₹200 per day respectively. P can stich 6 shirts and 4 trousers a day, while Q can stich 10 shirts and 4 trousers per day. How many days should each work to produce atleast 60 shirts and 32 trousers at minimum labour cost?
Q(27) Reshma wishes to mix two types of foods P and Q in such a way that the vitamin contents of the mixture contains atleast 8 units of vitamin A and 11 units of vitamin B. Food P costs ₹60/kg and food Q costs ₹80/kg. Food P contains 3 units/kg of vitamin A and 5 units/kg of vitamin B while food Q contains 4 units/kg of vitamin A and 2 units/kg of vitamin B. Determine the minimum cost of the mixture
Q(28) There are two types of fertilisers F1 and F2. F1 consists of 10% nitrogen and 6% phosphoric acid and F2 consists of 5% nitrogen and 10% phosphoric acid. After testing the soil conditions, a farmer finds that she needs atleast 14 kg of nitrogen and 14 kg of phosphoric acid for her crop. If F1 costs ₹6/kg and F2 costs ₹5/kg, determine how much of each type of fertiliser should be used so that neutrient requirements are met at a minimum cost. What is the minimum cost?
Q(29) David wants to invest atmost ₹12000 in Bonds A and B. According to the rule, he has to invest atleast ₹2000 in Bond A and atleast ₹4000 in Bond B. If the rates of interest on Bonds A and B respectively are 8% and 10% per annum, formulate the problem as L.P.P. and solve it graphically for maximum interest. Also determine the maximum interest received in a year.
Q(30) A farmer mixes two brands P and Q of cattle feed. Brand P costing ₹250 per bag, contains 3 units of nutritional element A, 2.5 units of element B and 2 units of element C. Brand Q costing ₹200 per bag contains 1.5 units of nutritional element A, 11.25 units of element B and 3 units of element C. The minimum requirements of nutrients A, B and C are 18 units, 4.5 units and 24 units respectively. Determine the number of bags of each brand which should be mixed in order to produce a mixture having a minimum cost.
Q(31) A toy company manufactures two types of dolls, A and B. Market tests and available resources indicated that the combined production level should not exceed 1200 dolls per week and the demand of the dolls of B type is atmost half of that for dolls of type A. Further, the production level of dolls of type A can exceed three times the production of dolls of other type by atmost 600. If the company makes profit of ₹12 and ₹16 per doll respectively on dolls A and B, how many of each type should be produced weekly in order to maximize the profit?
Q(32) An oil company has two depots A and B with capacities of 7000 litre and 4000 litre respectively. The company is to supply oil to three petrol pumps D, E and F, whose requirements are 4500 litre, 3000 litre and 3500 litre respectively. The distance (in km) between the depots and the petrol pumps is given in the following table :
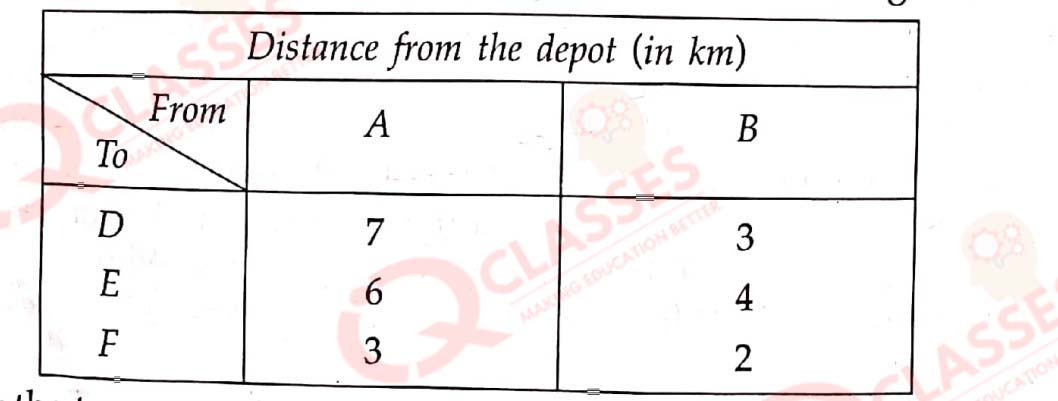
Assuming that transportation cost per km is ₹1 per litre,how should the delivery be scheduled in order that the transportation cost is minimum? What is the minimum cost?


Add a comment