1
What is an alternating current (AC)?
Show that the average value of AC over a complete cycle is zero while for a half-cycle it is 2Io/π, where Io is the peak value of the current. Solution
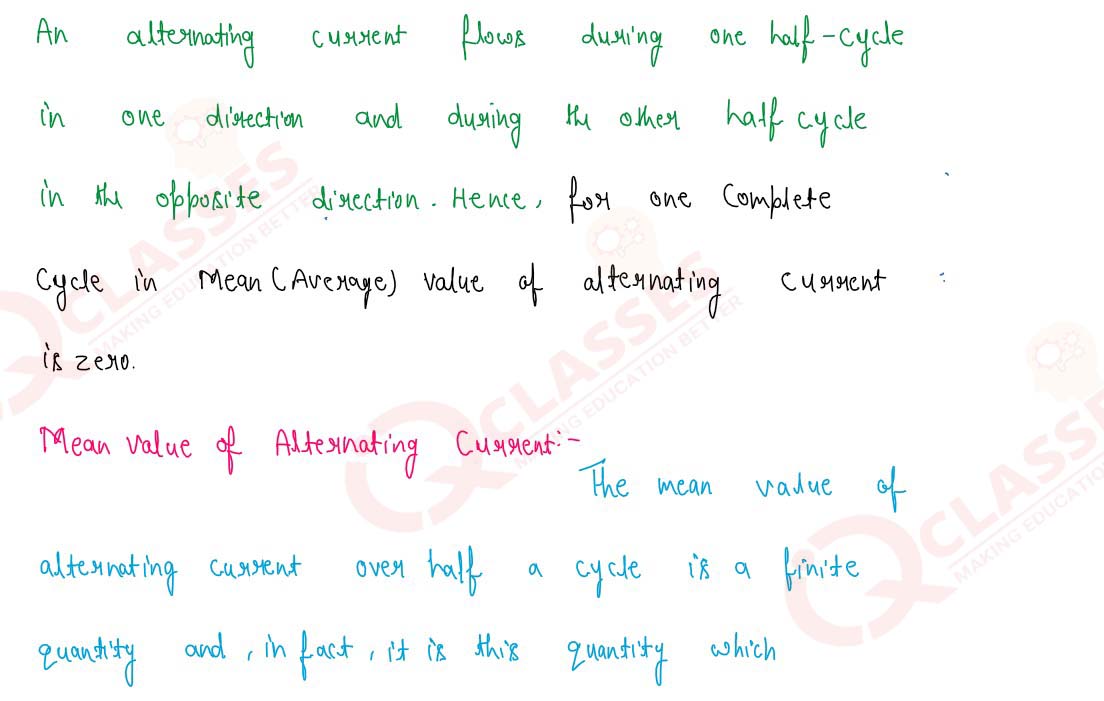
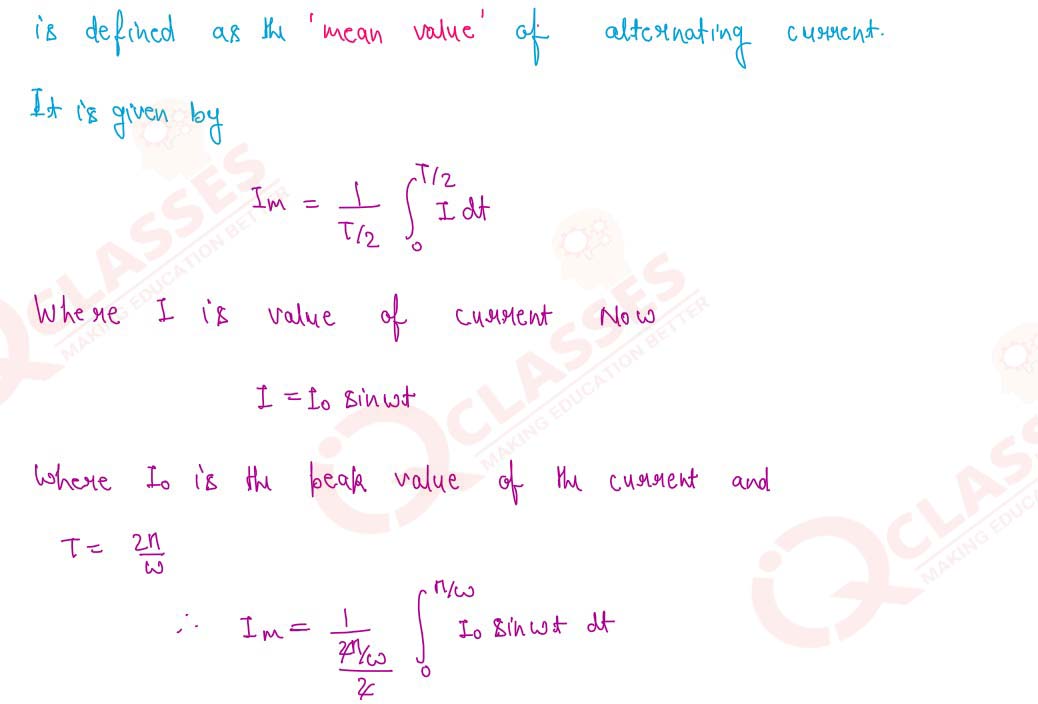
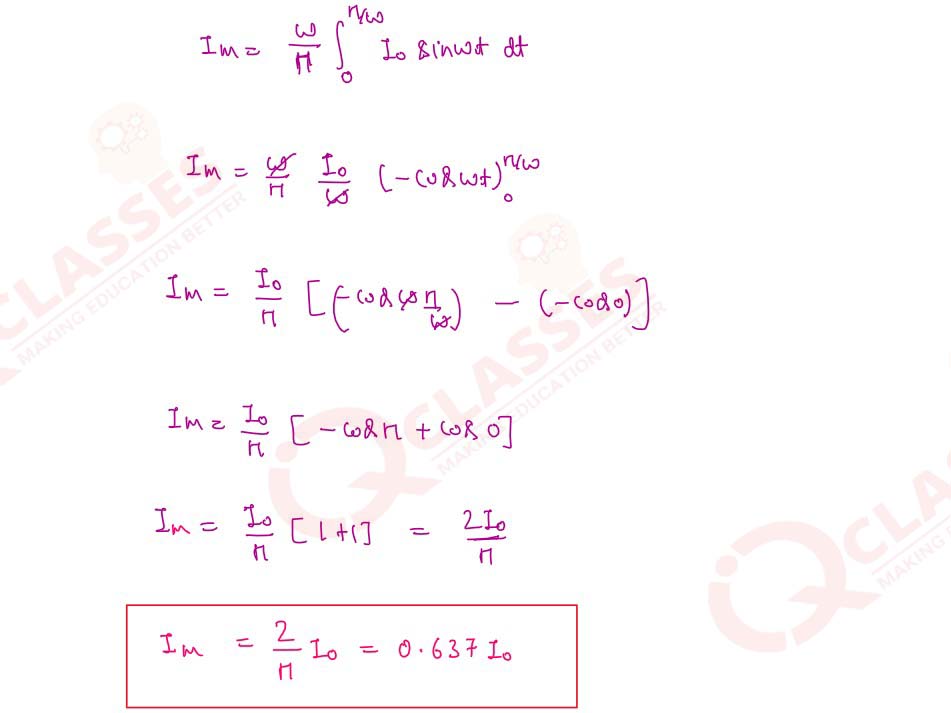
Show that the average value of AC over a complete cycle is zero while for a half-cycle it is 2Io/π, where Io is the peak value of the current. Solution




2
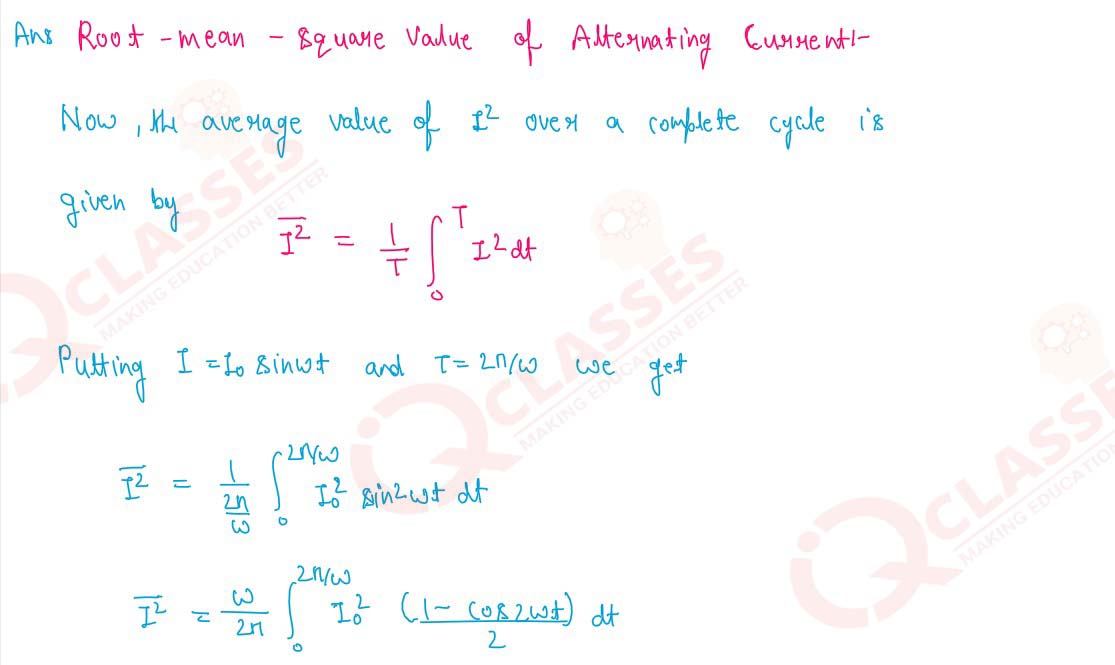
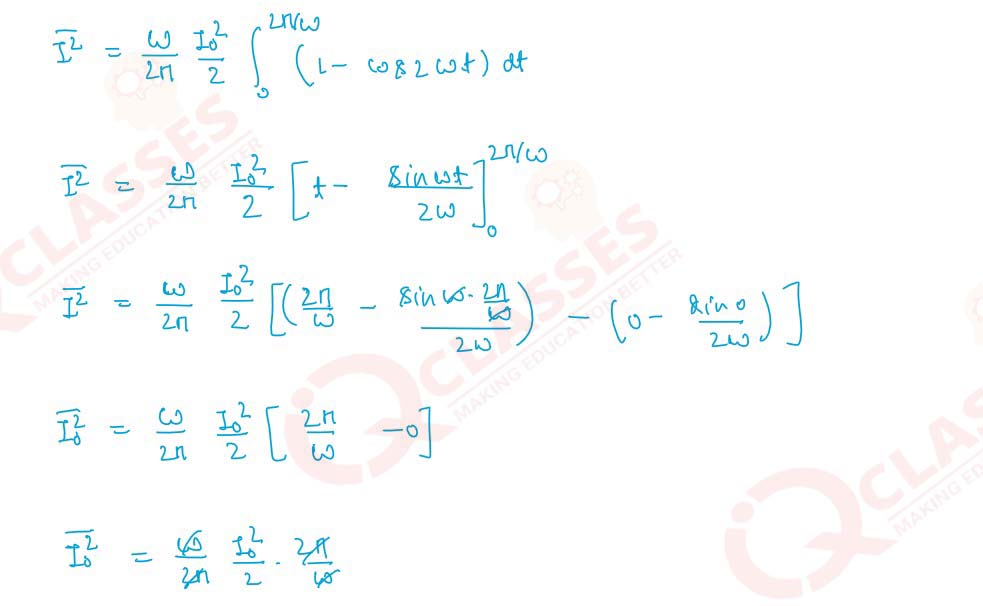
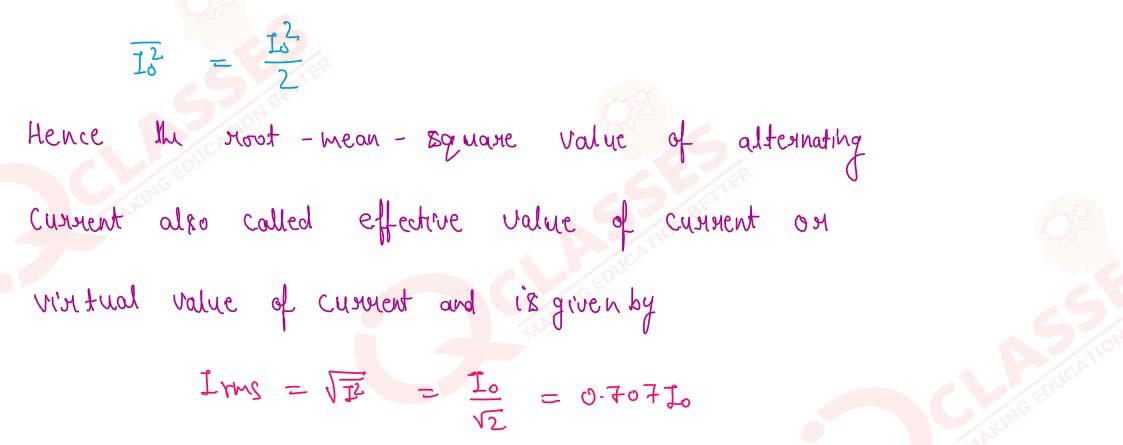
Show that Irms=lo/√2, where Irms is the root- mean-square value of the
alternating
current and lo is its
peak value.
Solution



3
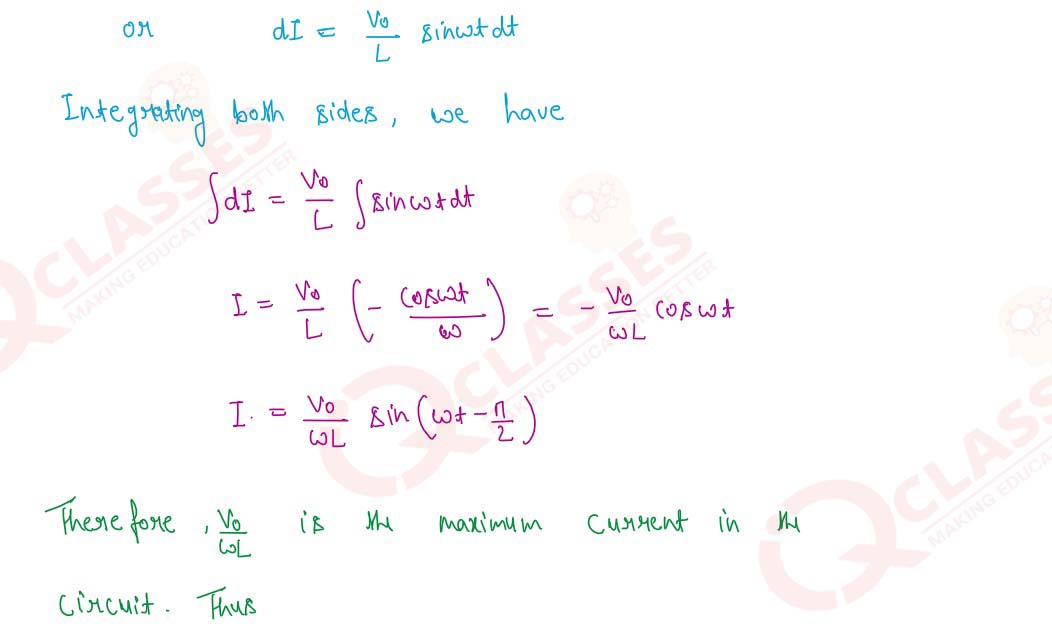
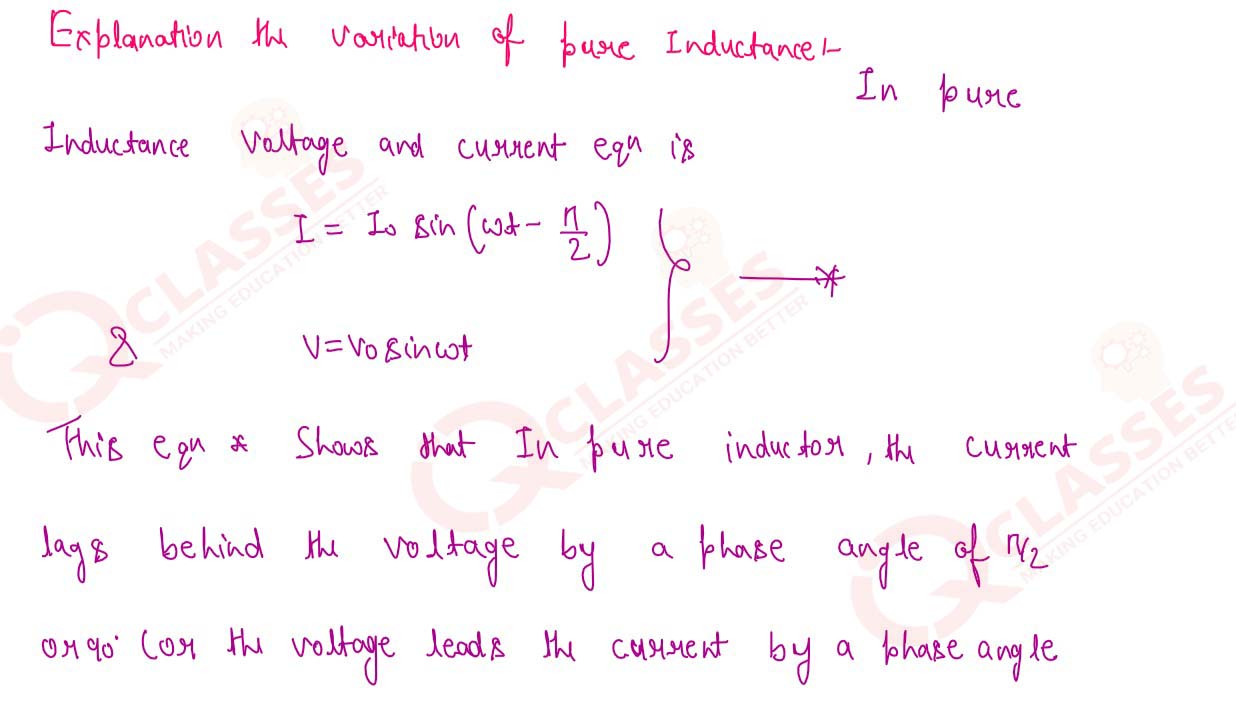
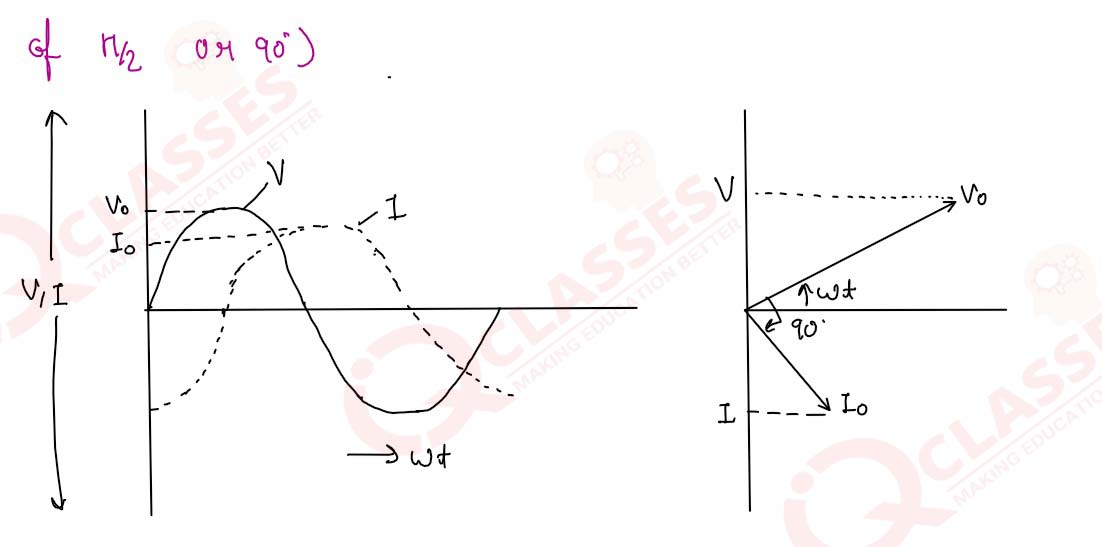
Show mathematically that in an AC circuit containing
inductance only, the current lags behind the voltage
by π/2
Solution

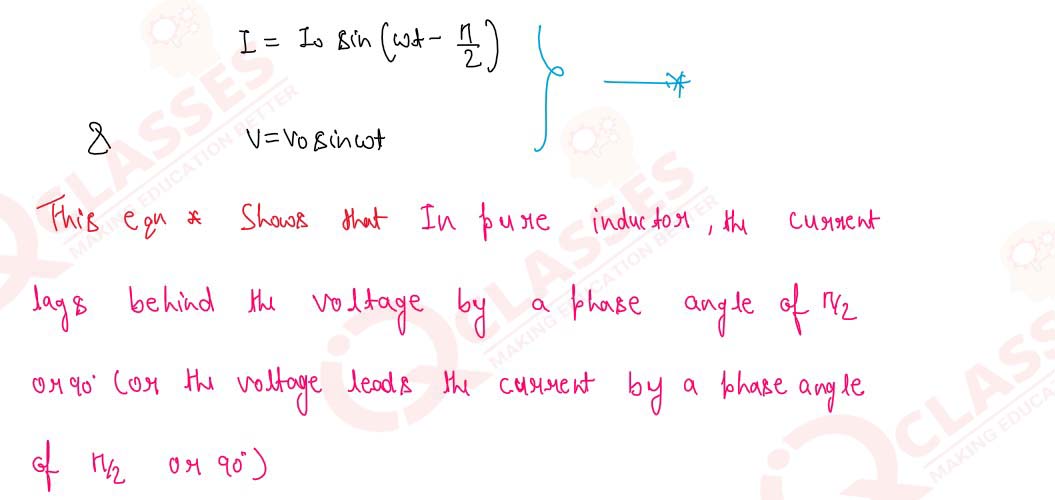


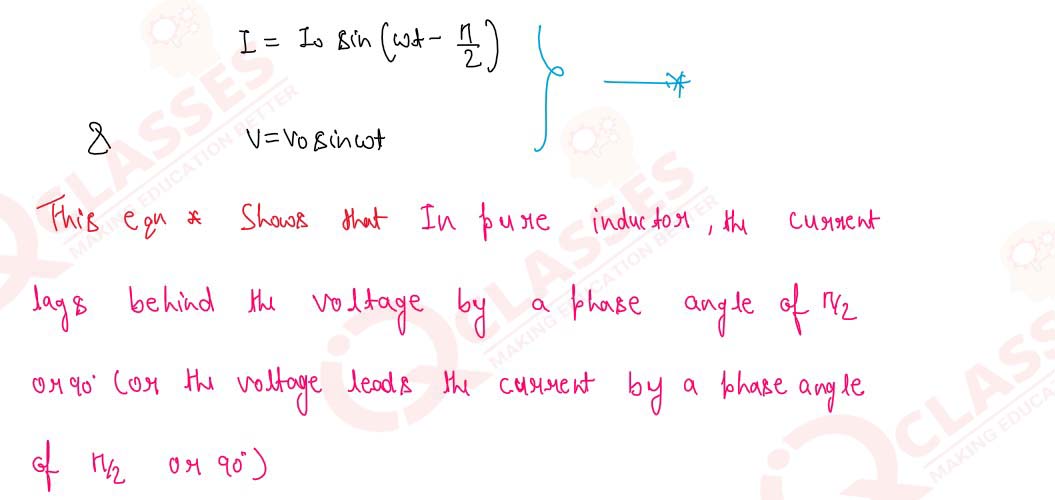

4
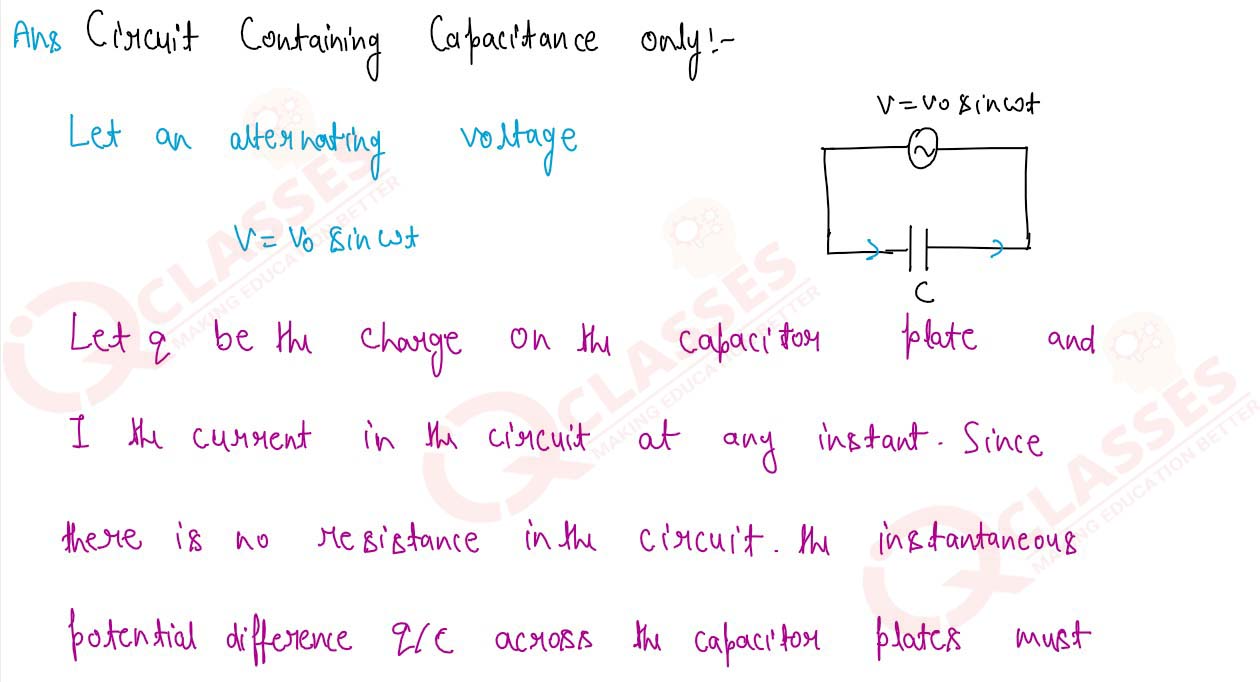
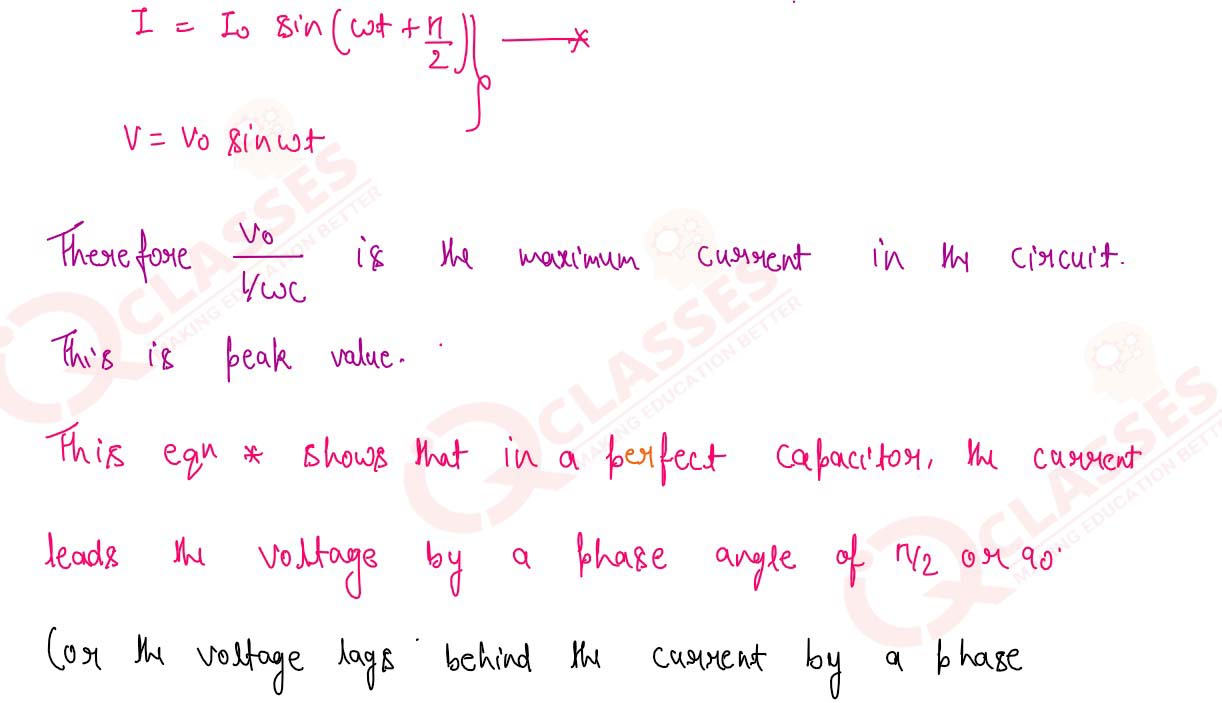
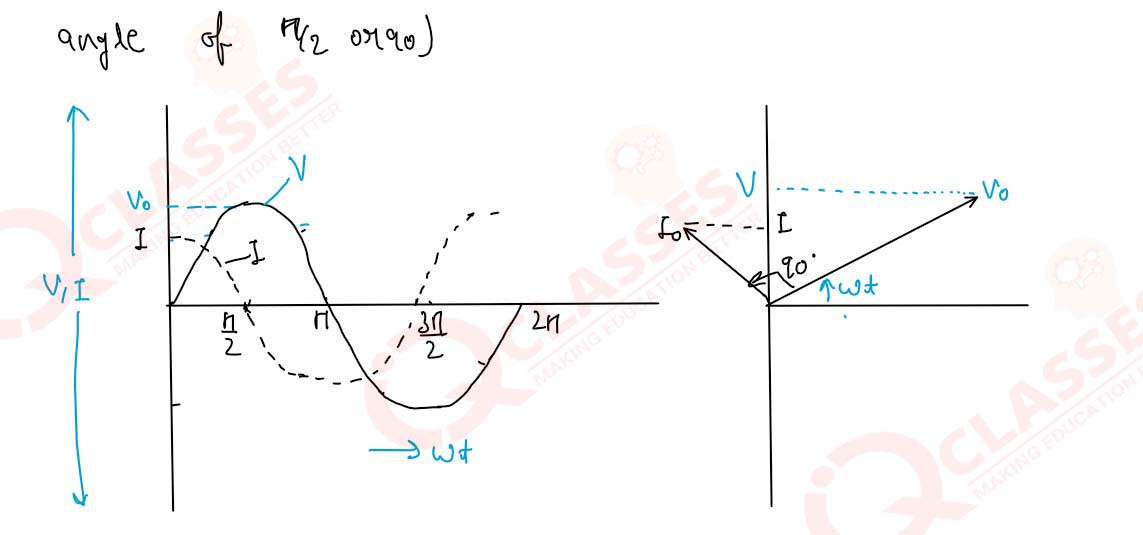
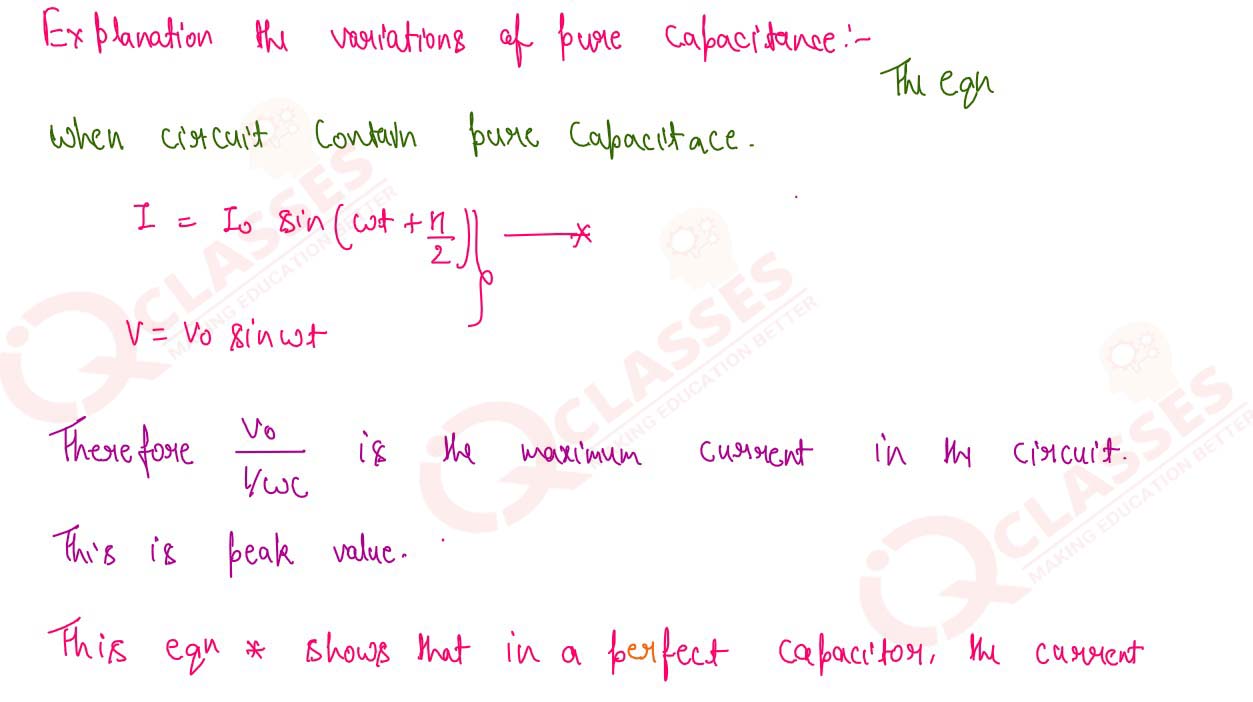
Show mathematically that in a pure capacitive AC circuit, the current leads the voltage by r/2.
Solution
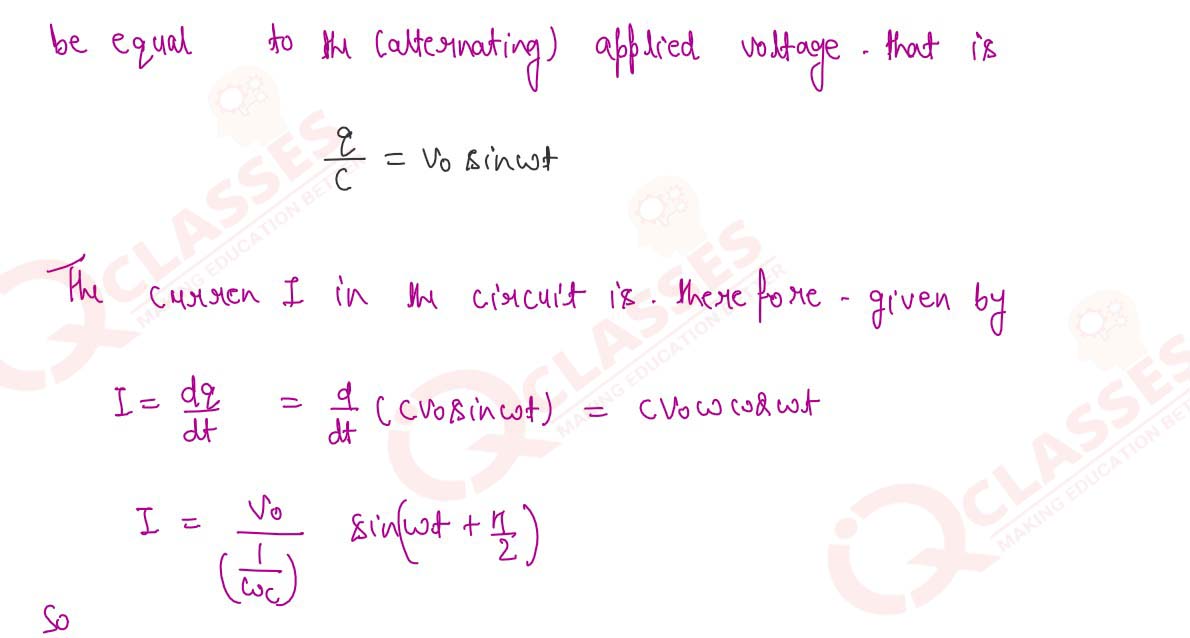

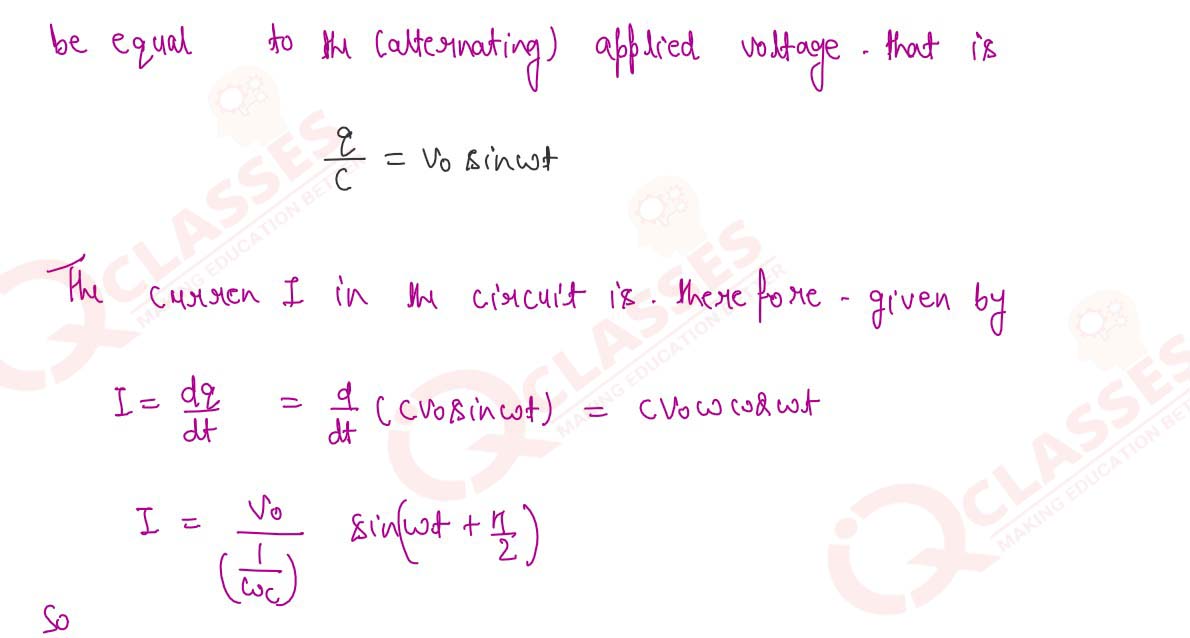


5
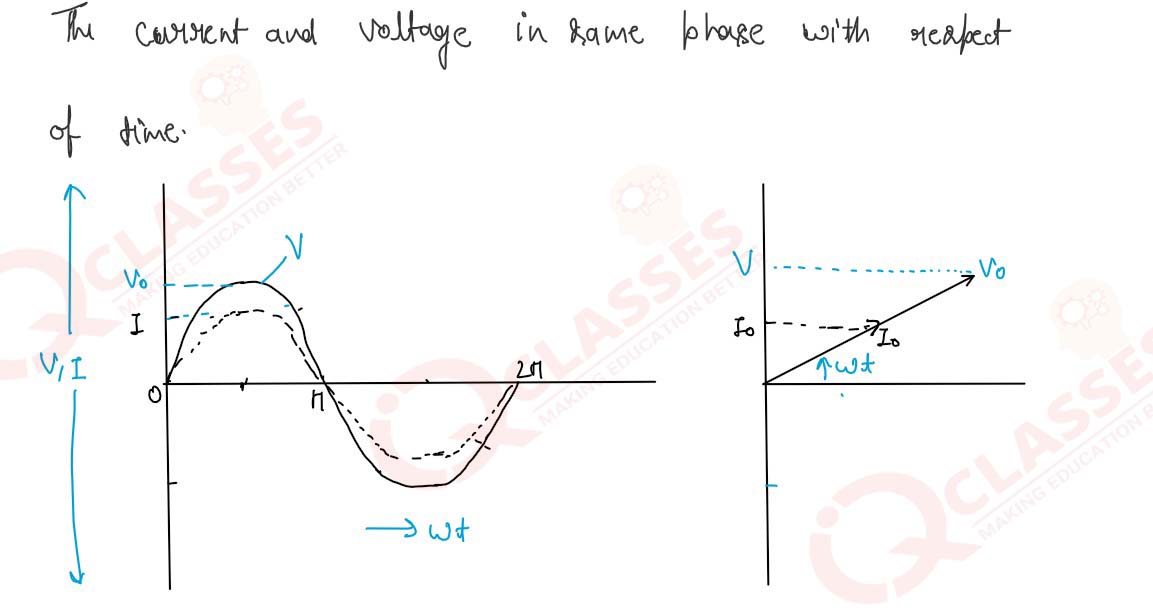
Show, with explanation, the variations of emf and current with time in AC circuits having pure
resistance, pure inductance and pure capacitance.
Solution








6
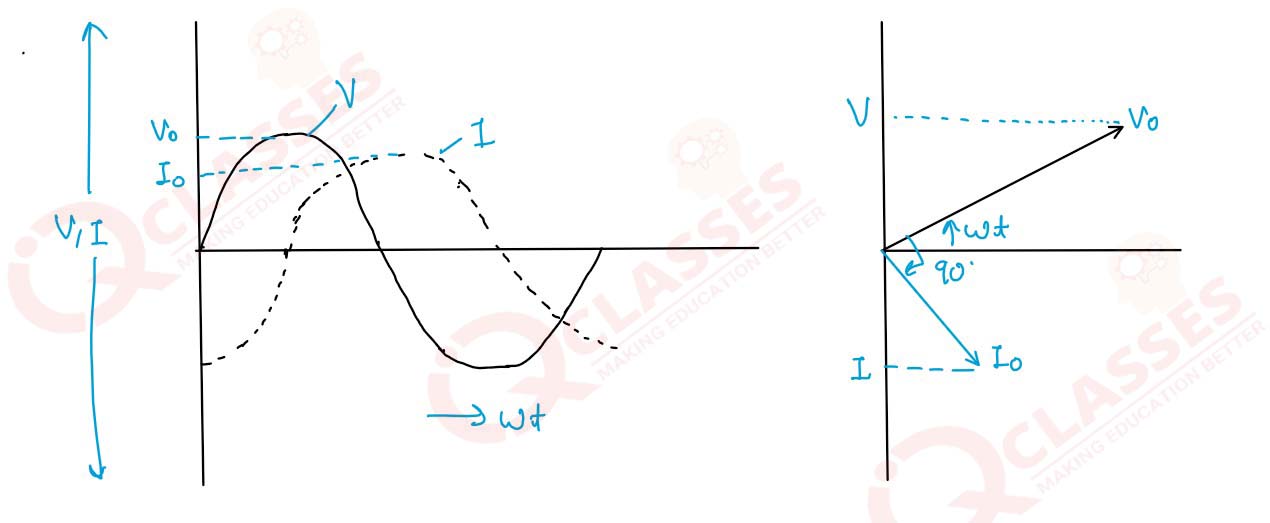
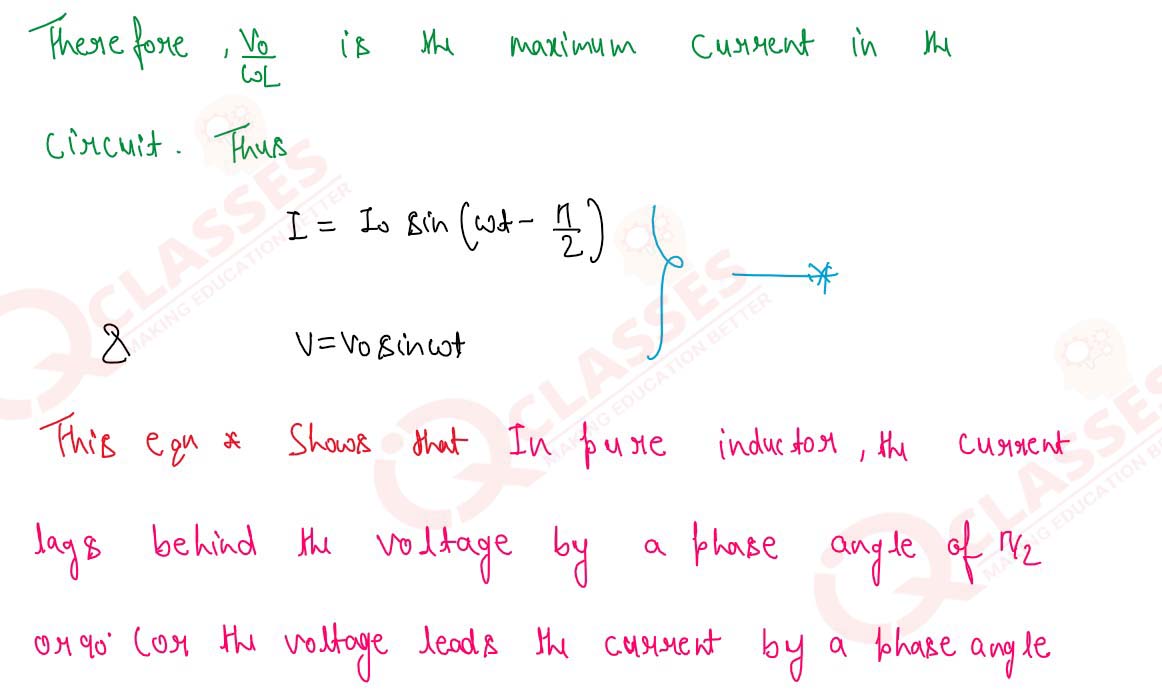
An AC emf V = Vo sin wt is applied across a pure inductance L. Obtain expressions for (i) the
current I
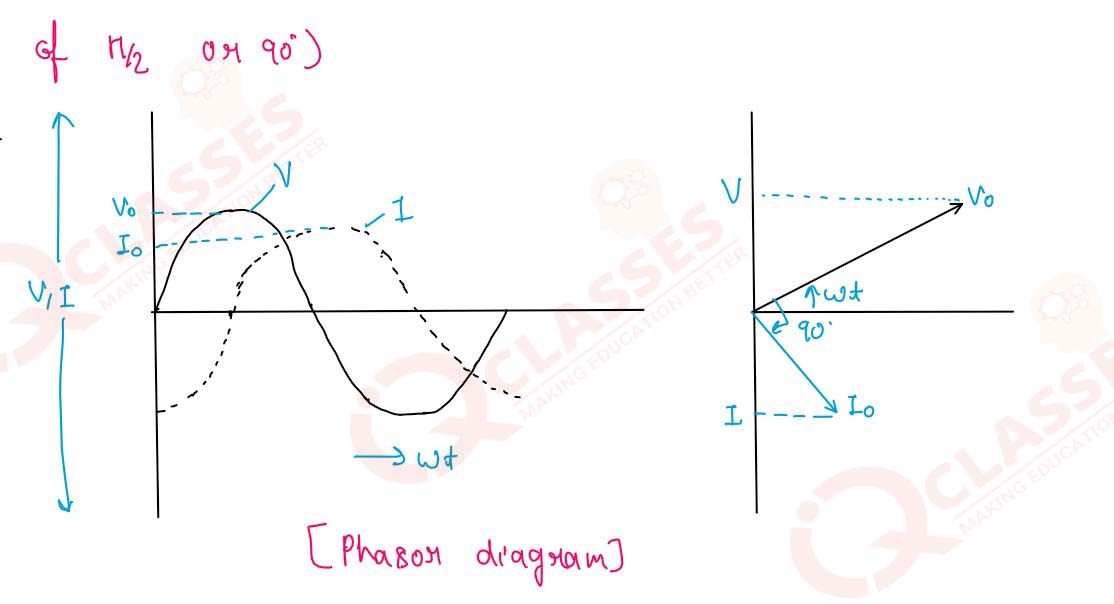
and (ii) the inductive reactance XL in the circuit. Draw a phasor diagram showing emf
Vo,
current lo and
their phase difference.
Solution

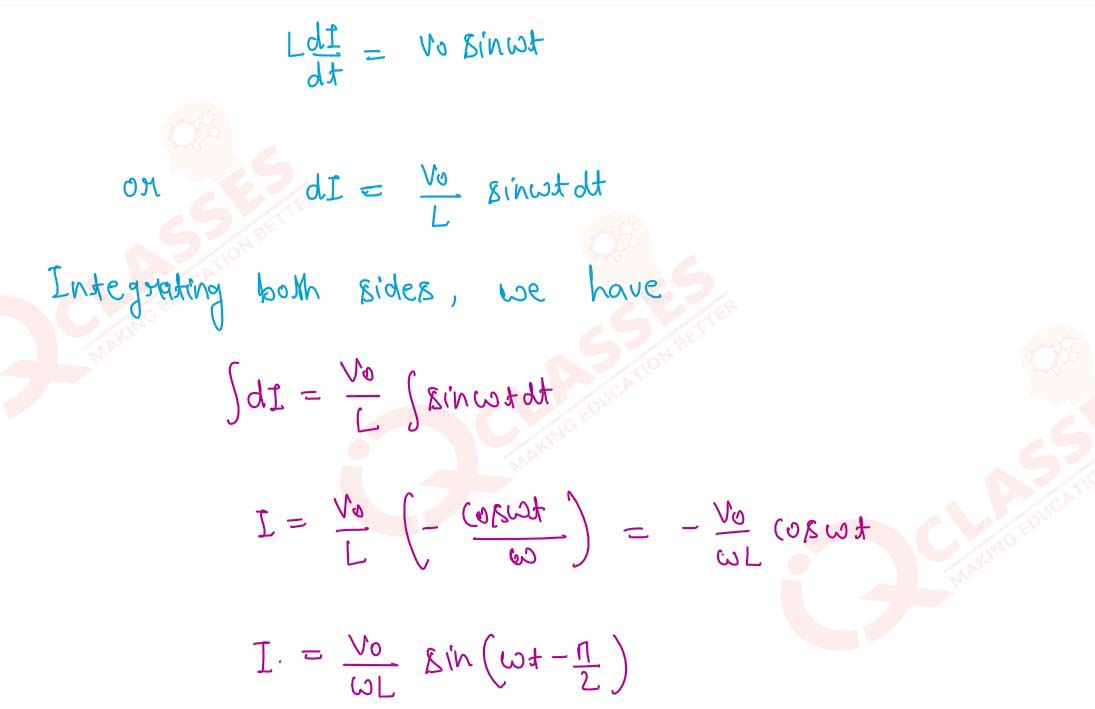


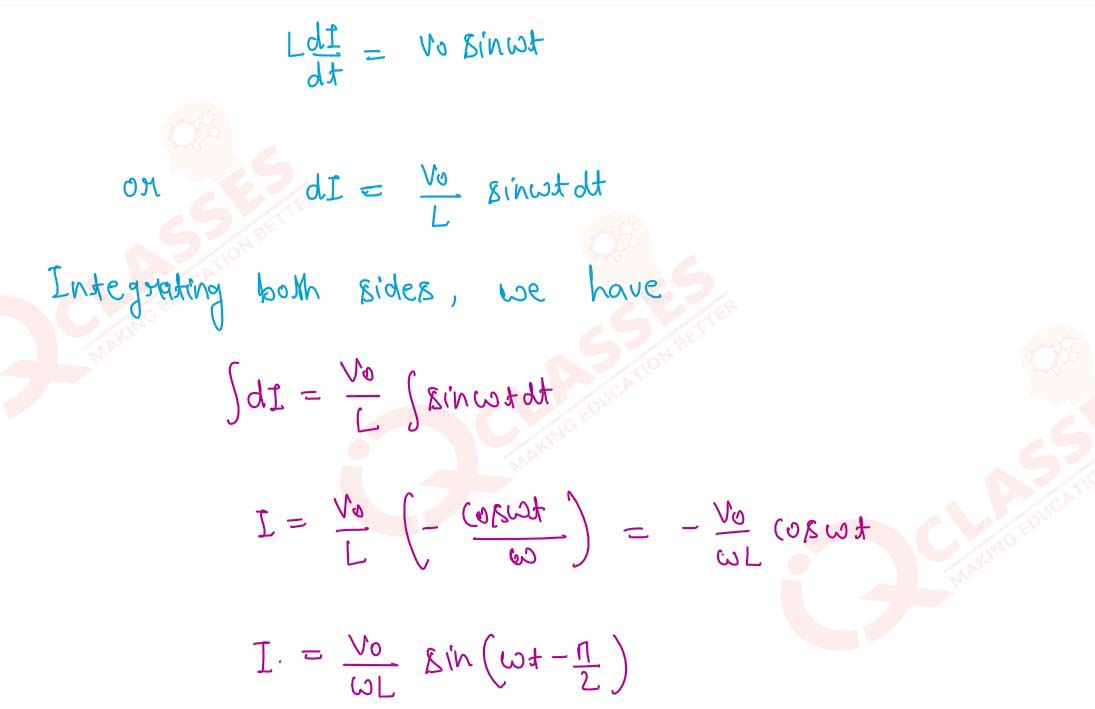




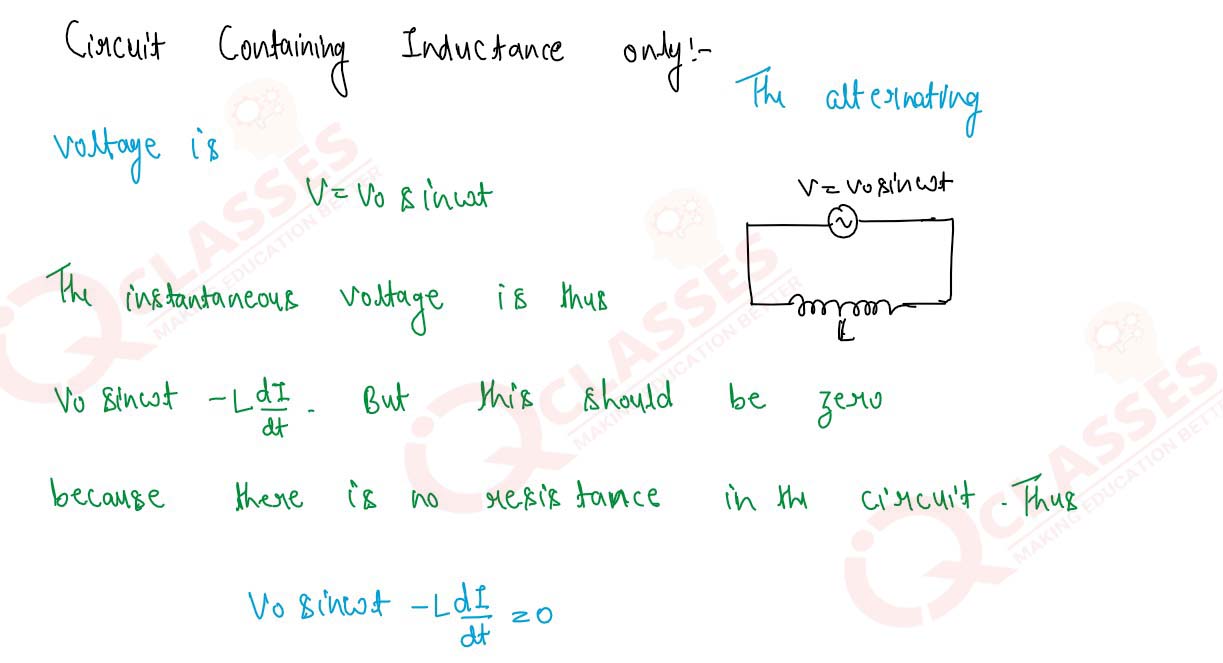
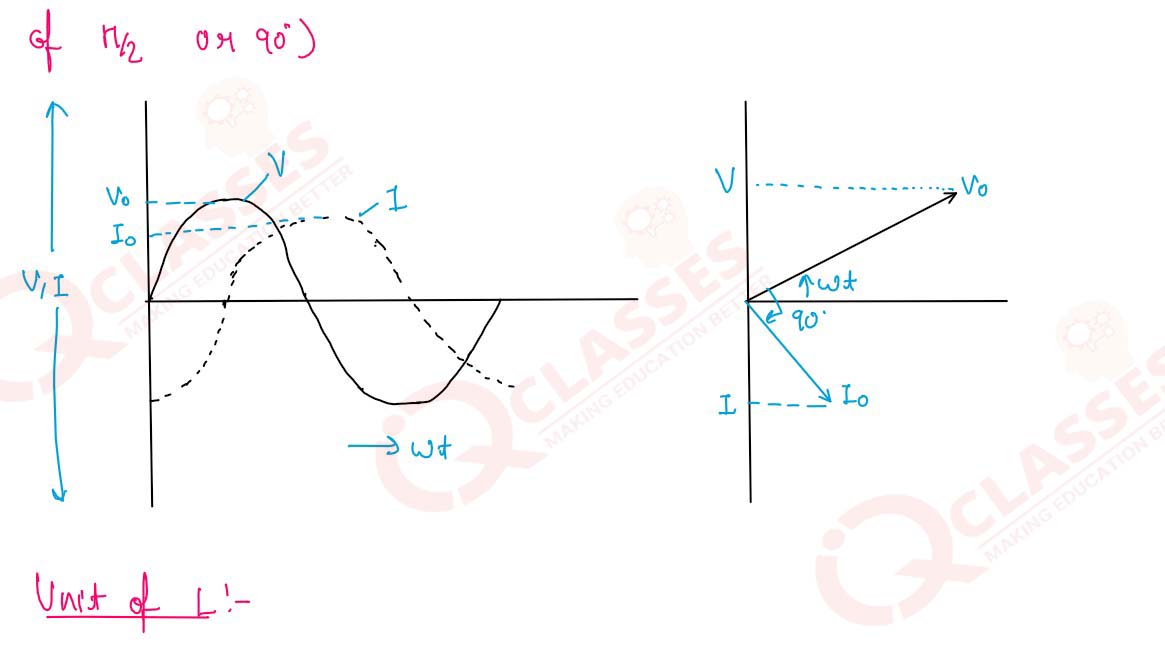
7
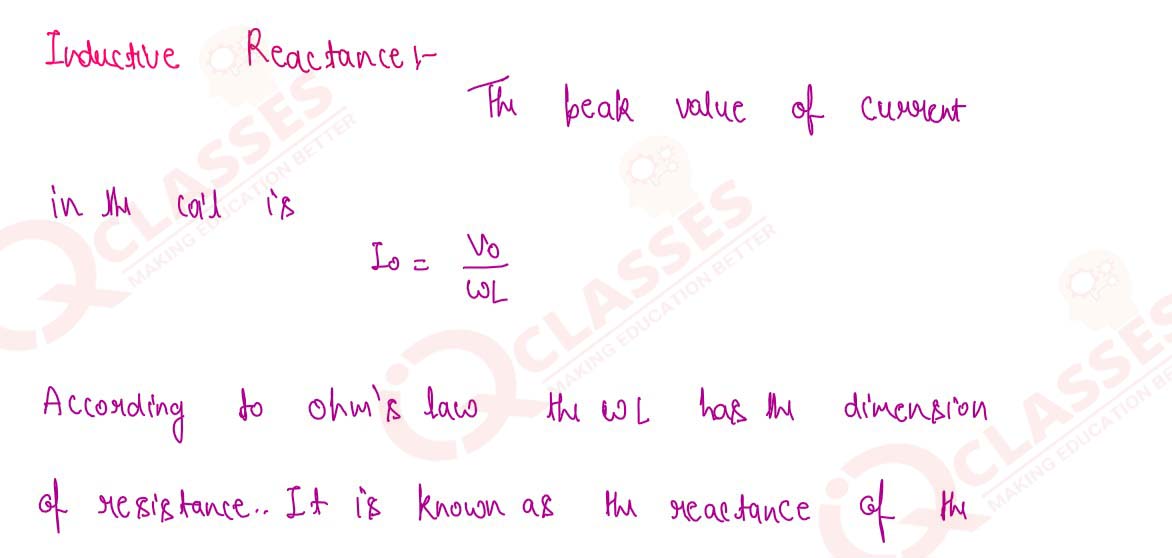
Explain the meaning of ‘reactance’.Derive expression for the reactance of an inductor L connected across
an AC source. Give its unit. Show that no power is dissipated in an inductor when AC passes through it.
Solution



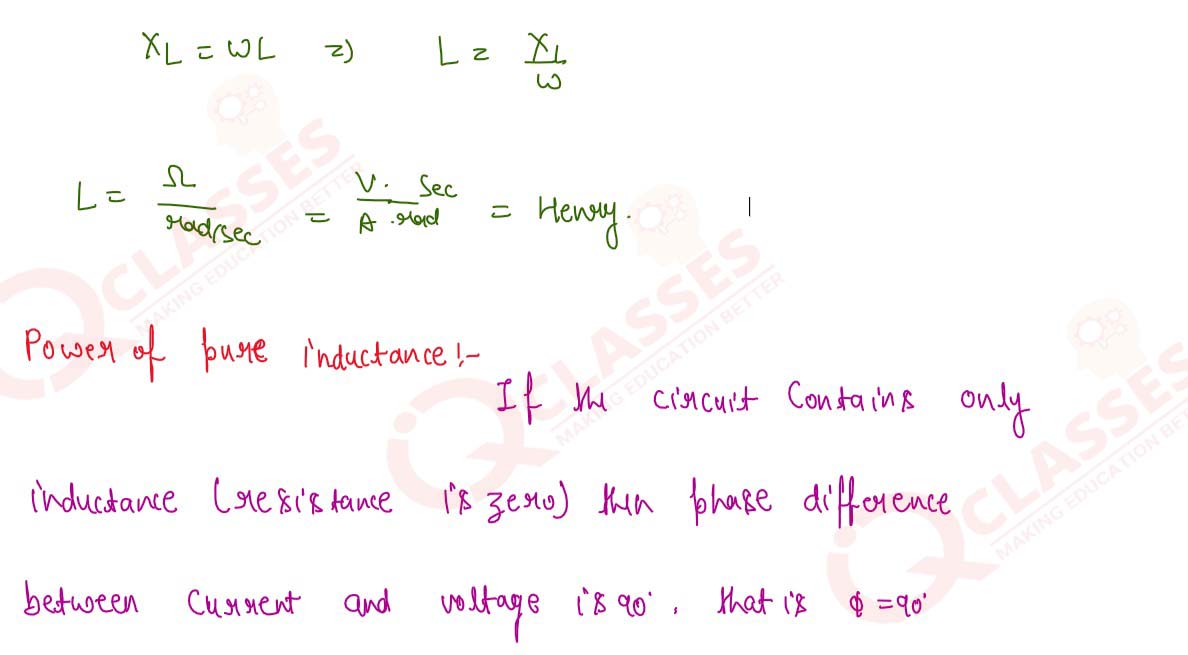






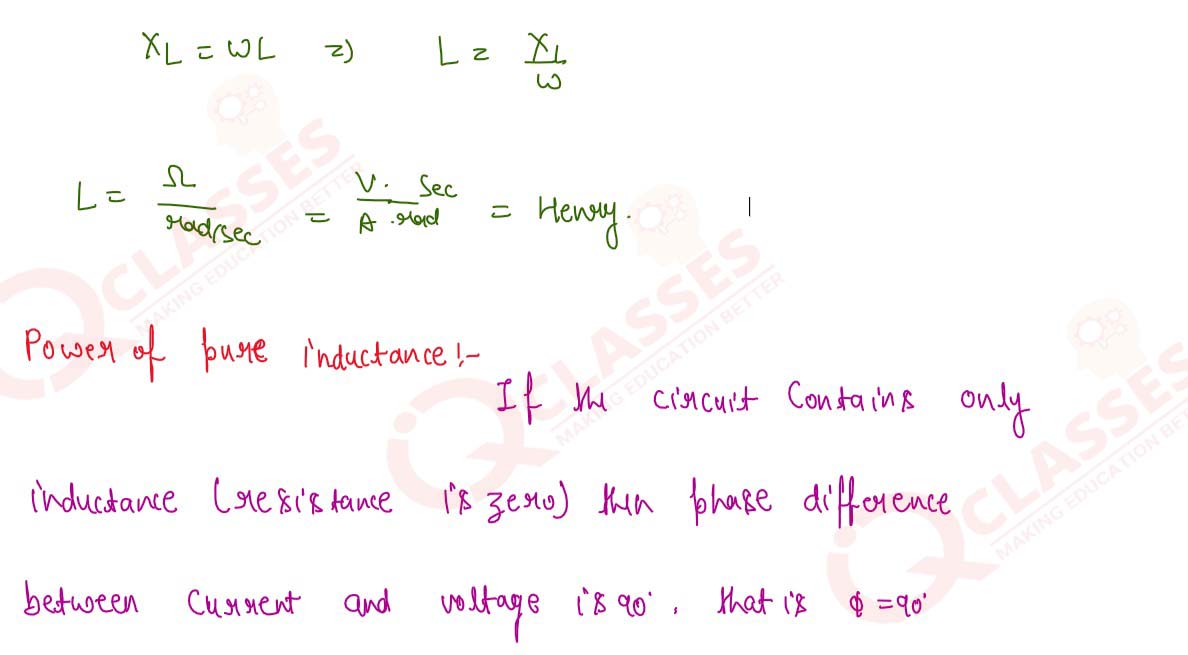

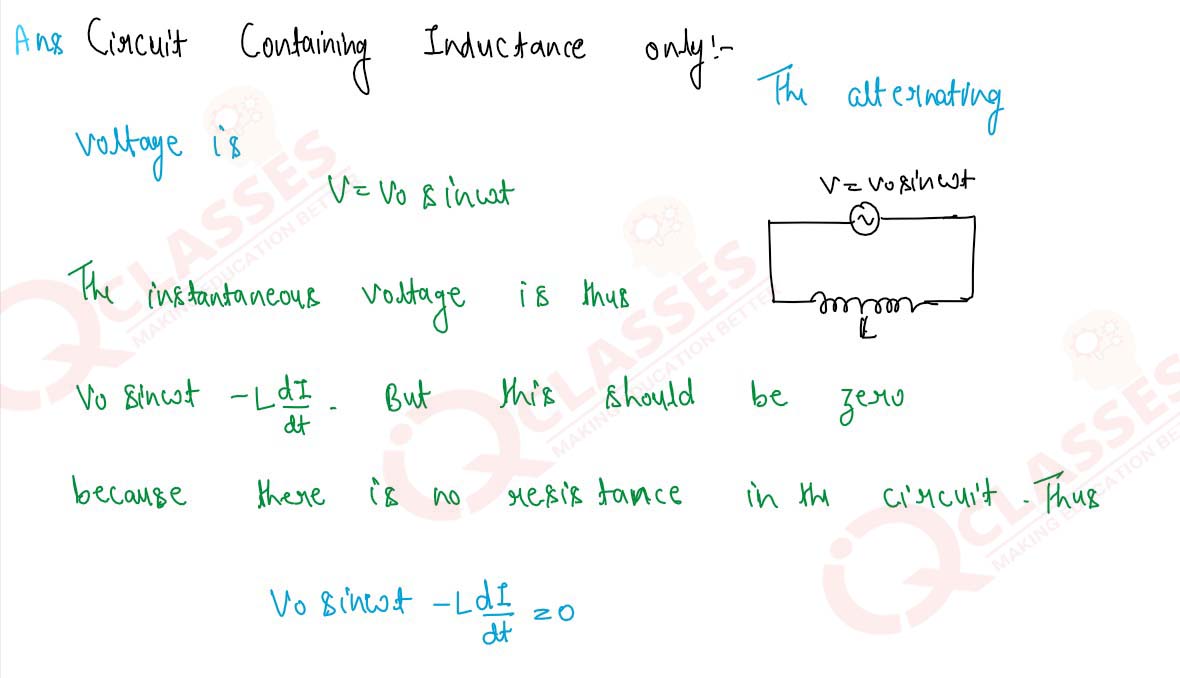
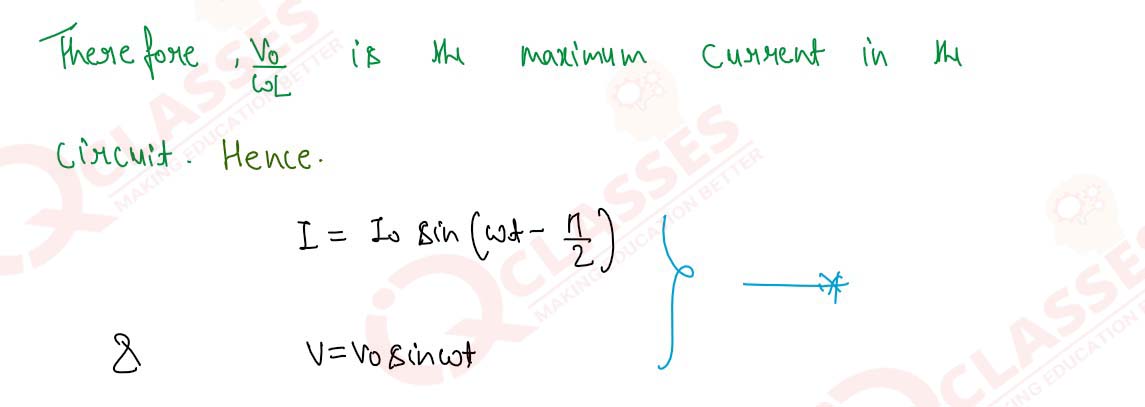
8
Obtain the relation I=I0 sin (wt + π/2) for a pure inductor across which an
alternating
emf V=Vo sin wt is applied.
Solution
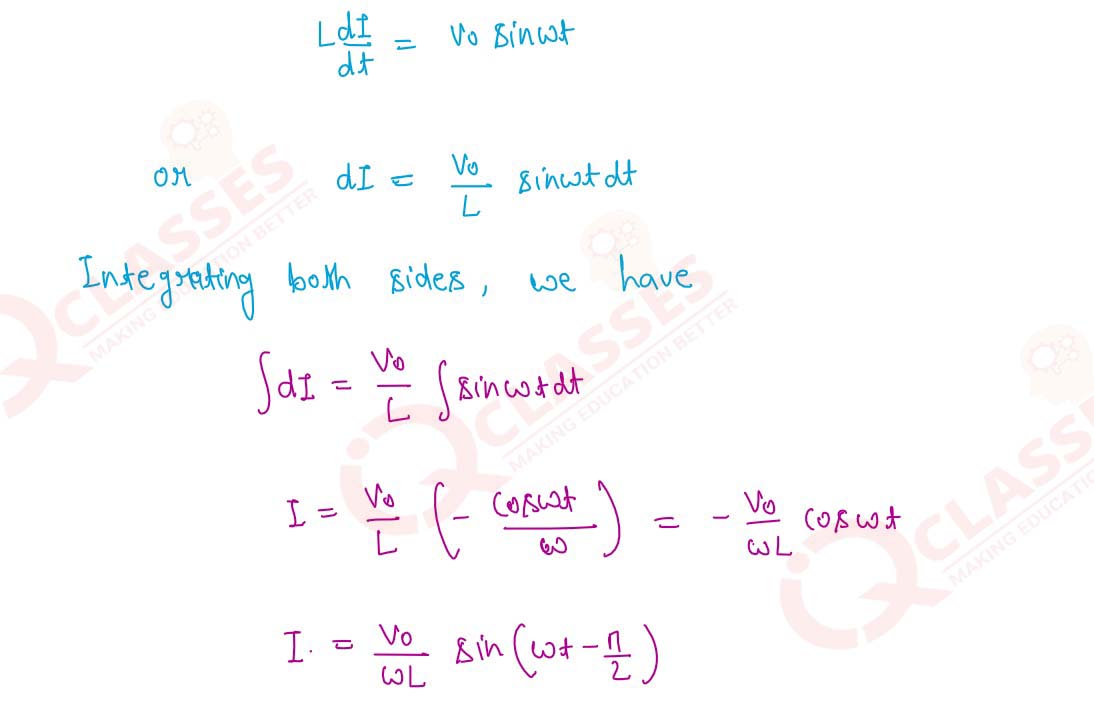

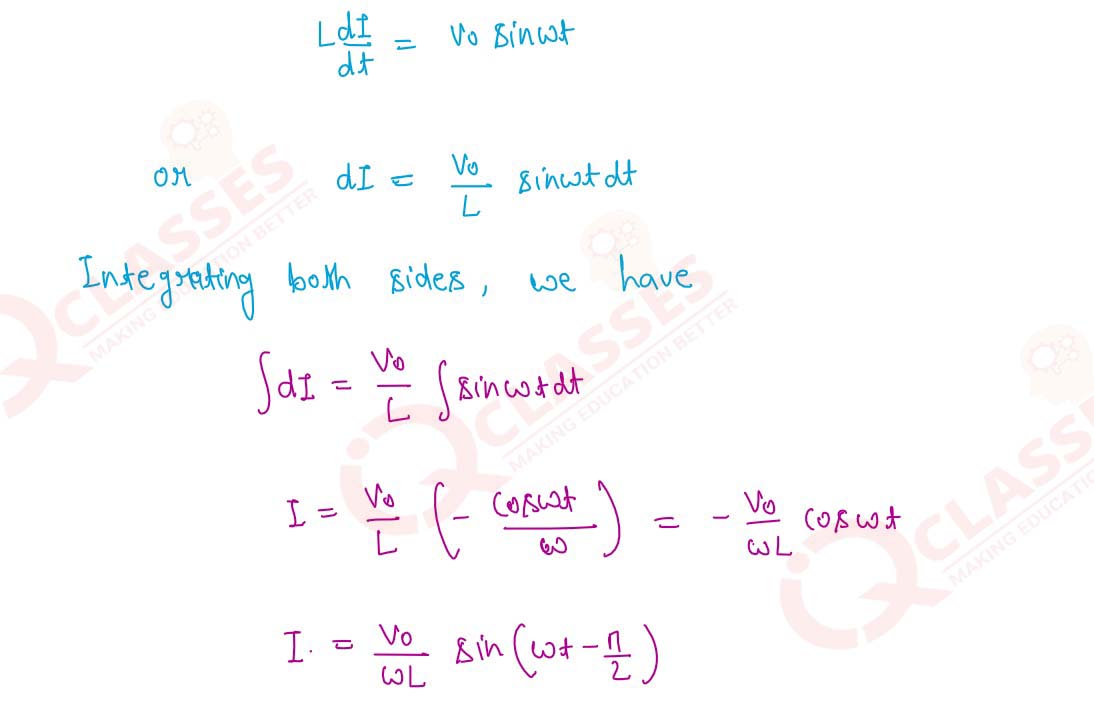

9
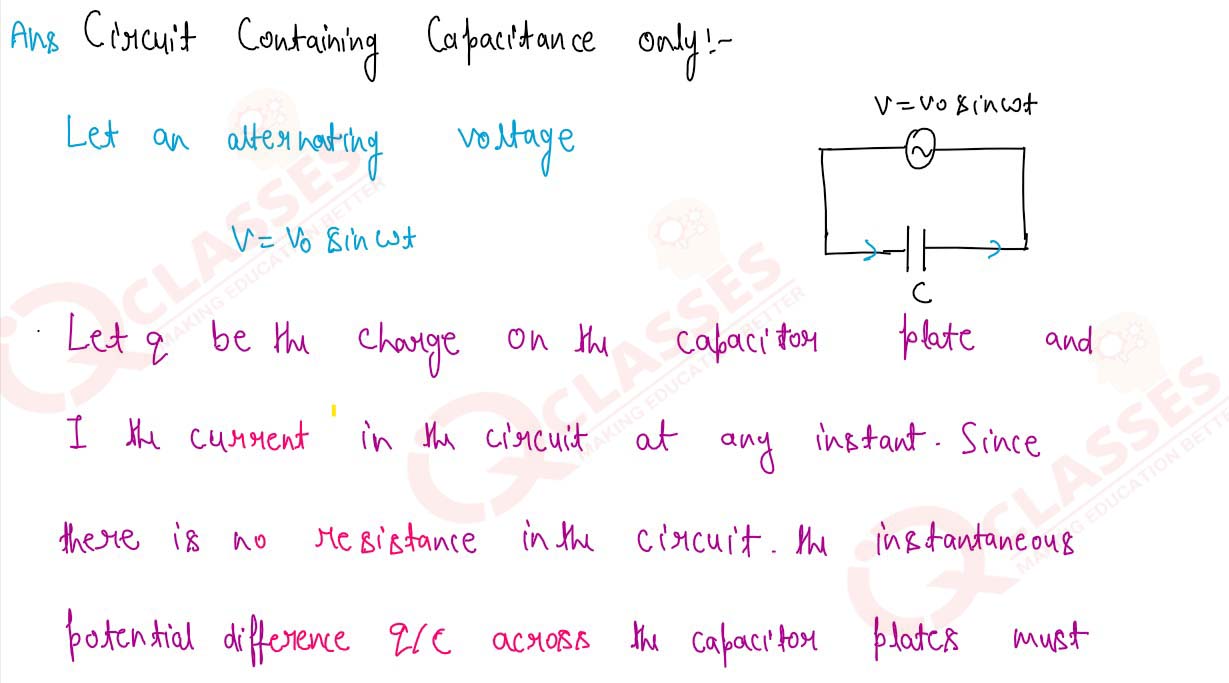
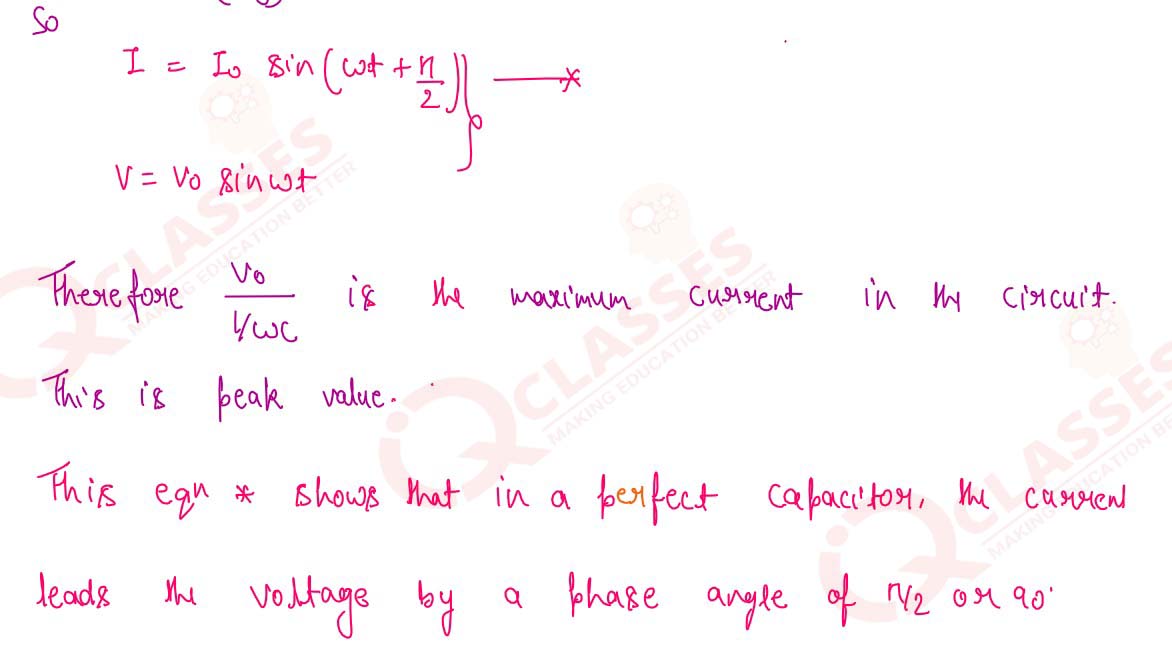
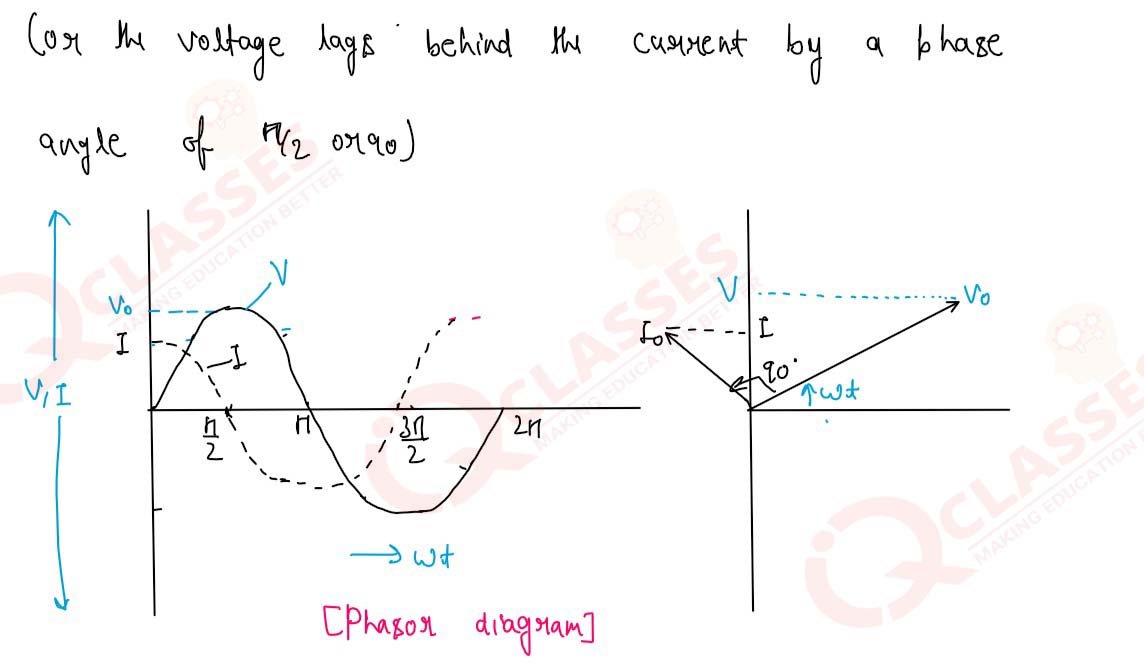
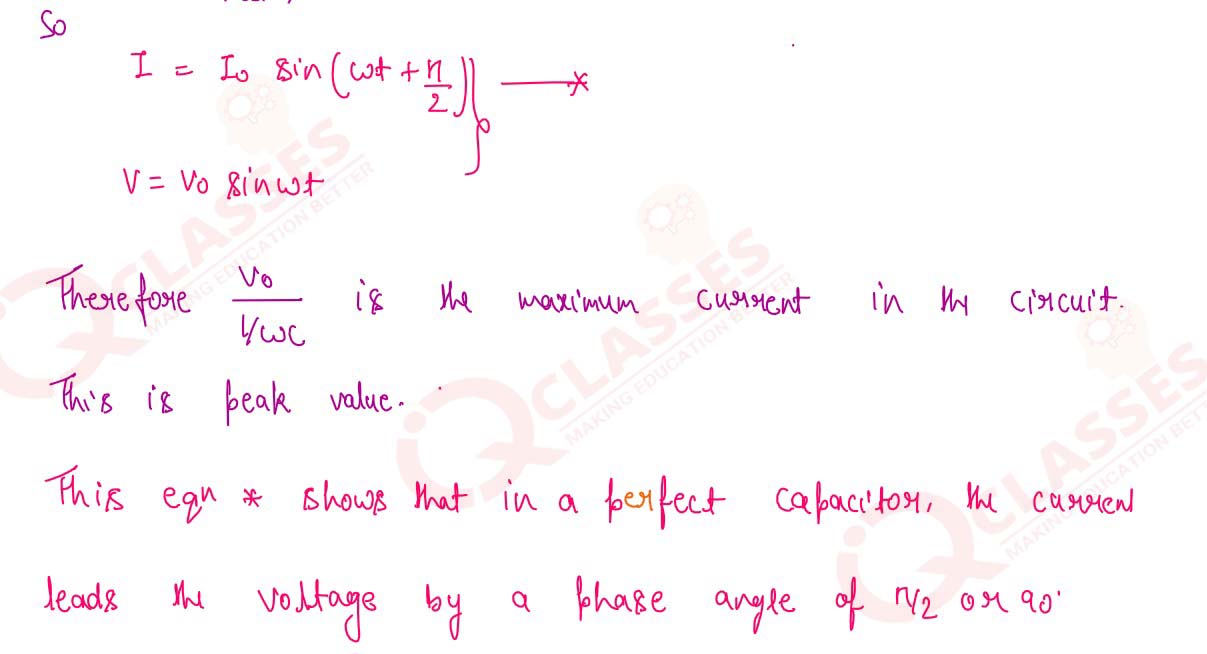
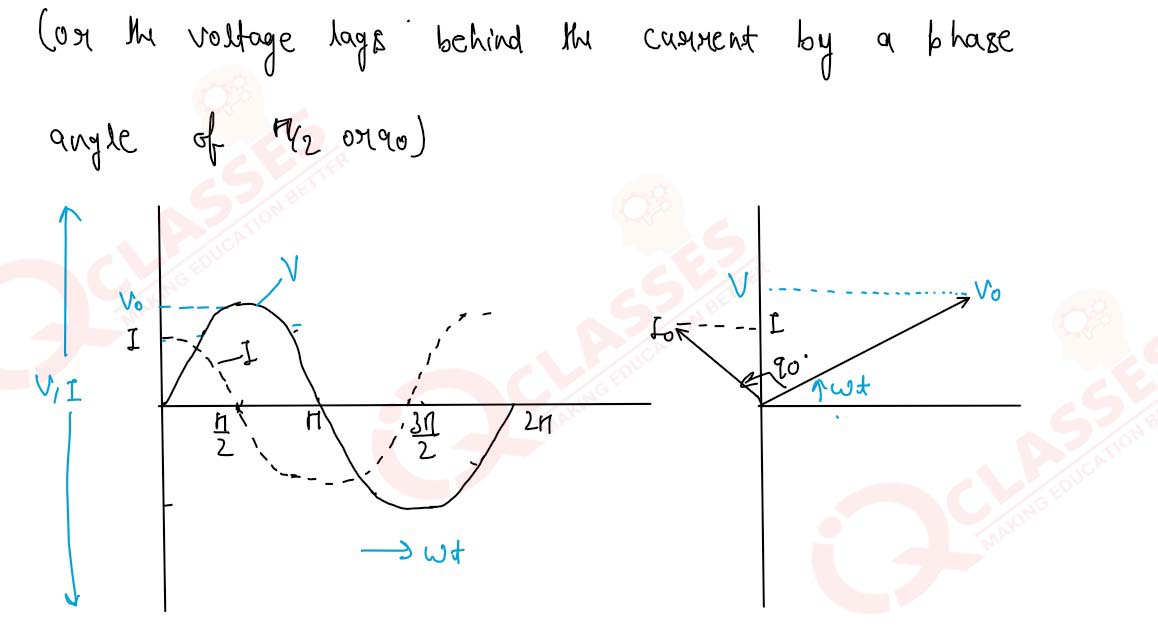
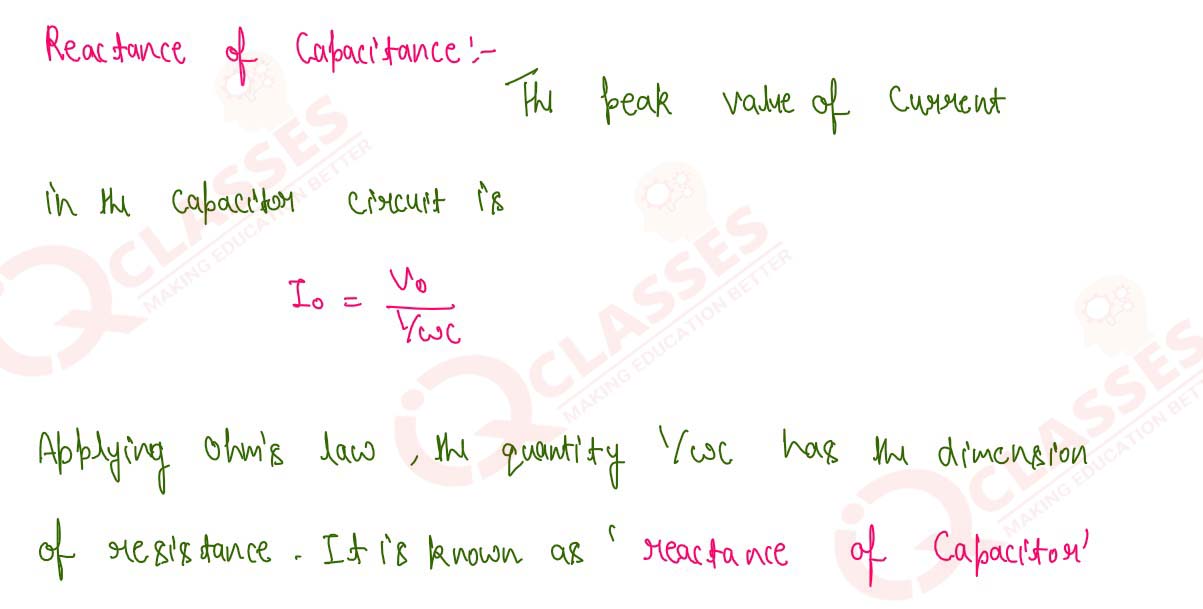
Obtain the relation I=Io sin (wt + π/2) and Xc=1/wC for a pure capacitor across
which an
alternating
emf V=Vo sin wt is applied. Draw a phasor diagram showing emf V, current I and their phase
difference.
Solution





10
A capacitor C is connected to an AC voltage source V=Vo sin wt. Draw a circuit diagram for
it. Obtain an
expression for the current I flowing in the circuit. Express it as a sin function. What is the phase
difference between current I and voltage V? What is the reactance of the capacitor?
Solution

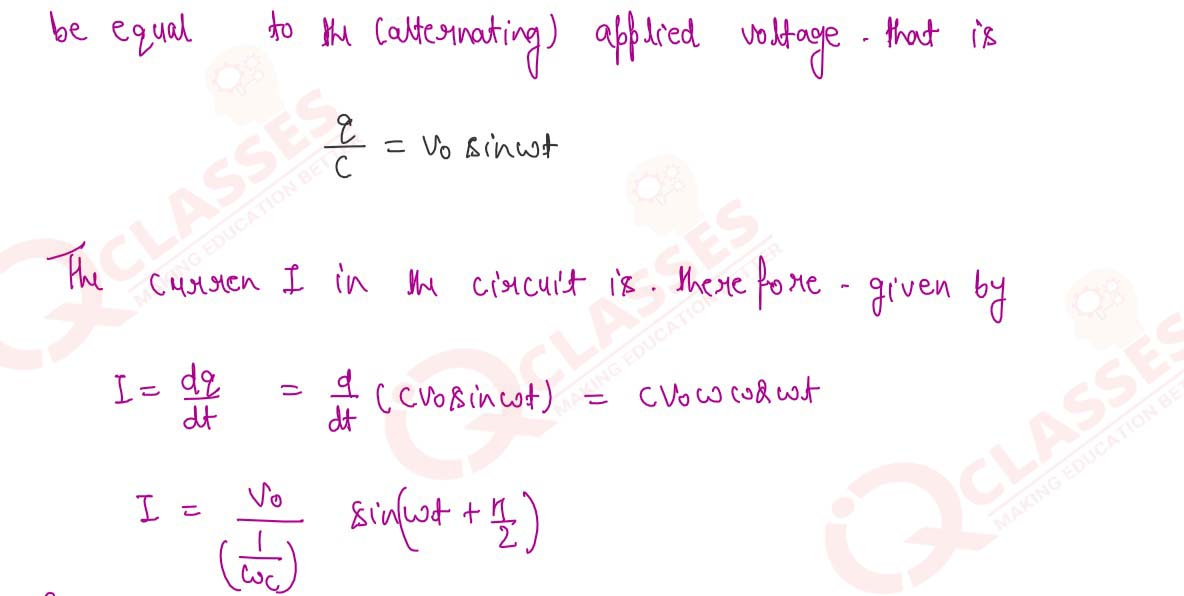
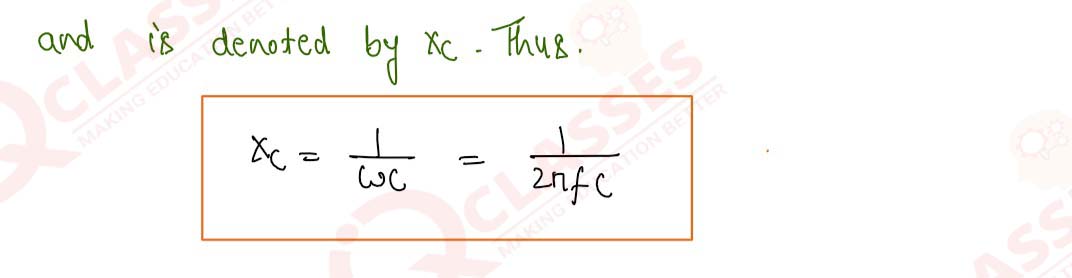

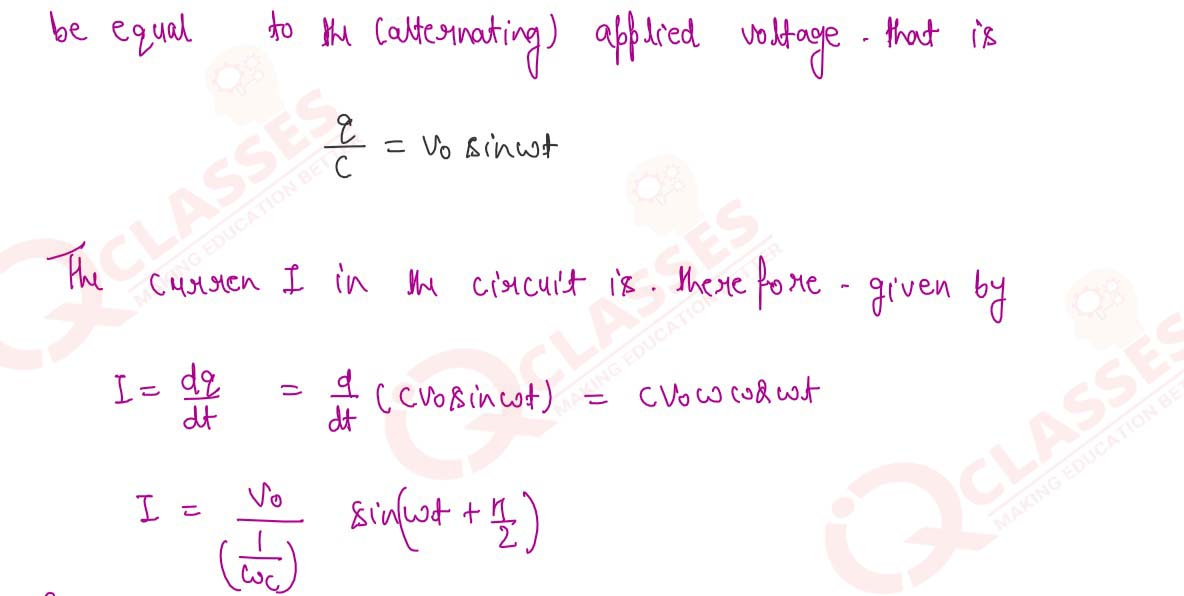



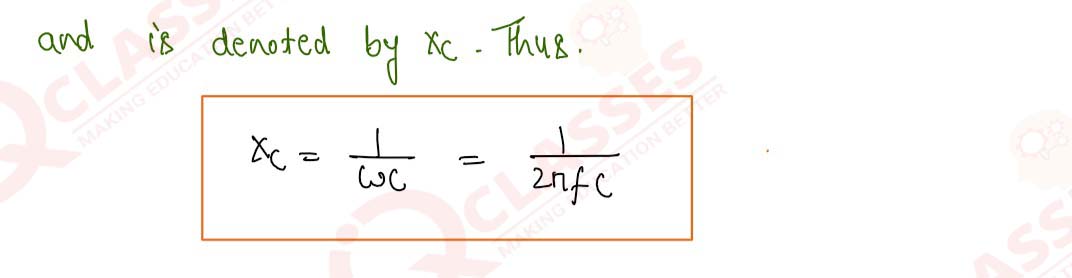
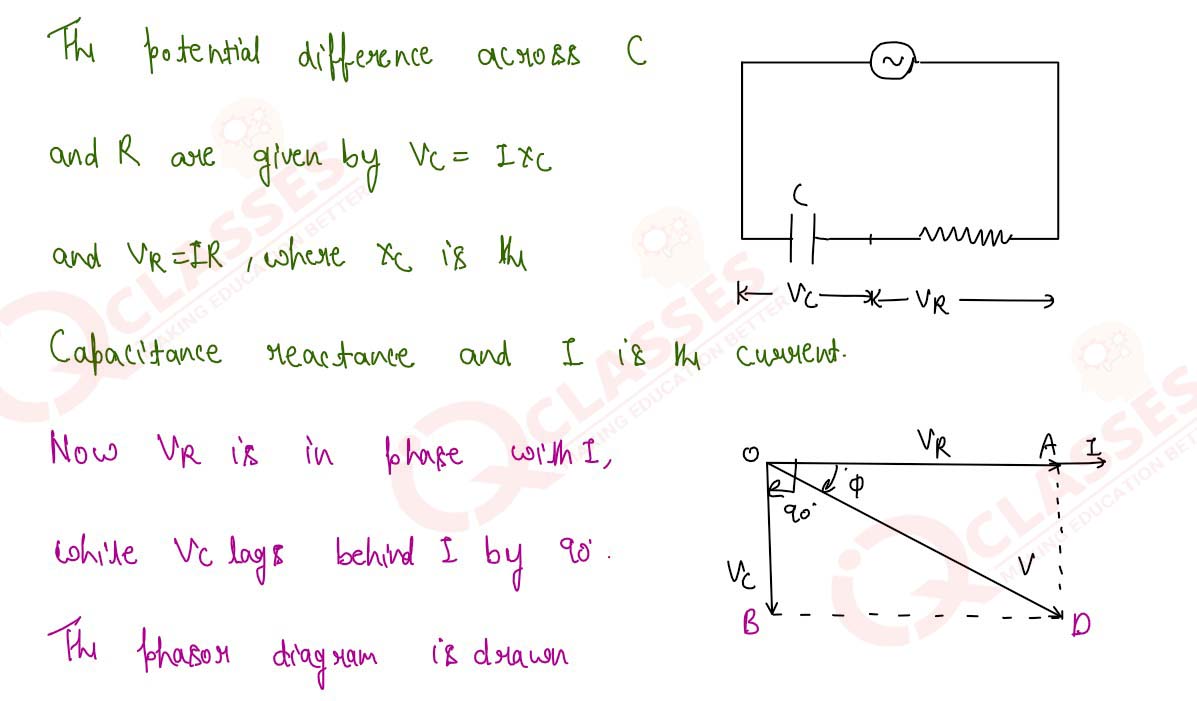
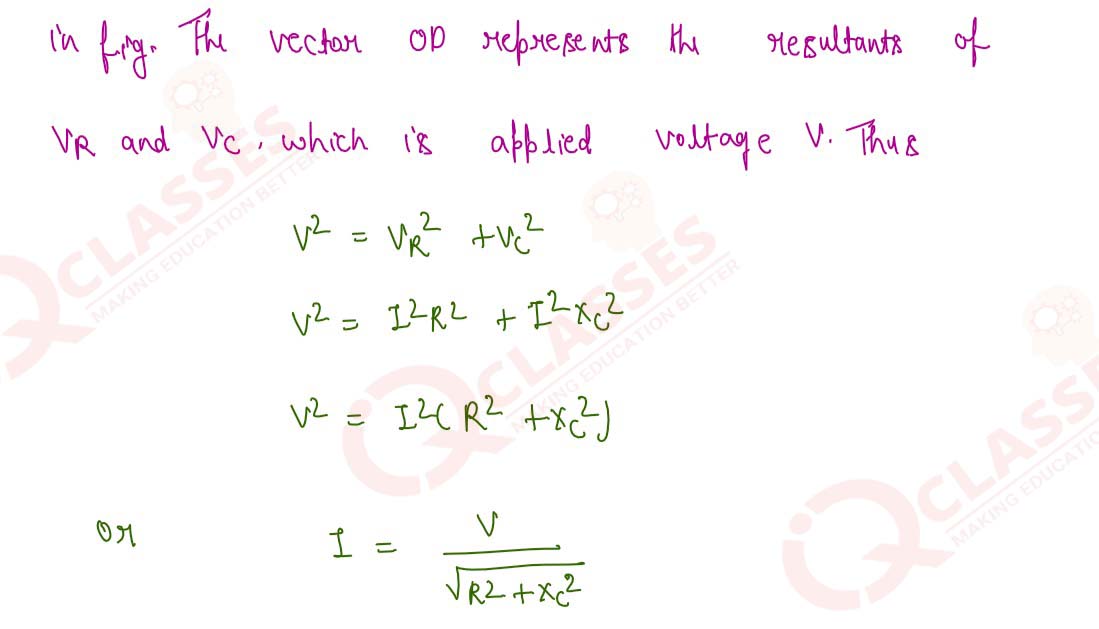
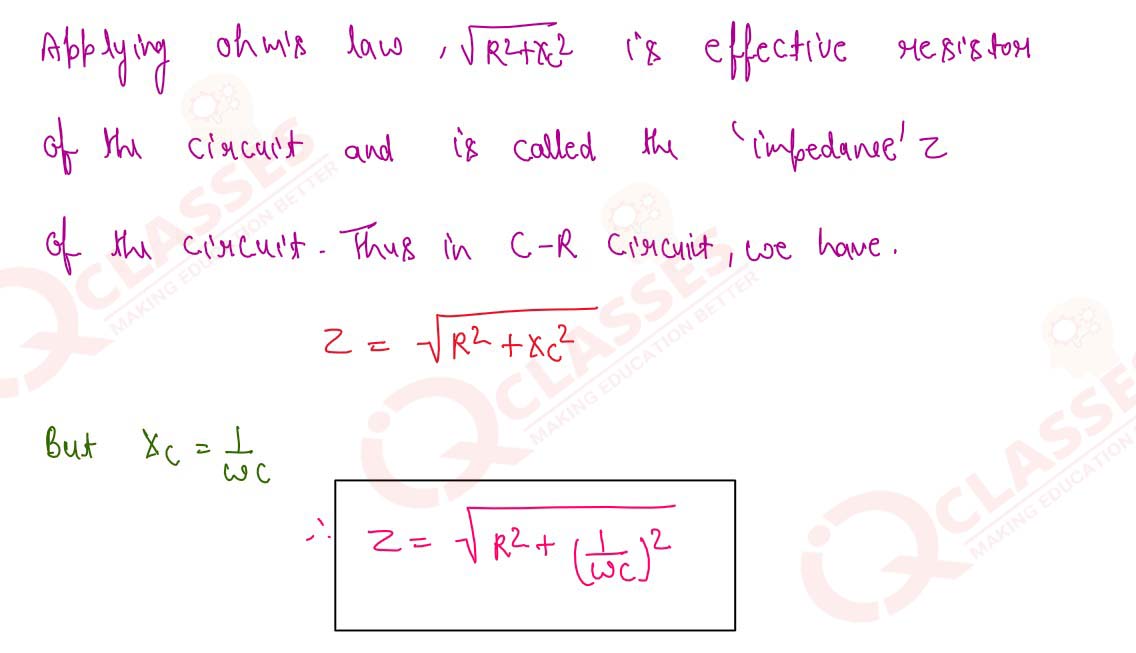
11
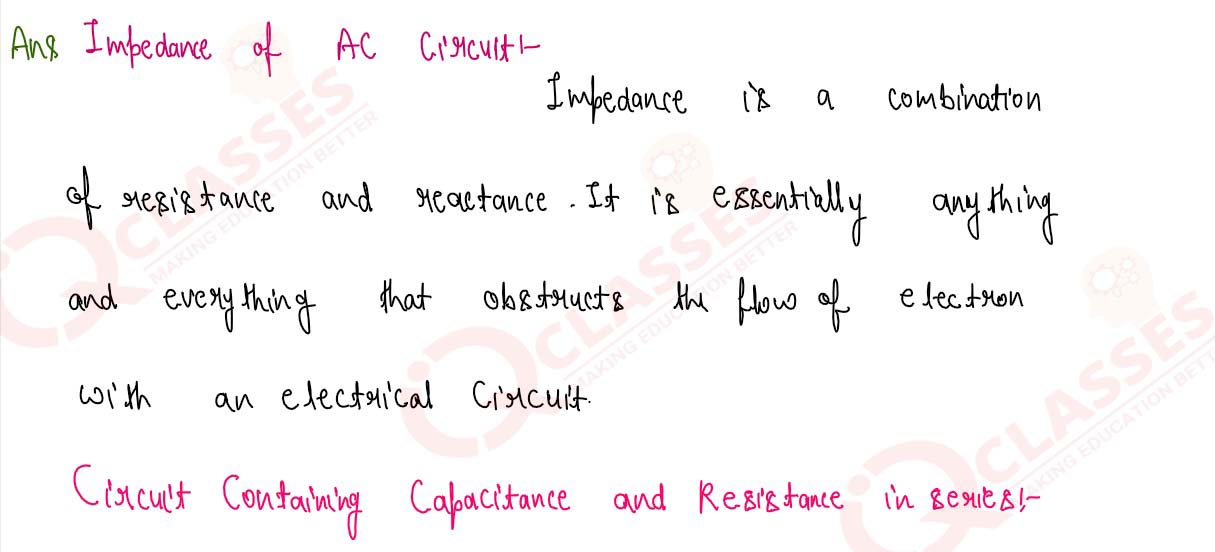
What is impedance of an AC circuit? A capacitor C and a resistor R are connected in series in an AC
circuit. Derive expression for the impedance Z of the circa Draw phasor diagram.
Solution






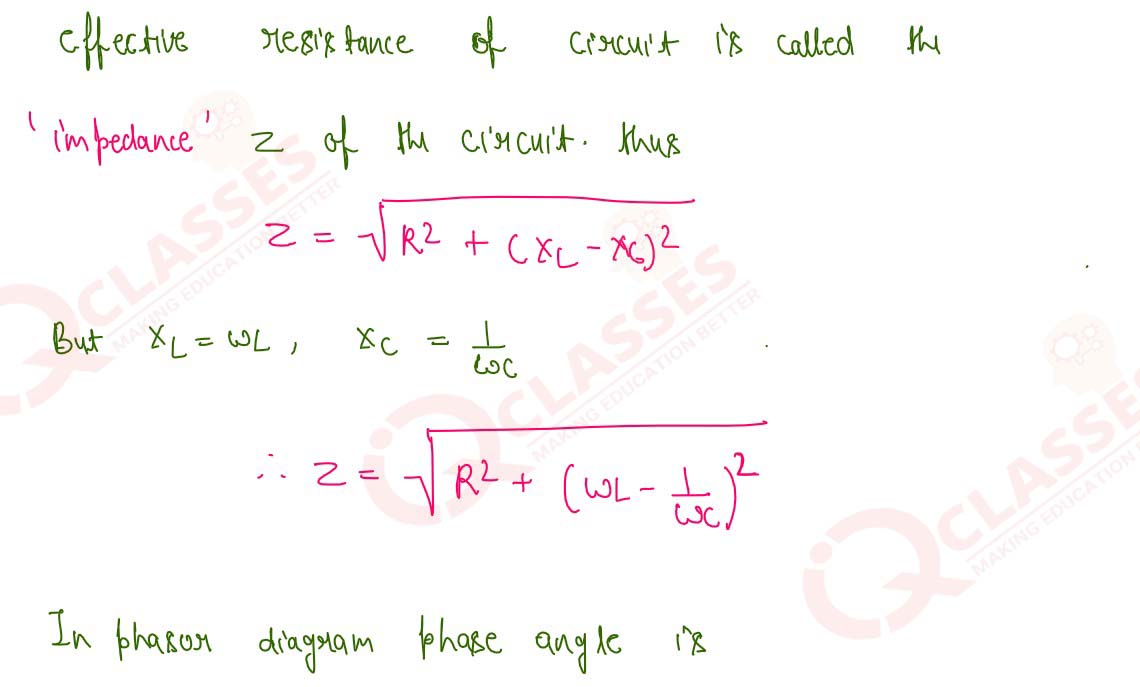
12
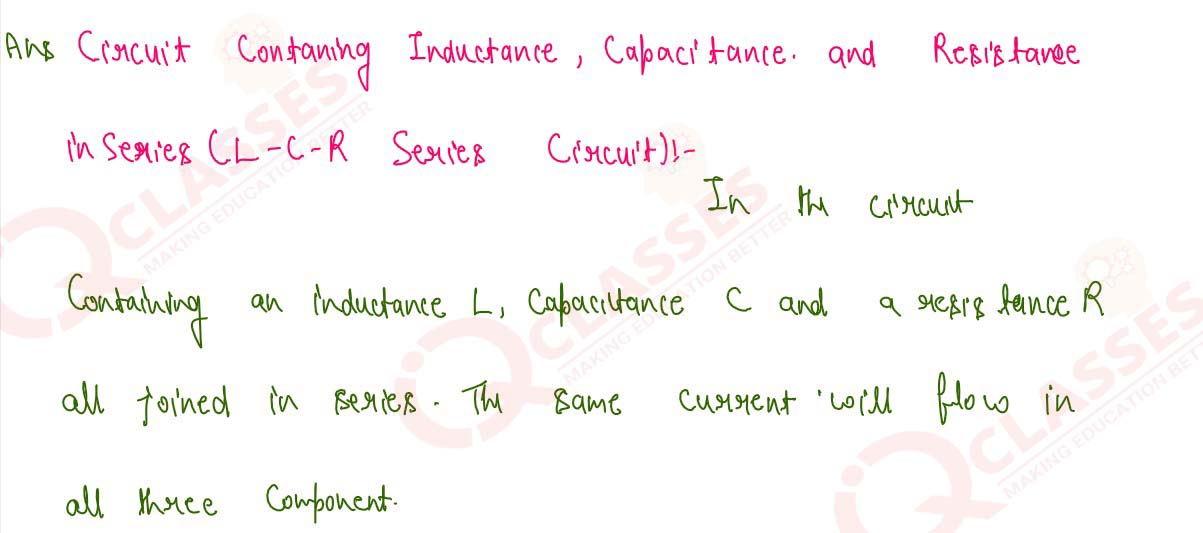
In an alternating-current circuit an inductance L, capacitance C and a resistance R are connected in
series. Derive expression for the impedance and the phase angle. What is the impedance at resonance?
Solution

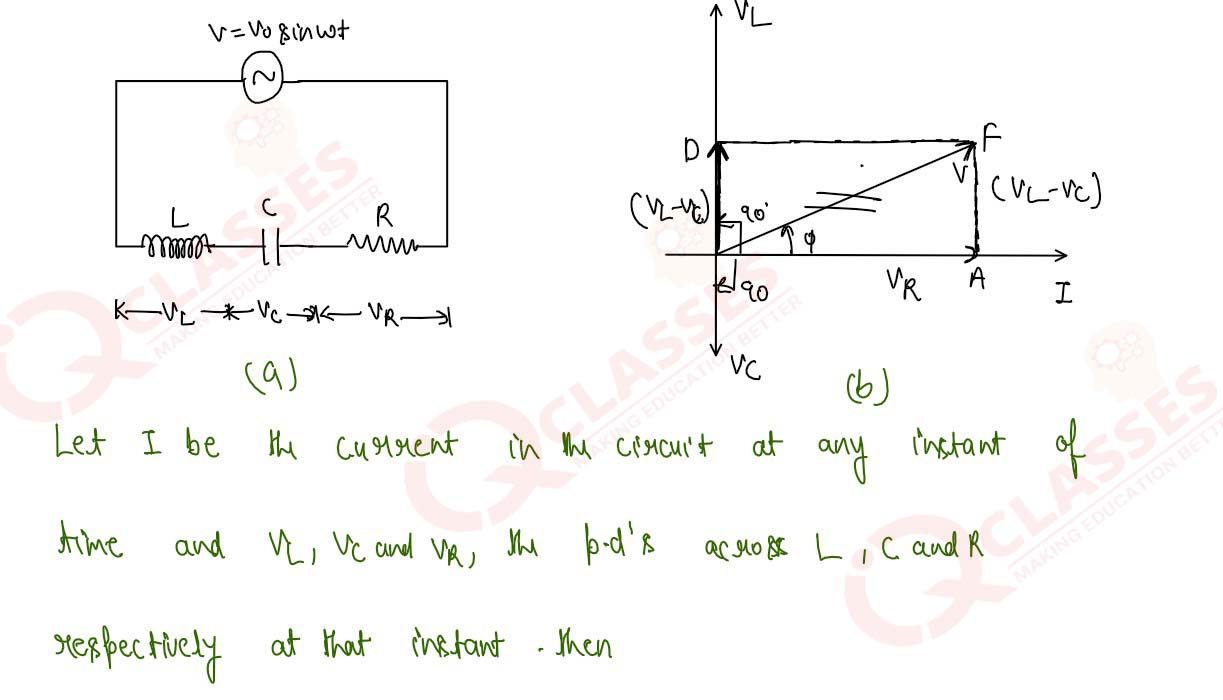









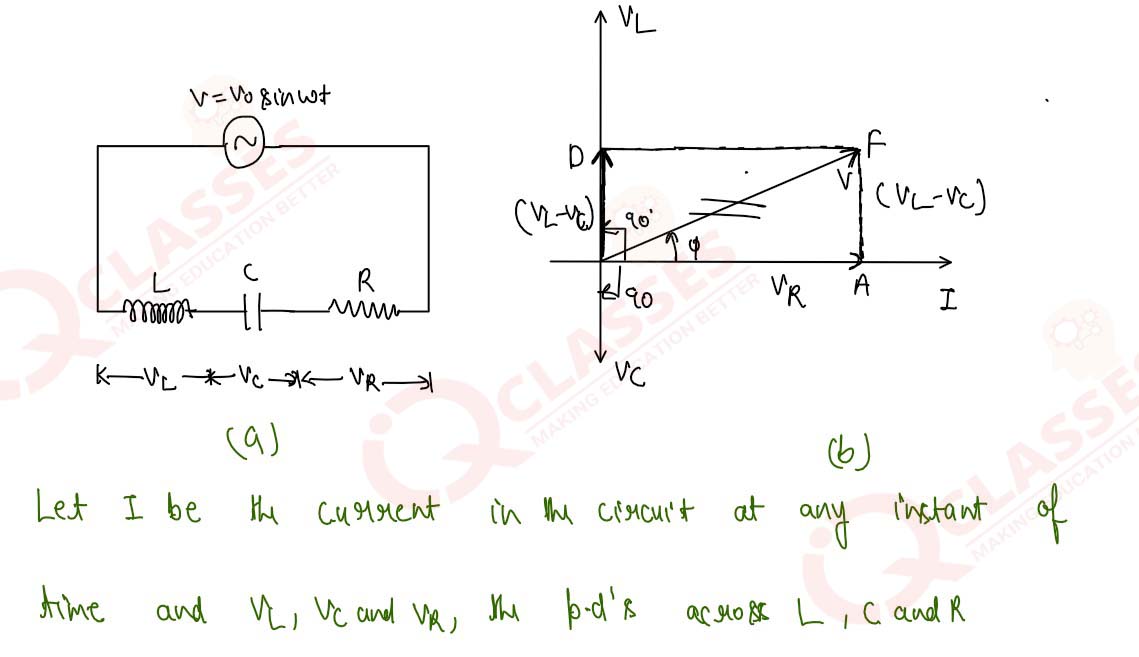
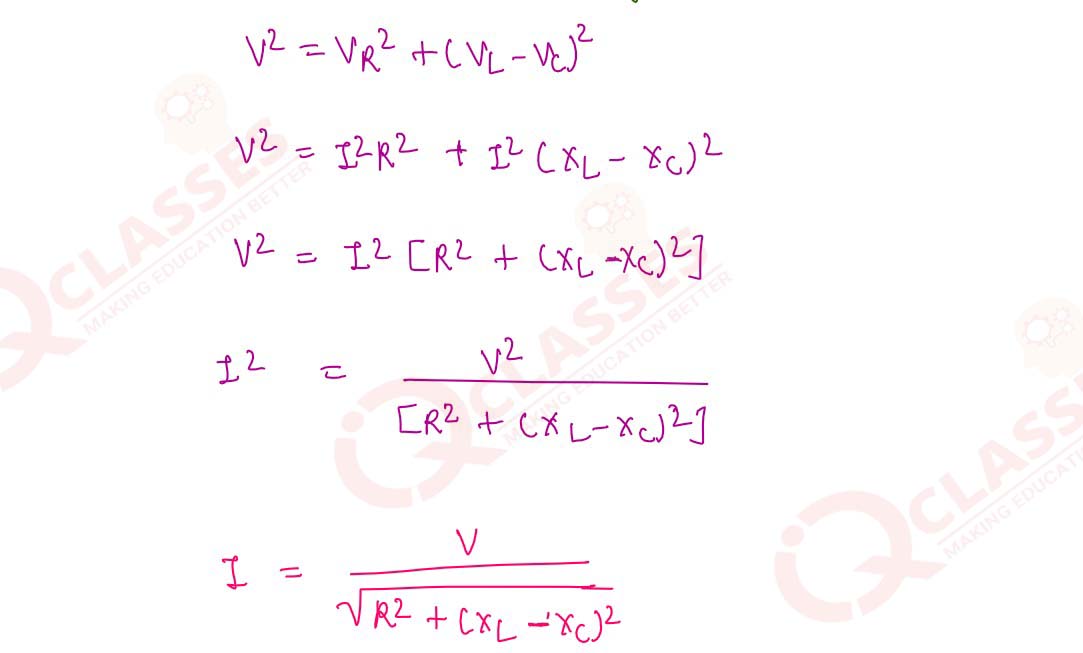
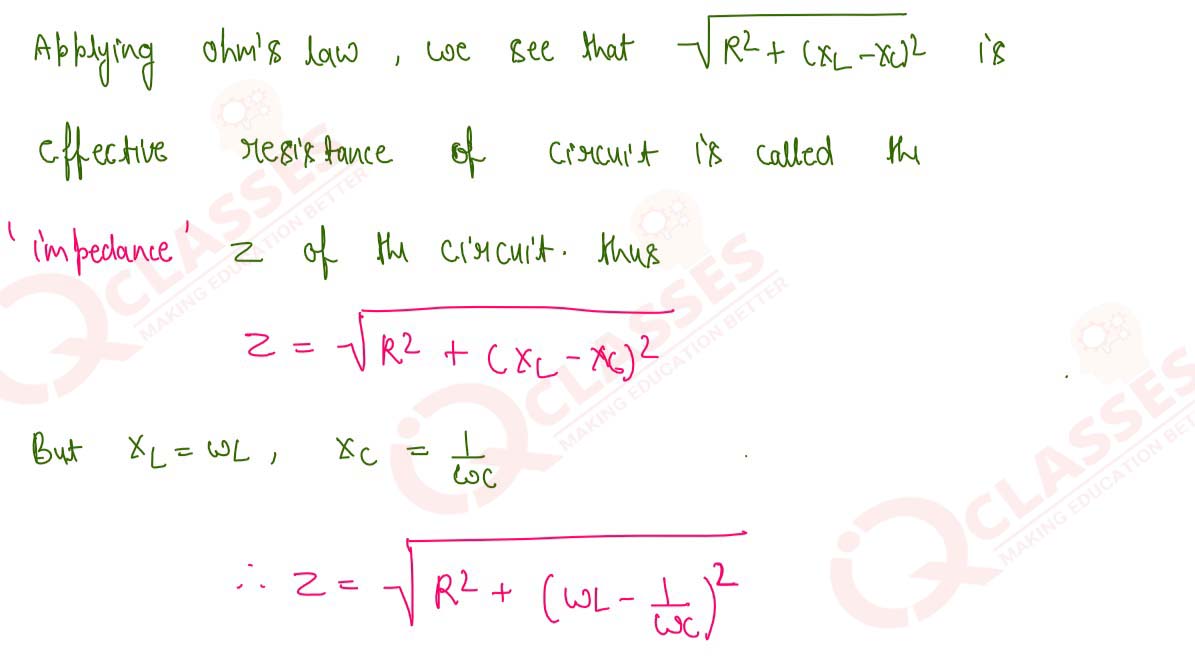
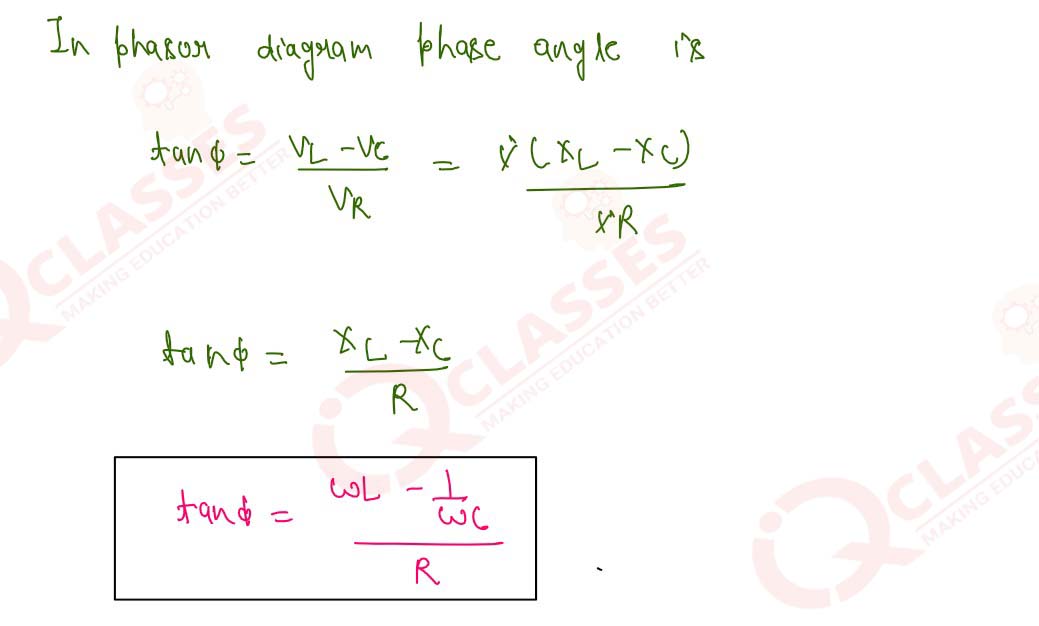
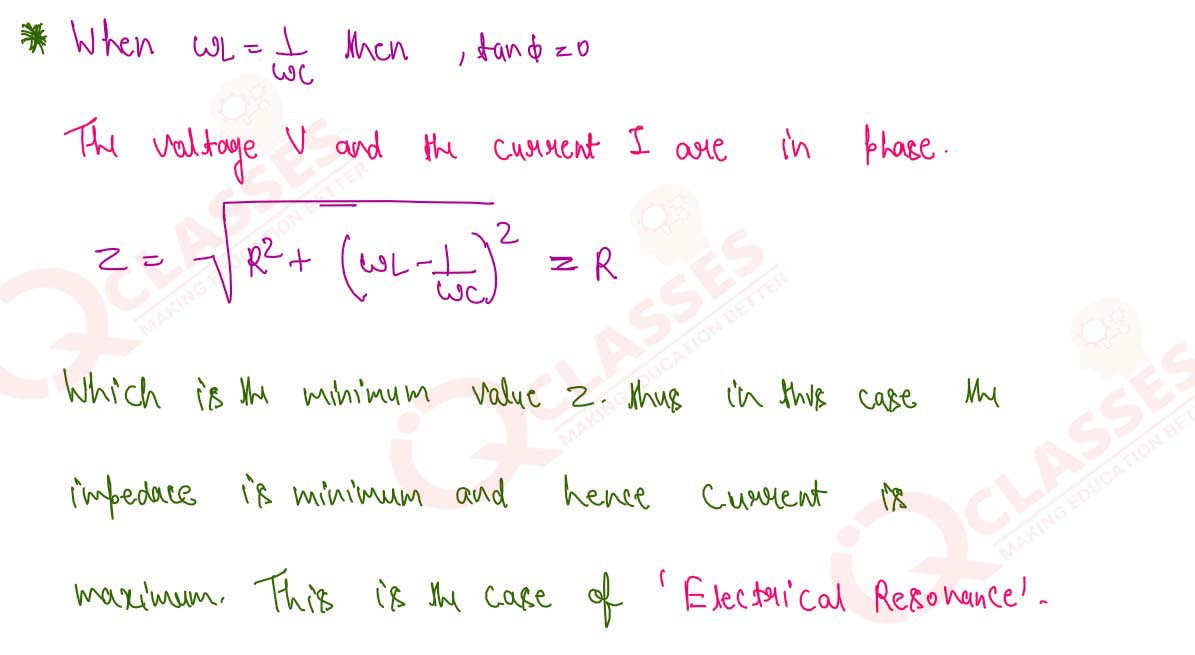
13
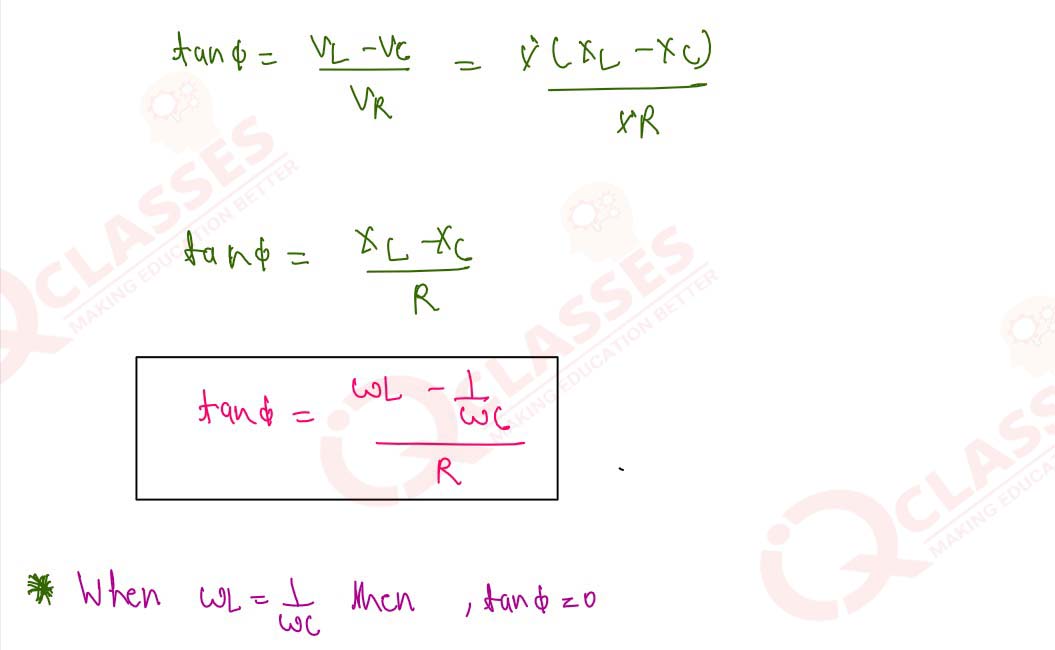
Derive an expression for the phase angle of an AC circuit with an inductor L, a capacitor C and a
resistor R in series. Draw the phasor diagram if the voltage across the capacitor is greater than that
across the inductor, Obtain an expression for the resonat frequency of the circuit.
Solution










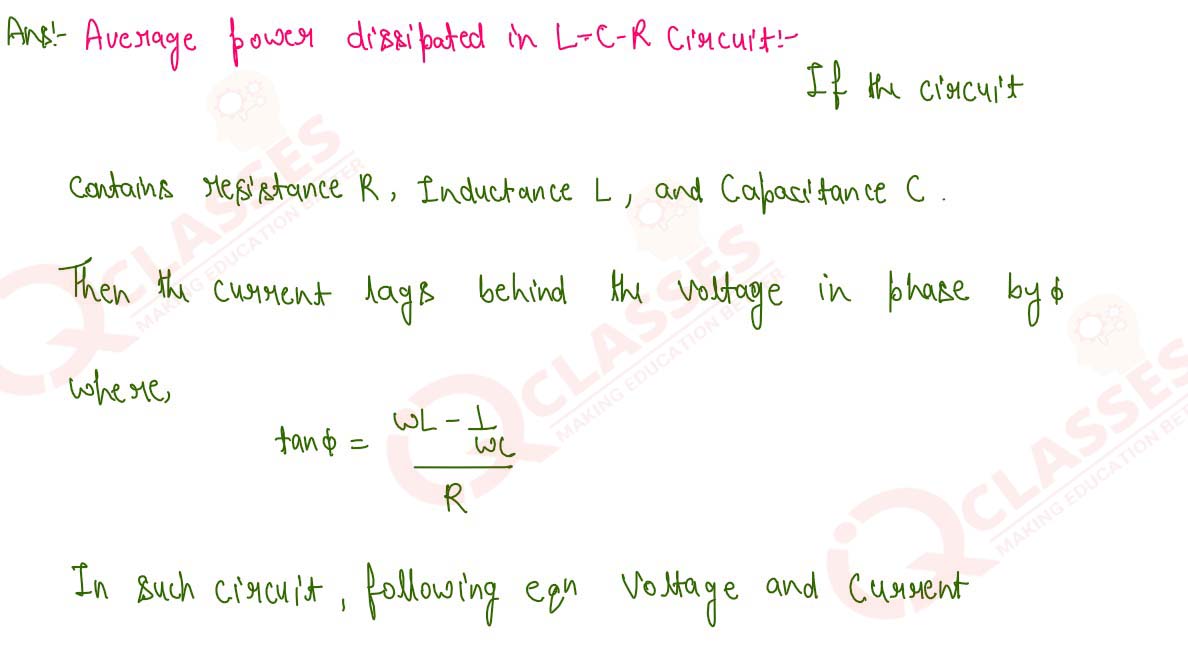
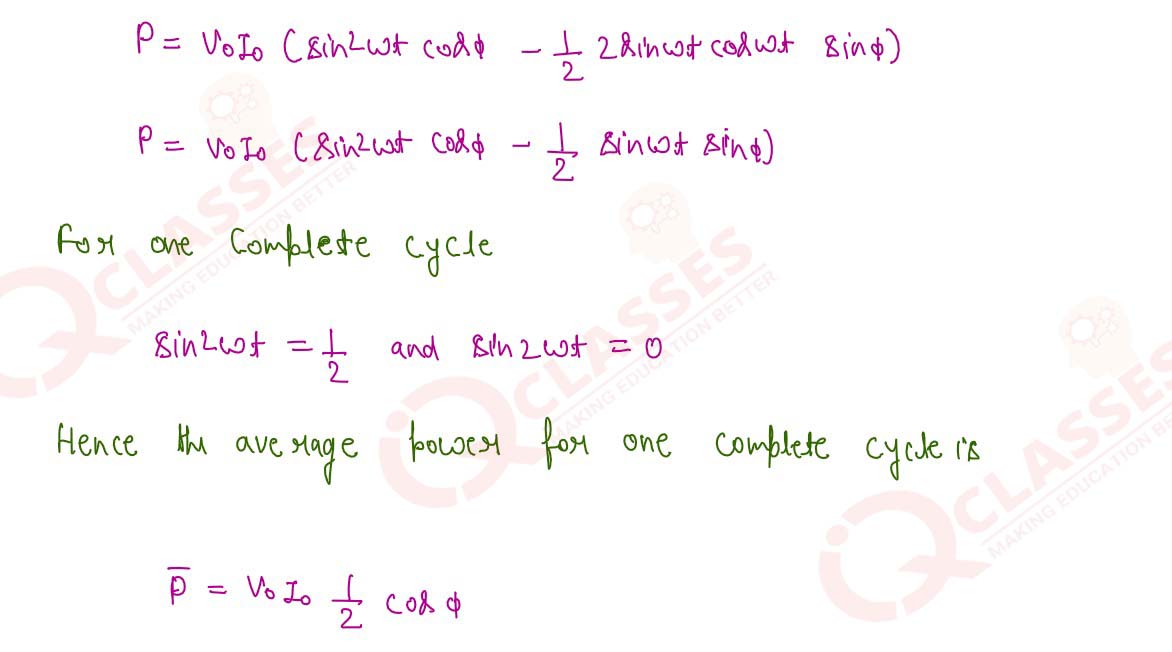
14
Show that the average power dissipated per cycle in an L-C-R circuit is given by P= Vrms
Irms x cos θ, where θ is the angle of lag or lead.
Solution

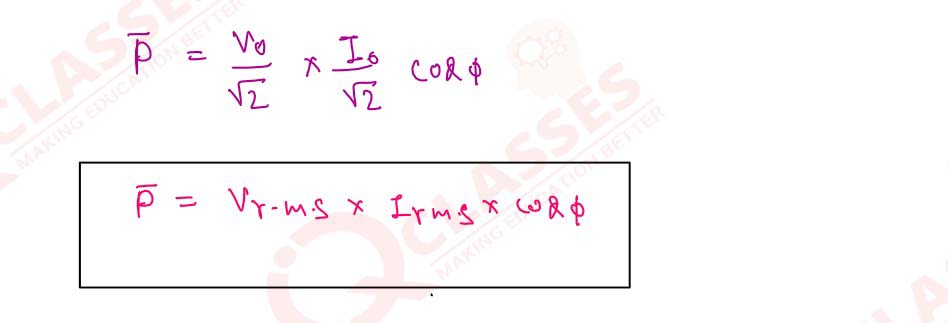



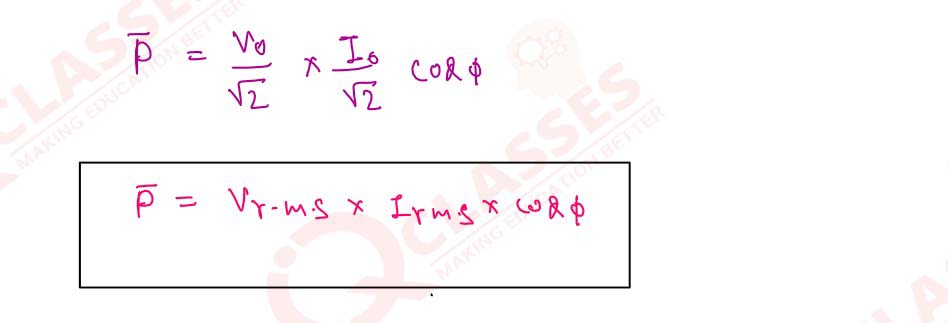
15
Show that the average power dissipated in an AC circuit is, in general, given by
P=Vrms xIrms xR/Z where R is the resistance of the circuit (defined as real part of the complex impedance) and Z is the impedance. Solution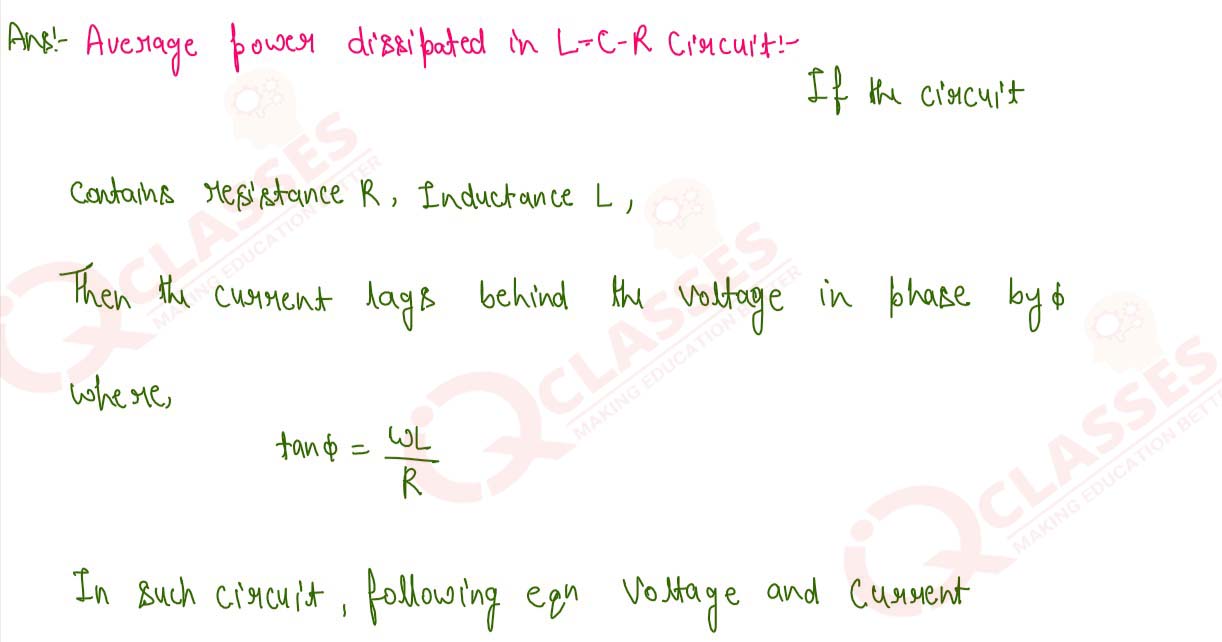

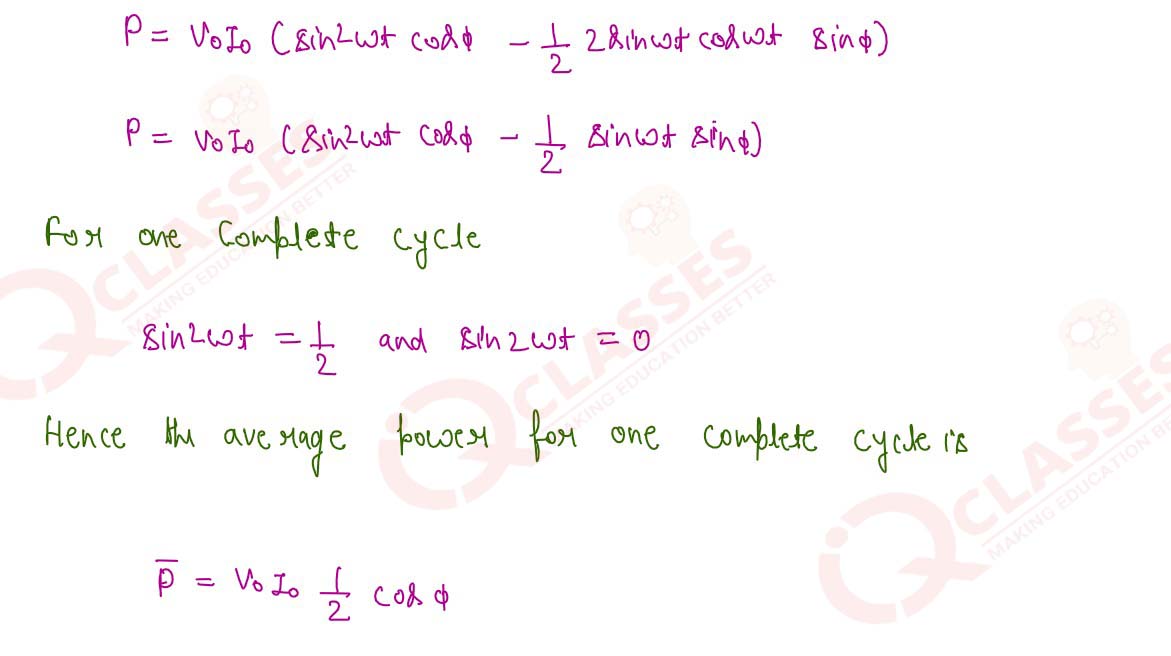
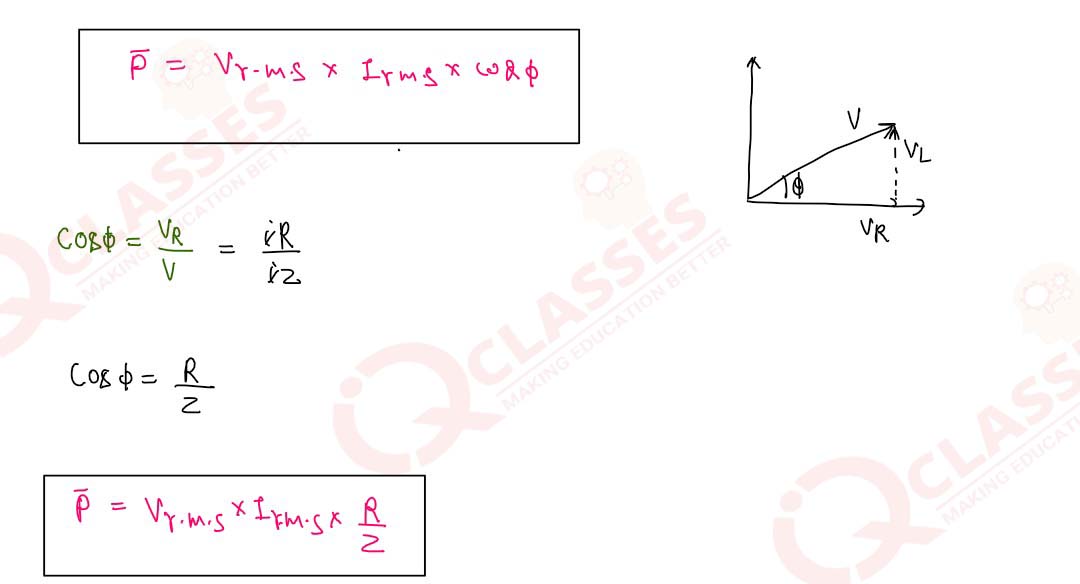
P=Vrms xIrms xR/Z where R is the resistance of the circuit (defined as real part of the complex impedance) and Z is the impedance. Solution




16
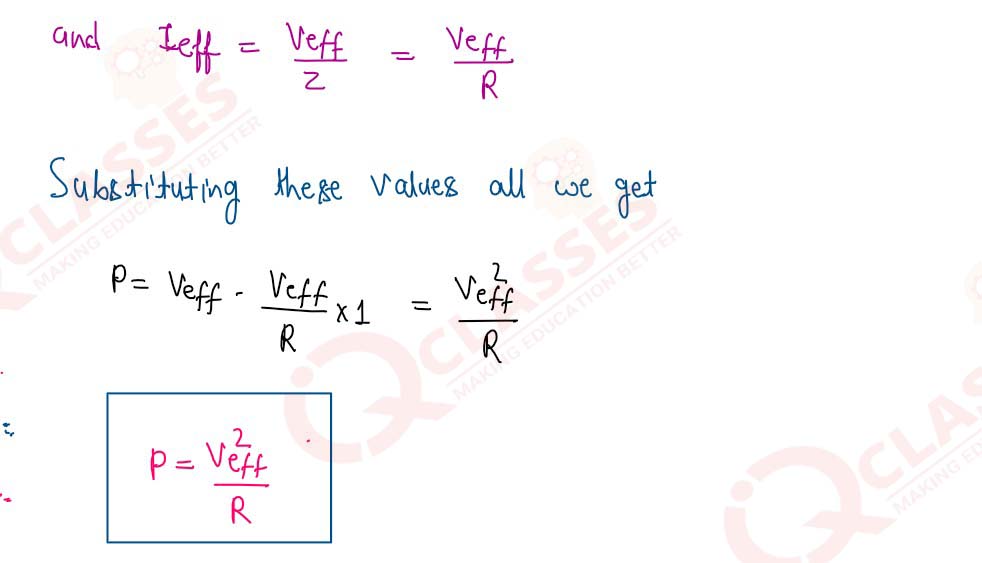
Prove that power dissipated in an ideal resistor connected to an AC source is
Veff2/R.
Solution



17
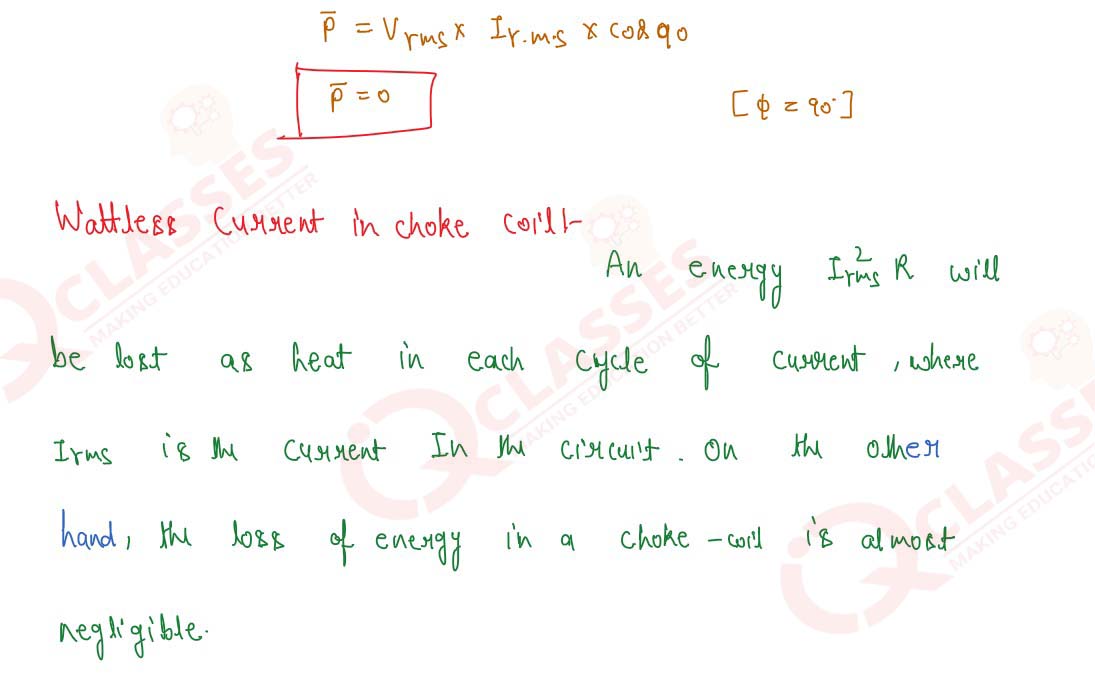
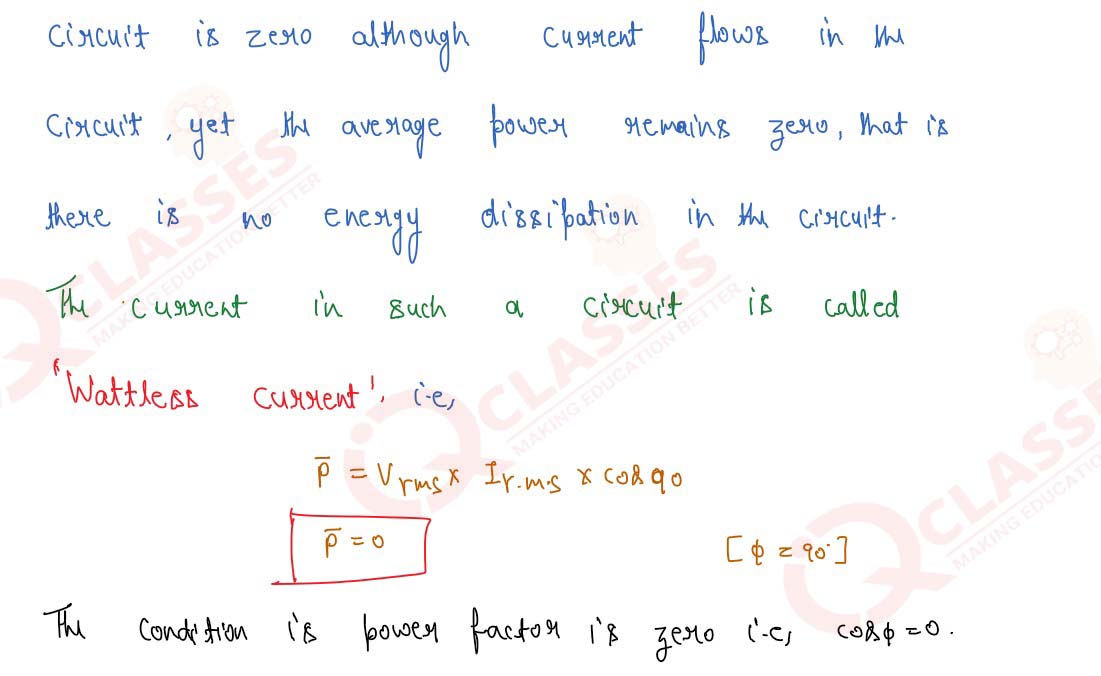
What do you understand by 'wattless current'? Why is
the current passing in a choke coil wattless?
Solution







18
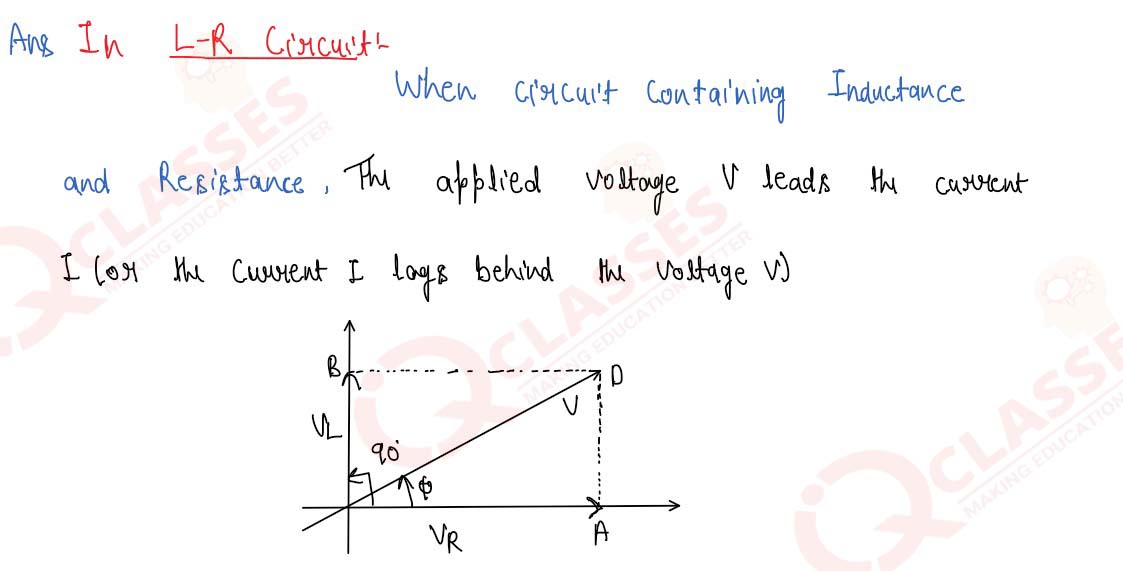
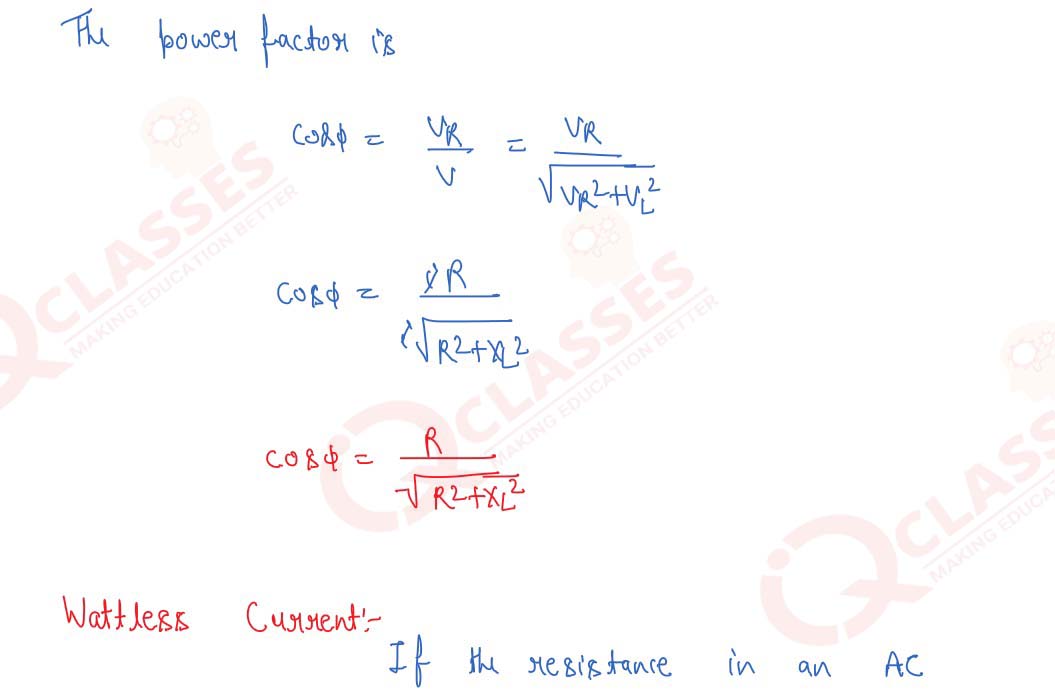
Obtain an expression for the power factor of an AC L-R circuit. Give the necessary condition for a
wattless current in the circuit.
Solution



19
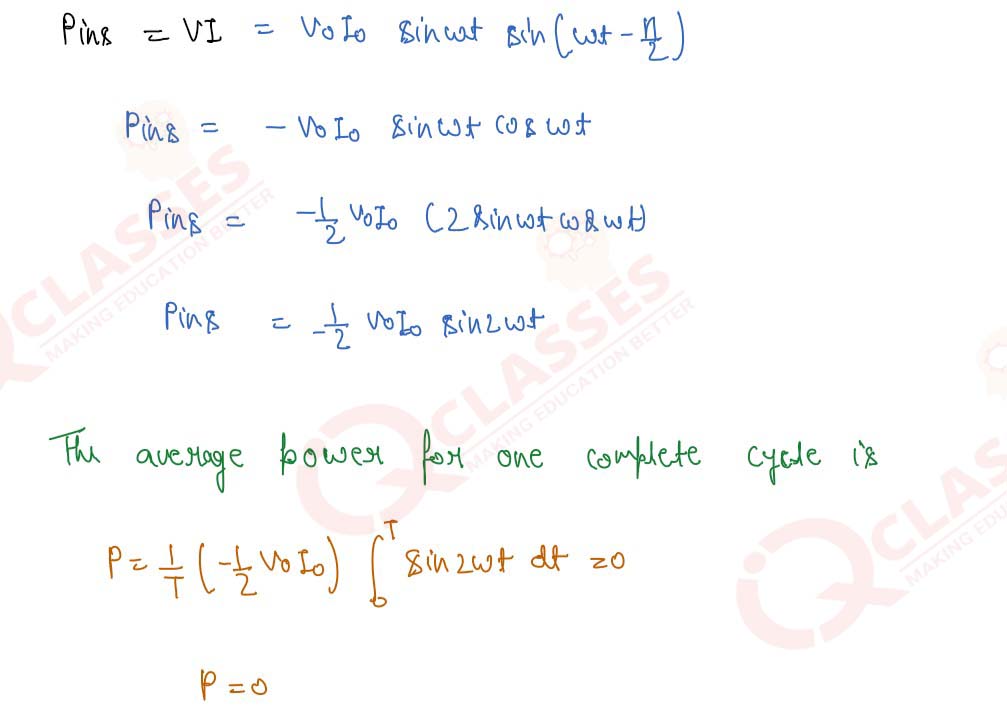
Prove that an ideal inductor does not dissipate power in an AC circuit.
Solution



20
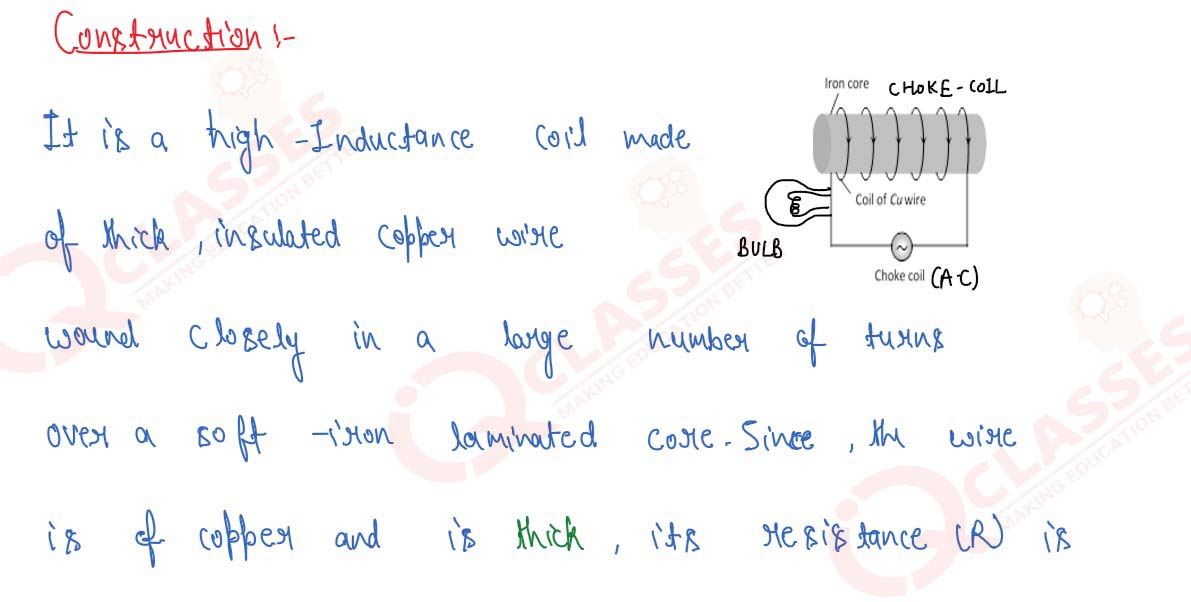
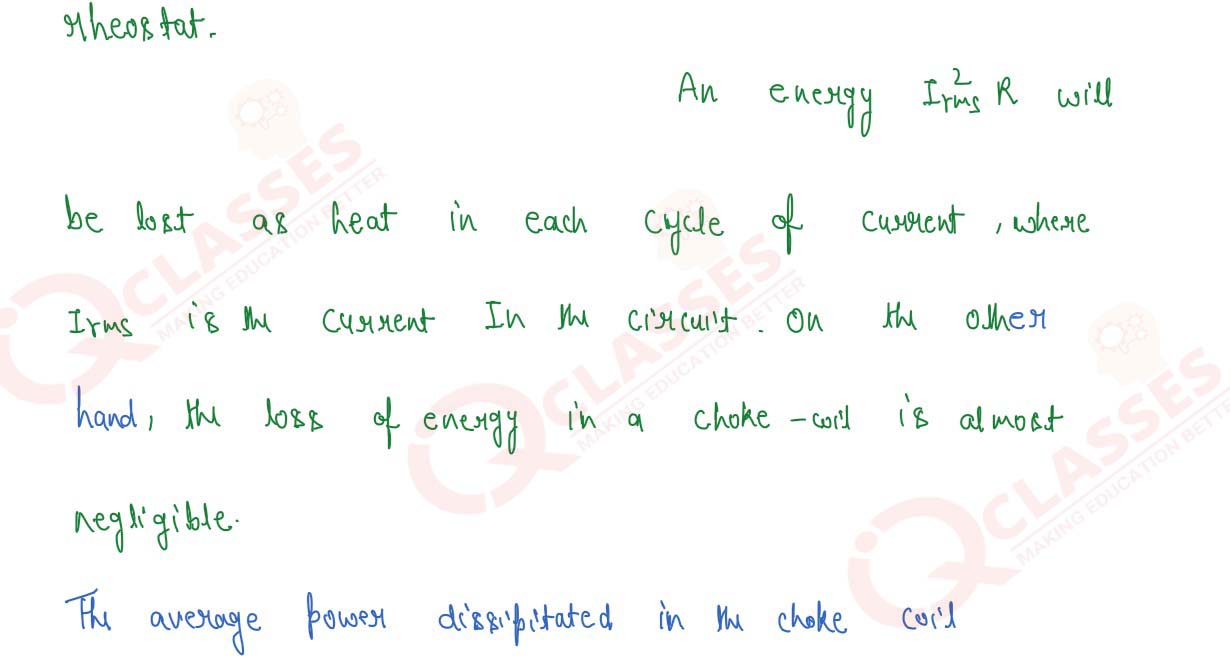
Explain the principle, construction and working of a
choke coil in controlling current in an AC circuit. Why
is it preferred to resistance in AC circuits?
Solution












21
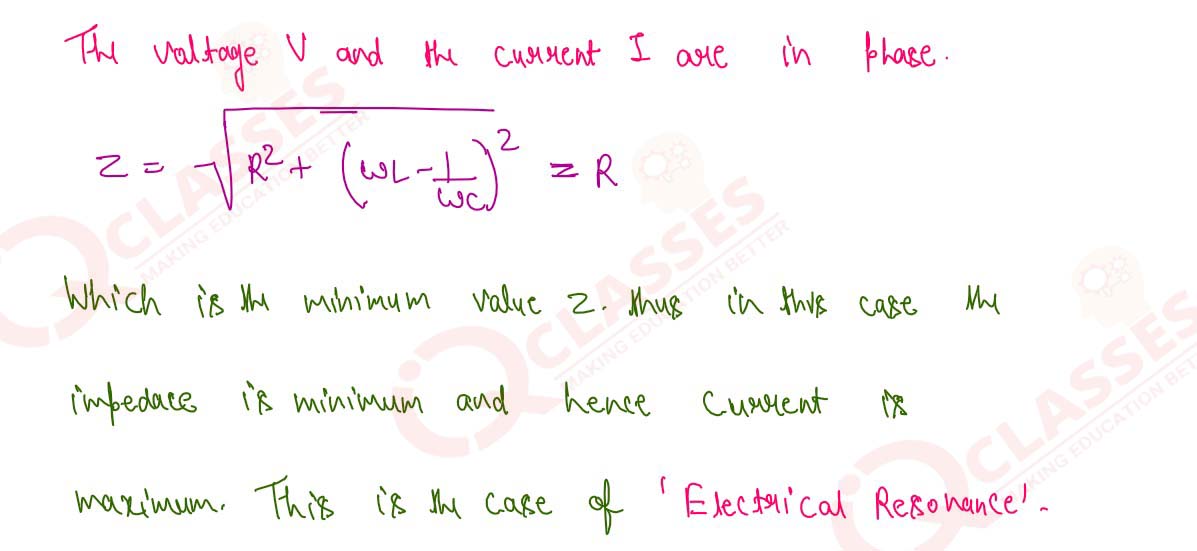
Discuss the phenomenon of resonance in an L-C-R
series AC circuit.
Solution
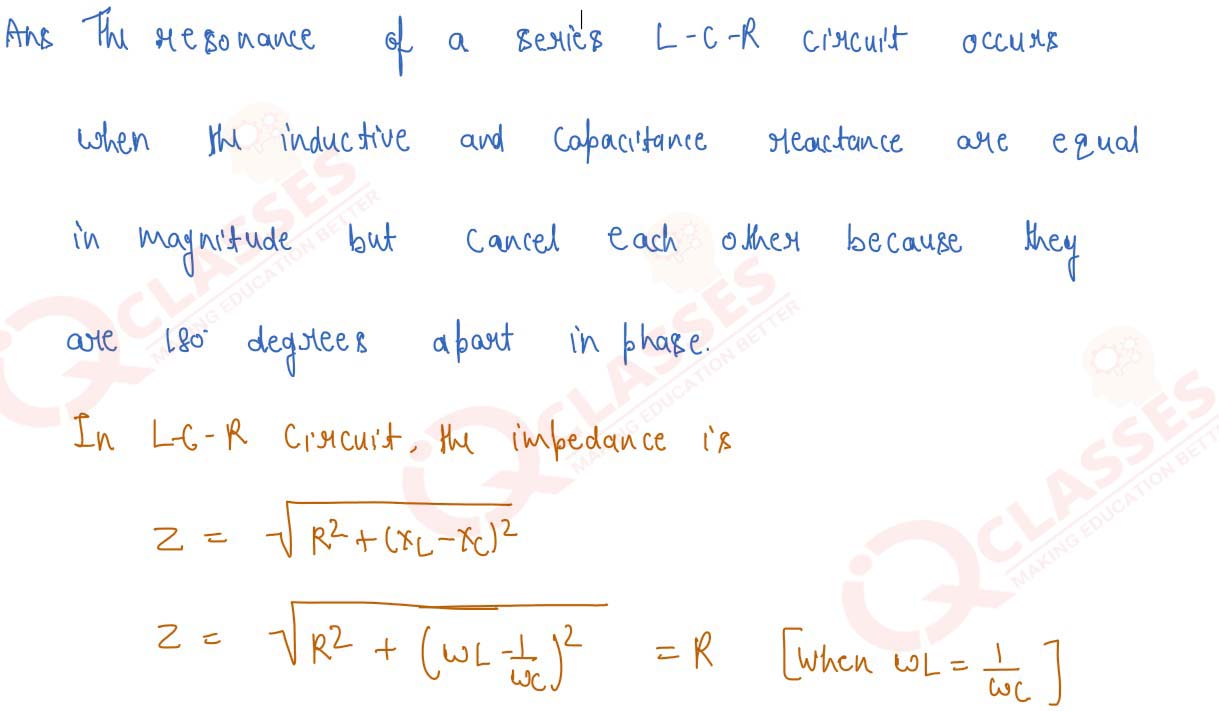
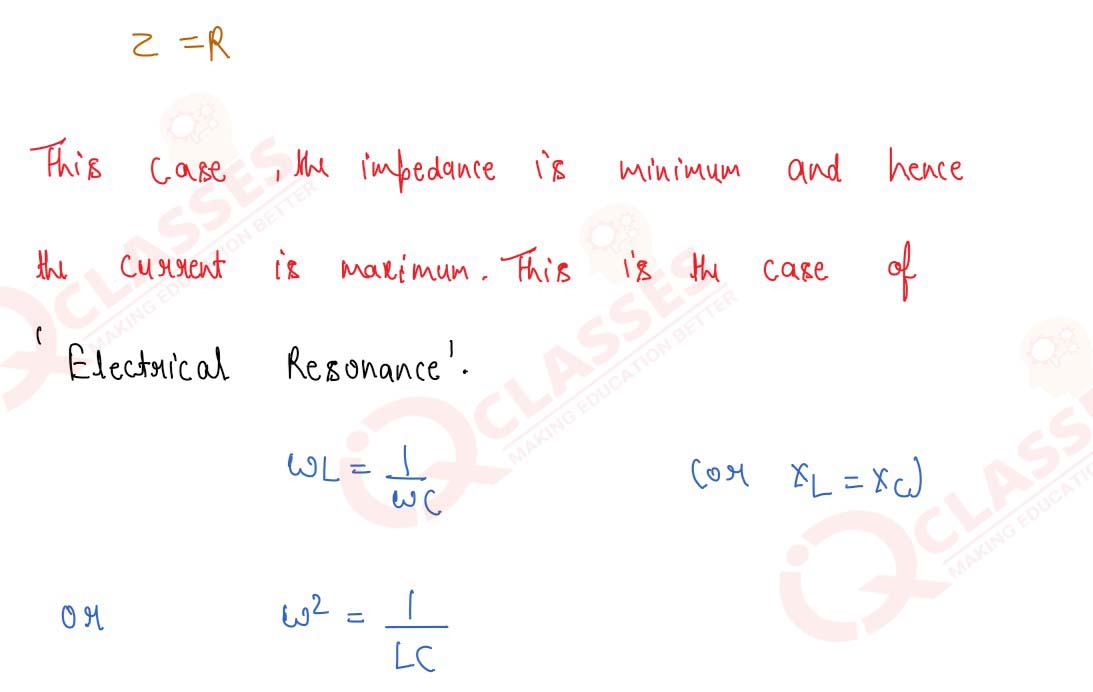


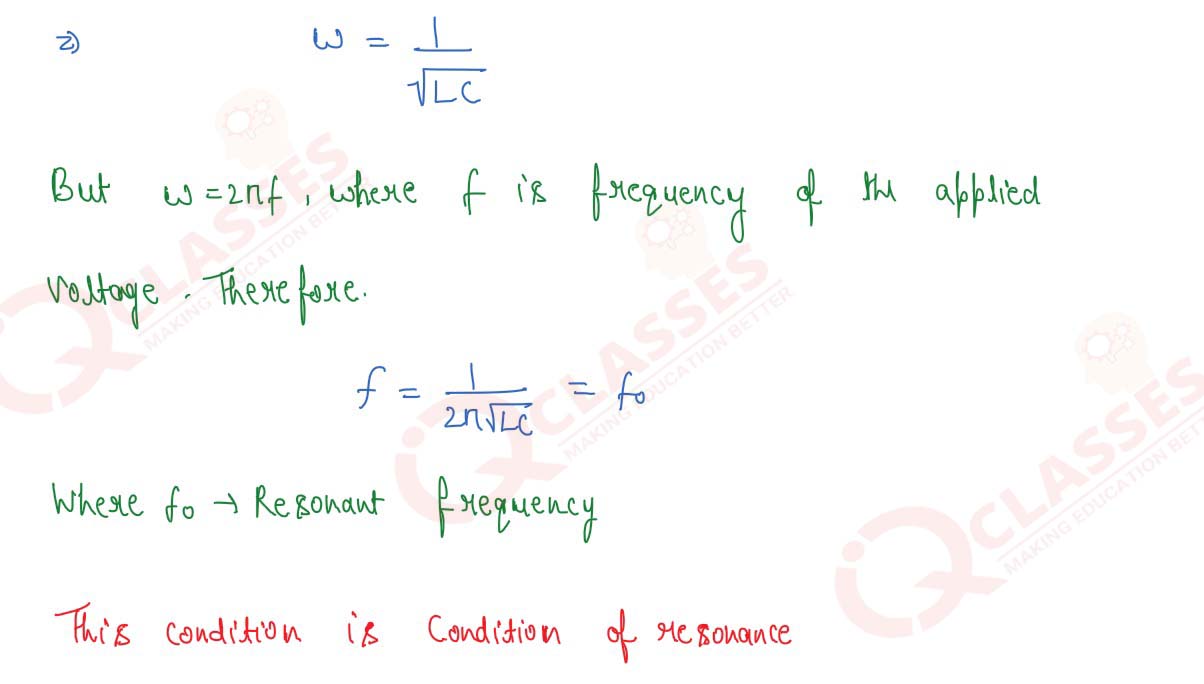





22
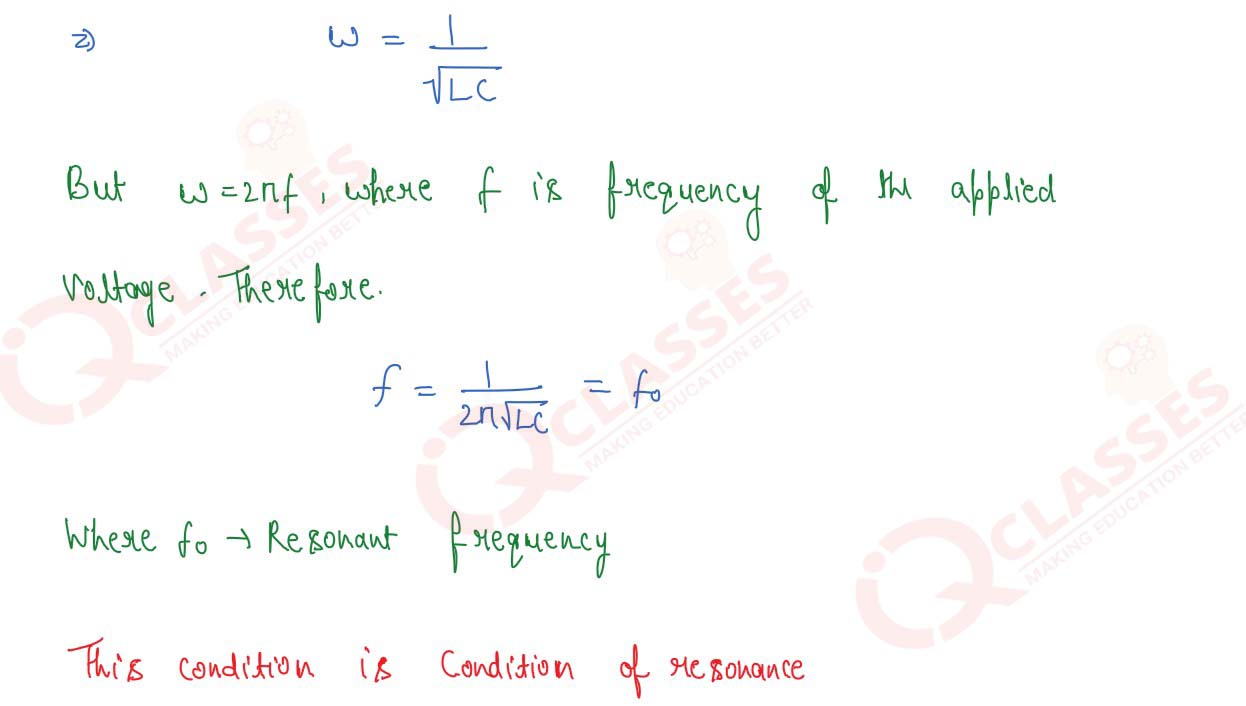
Obtain an expression for resonant frequency fo of a series L-C-R circuit
Solution
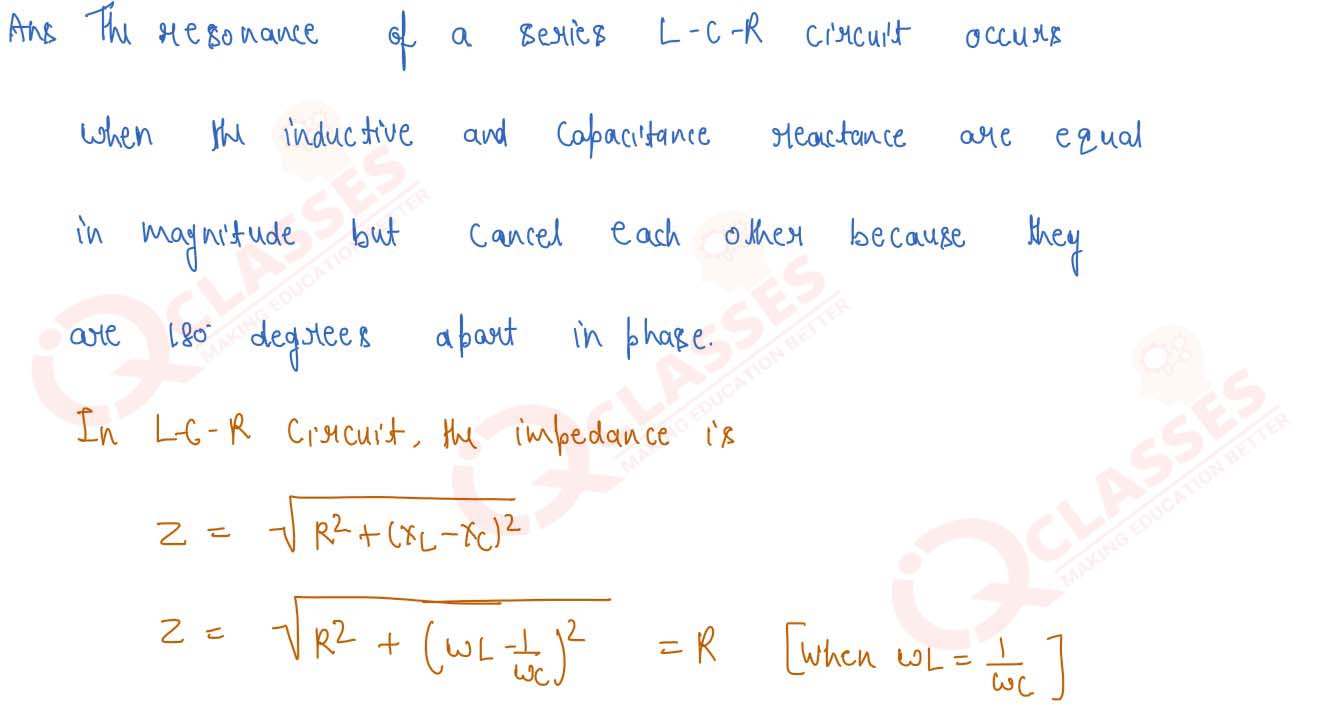

23
In series L-C-R circuit, obtain an expression for the resonant frequency
Solution