Q1. Show that each of the following is a composite number:
(i) 5 x 11 x 13 + 13
(ii) 6 x 5 x 4 x 3 x 2 x 1 + 5

Solution

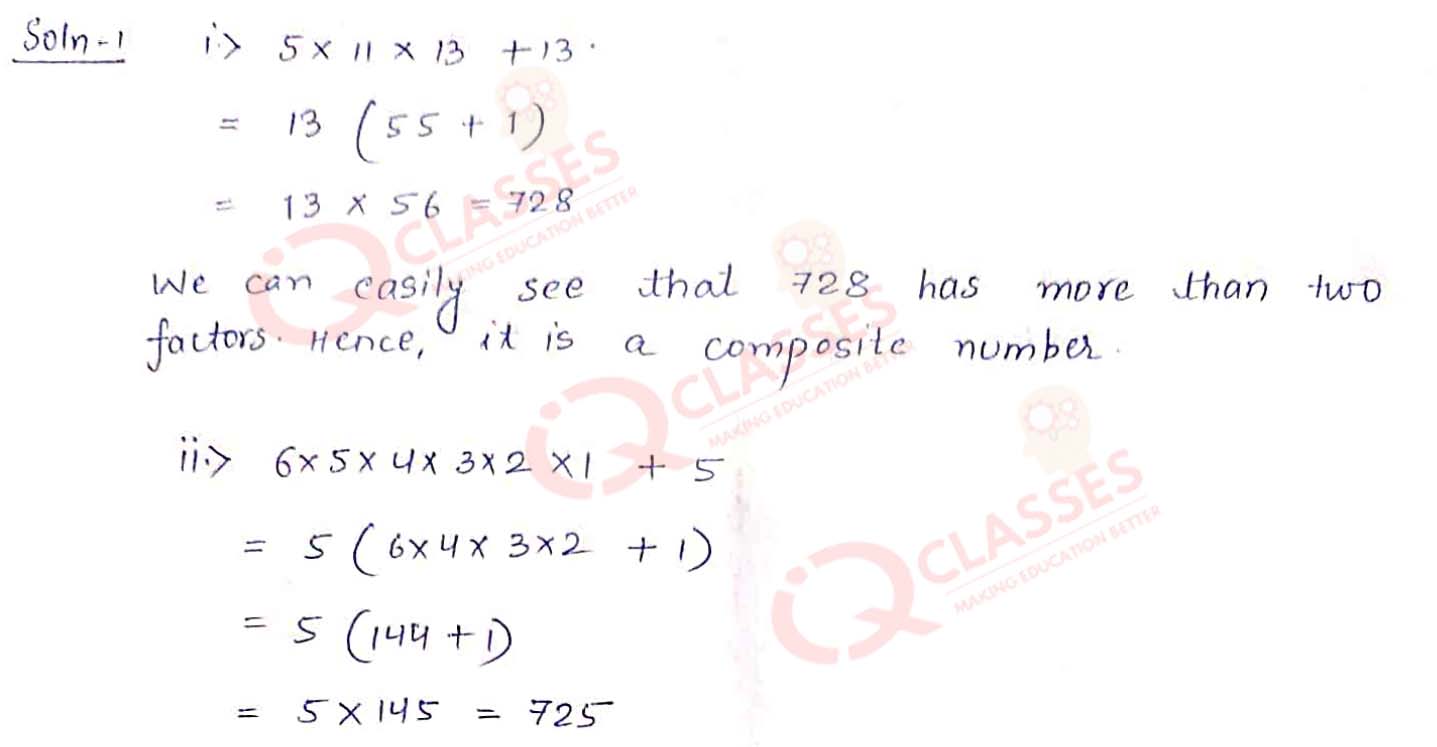
We can see that 725 has more than two factors hence it is a composite number.

Q2. Show that any number of the form 6", where n∈N can never end with the digit 0.

Solution

If 6ⁿ ends with 0, it must have 5 as a factor.
But, 6ⁿ = (2x3)ⁿ = 2ⁿ X 3ⁿ------------(1)
from equation (1),
we can easily see that 2 and 3 are only prime factors of 6ⁿ.
=> 5 is not a factor of on can be concluded
Hence, 6ⁿ can never end with 0.

Q3. Find the HCF and LCM of 612 and 1314 using prime factorization method.

Solution

612 = 2 X 2 X 3 x 3 x 17 = 2² x 3² x 17.
1314 = 2 X 3 X 3 X 73 = 2 x 3² x 73.
=> HCF (612, 1314) = prod. of common terms with lowest powers
= 2 x 3² = 18
LCM (612, 1314) = prod. of prime factors with highest power
= 2² x 3²x 17 x 73 = 44676

Q4. Given that HCF(252, 594) = 18, find LCM(252, 594).

Solution

Two numbers Given = 252 and 594
HCF (252, 594) = 18
Let LCM (252, 594) = x.
Now, we know that,

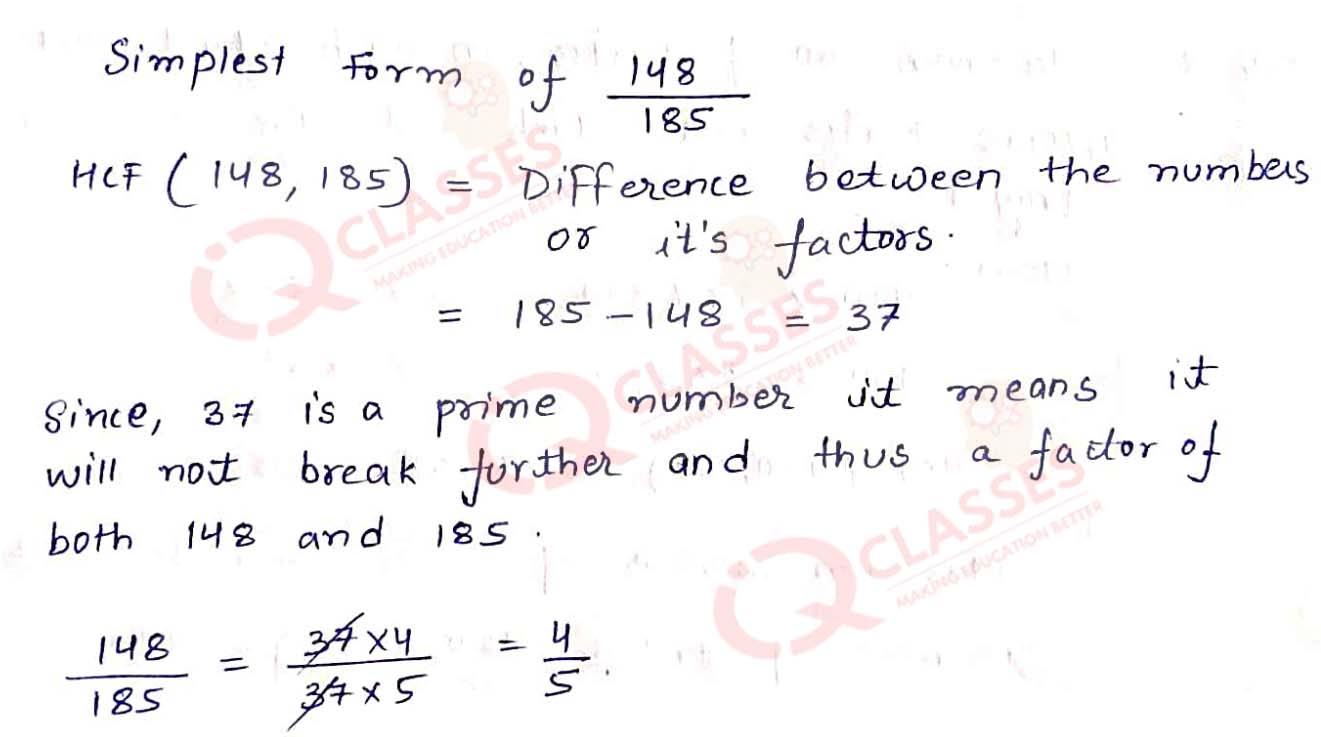
Q5. Find the simplest form of 148/185

Solution

Q6. Find the largest number that will divide 398, 436 and 542, leaving remainders 7, 11 and 15 respectively.

Solution

It means, the required number must divides
(398- 7) = 391, (436 - 11) = 425 , (542 -15) = 527
Required number -> HCF (391, 425, 527)
Now,
391 = 17 x 23
425 = 52 x 17
527 = 17 x 31

HCF (391 , 425 , 527) = 17
Required Number = 17

Q7. An army contingent of 612 members is to march behind an army band of 48 members in a parade. The two groups are to march in the same number of columns. What is the maximum number of columns in which they can march?

Solution

Maximum Number of columns => HCF (612,48)
Now, 612 = 2 x 2 x 3 x 3 x 17 = 2² x 3² x 17
48 = 2⁴ x 3
HCF = 2² x 3 = 12
-> Required Number of columns in which they can march = 12.

Q8. A sweetseller has 420 kaju burfis and 150 badam burfis. He wants to stack them in such a way that each stack has the same number, and they take up the least area of the tray. How many of these can be placed in each stack? How many stacks are formed?

Solution

Maximum no. of Barfis in each stack → HCF (420,150)
#(more Burfis in a stack will take less area of tray.)
Now,
420 => 6 X 7 X 10 = 2²x3x5x7
150 =>2x3x5²= 2x3x5²
HCF (420,150) => 2x3x5 = 30
Therefore, Maximum number of Barfis in each stack = 30.
Therefore, Number of Stacks =(420/30 + 150/30 ) = 14+5 = 19

Q9. In a school there are two sections, namely A and B, of class X. There are 30 students in section A and 28 students in section B. Find the minimum number of books required for their class library so that they can be distributed equally among students of section A or section B.

Solution

Required number of books are to be distributed equally among the students of Section A or B.

So, Number of such books is LCM (30,28)
Now,
30=2x3x5
28 = 2²x7
LCM = product of prime factors with highest power.

= 2²x3x5x7 = 420
Hence, the Required number of books = 420

Q10. Use Euclid's algorithm to find HCF of 1651 and 2032. Express the HCF in the form 1651m + 2032n.

Solution

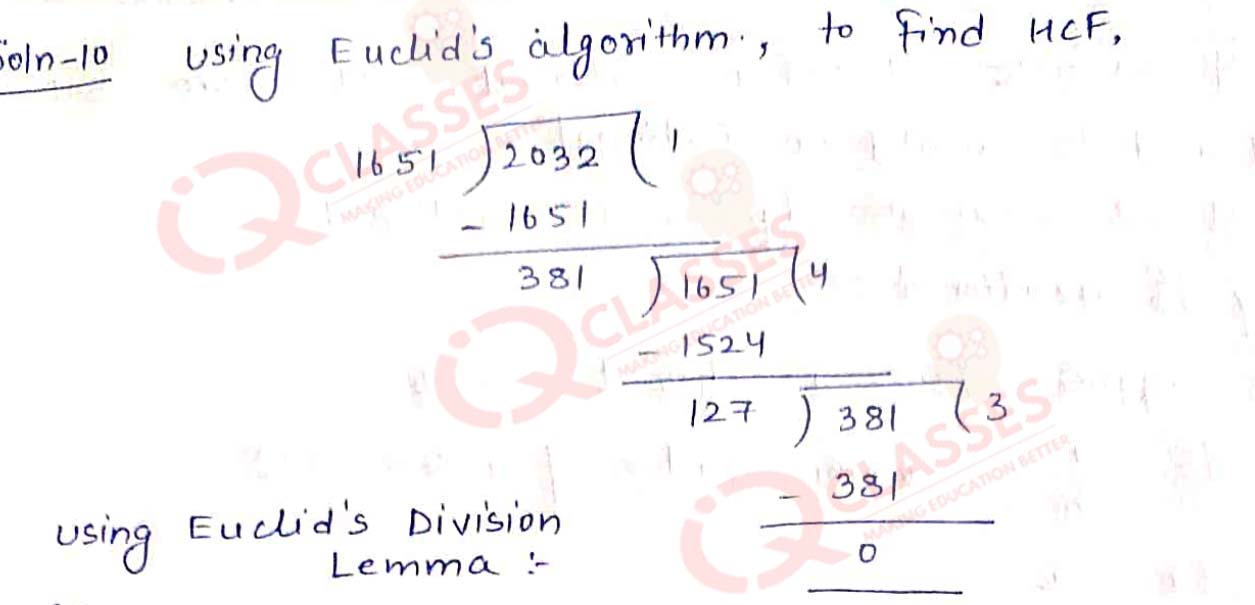
Step-1
1651 X 1 + 381 = 2032
Step-2
381 x 4 + 127 = 1651
Step-3
127 x 3 + 0 = 381
The Remainder is now 0 in 3^rd step so our process stop
=> HCF (1651 , 2032) = 127.

Q11. Show that every positive odd integer is of the form (6m + 1) or (6m + 3) or (6m + 5)for some integer m.

Solution

Let n be a given positive odd integer. On dividing n by 6,let m be the quotient and r be the remainder.Then, by Euclid's Division Lemma,we have

n=6m+r (where, 0 ≤ r < 6)
So, n=6m or 6m+1, or 6m+2, 6m+3,6m+4,6m+5
But n= 6m or 6m+2 or 6m+ 4 are even
(2(3m)) = 2(3m+1) = 2(3m+2)
since they are multiple of 2
Thus, when n is odd, it's of the form 6m+1, 6m+3 or 6m+5 for some integer m.

Q12. Prove that (4 - √3) is irrational number, given that,√3 is an irrational number.

Solution

if possible (4-√3) is Rational,it means both 4 and √3 would be Rational. But we know already √3 is irrational.
Proving √3 irrational :
Let us suppose √3 =a rational number
Therefore √3 = a/b (common factor of a a and b is 1)
√3 b = a
or 3b²=a² -----1
Since , 3 is a factor of a², so 3 will also be a factor of a.So we can write a = 3c for some integer c.

Now, from equation 2, 3 is a factor of "b²" and hence, a factor of "b" as well
Thus, 3 is a common factor of a and b.
But this contradicts the fact that a and b have no common factor other than 1.
Hence √3 is not rational

Q13. Prove that ((3-4√2)/7) is irrational number, given that √2 is an irrational.

Solution

Q14. Which of the following has a terminating decimal expansion?

1. 32/91
2. 19/80
3. 23/45
4. 25/42

Solution

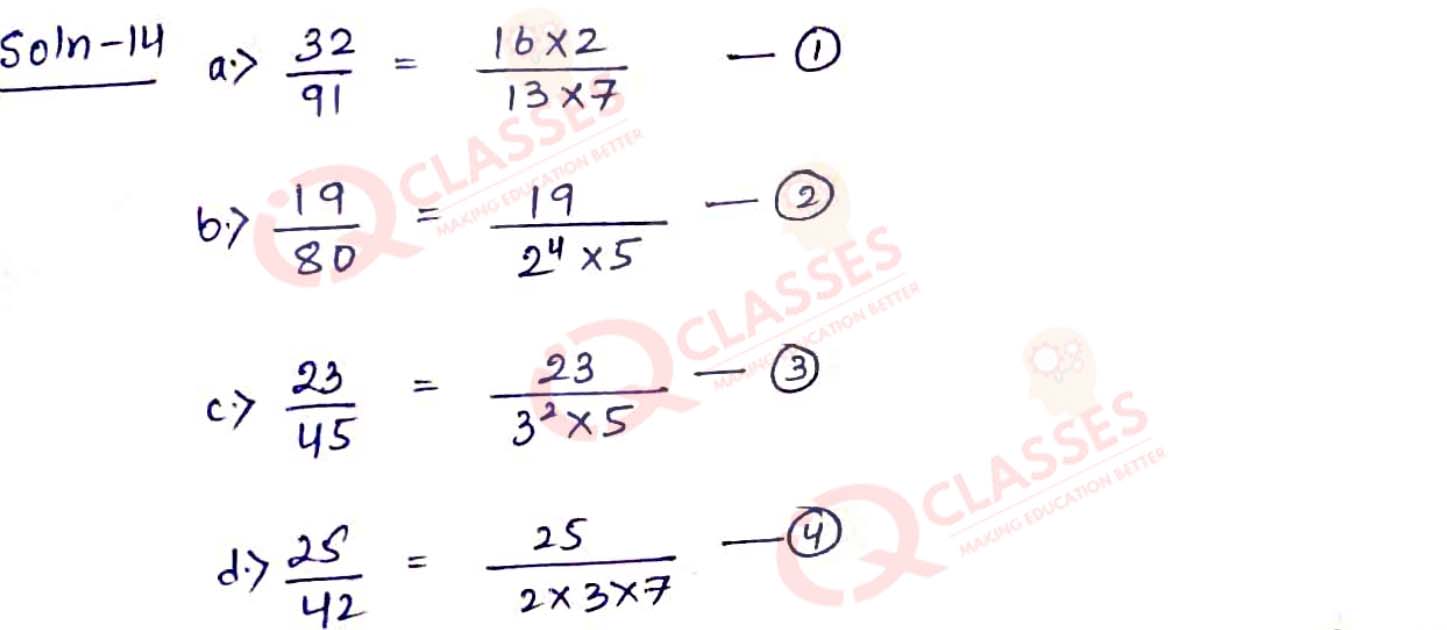
A number is terminating decimal, if denominator is of the form of 2^m x 5ⁿ (m and n are non-negative integers.) so, option (b) is terminating decimal expansion.

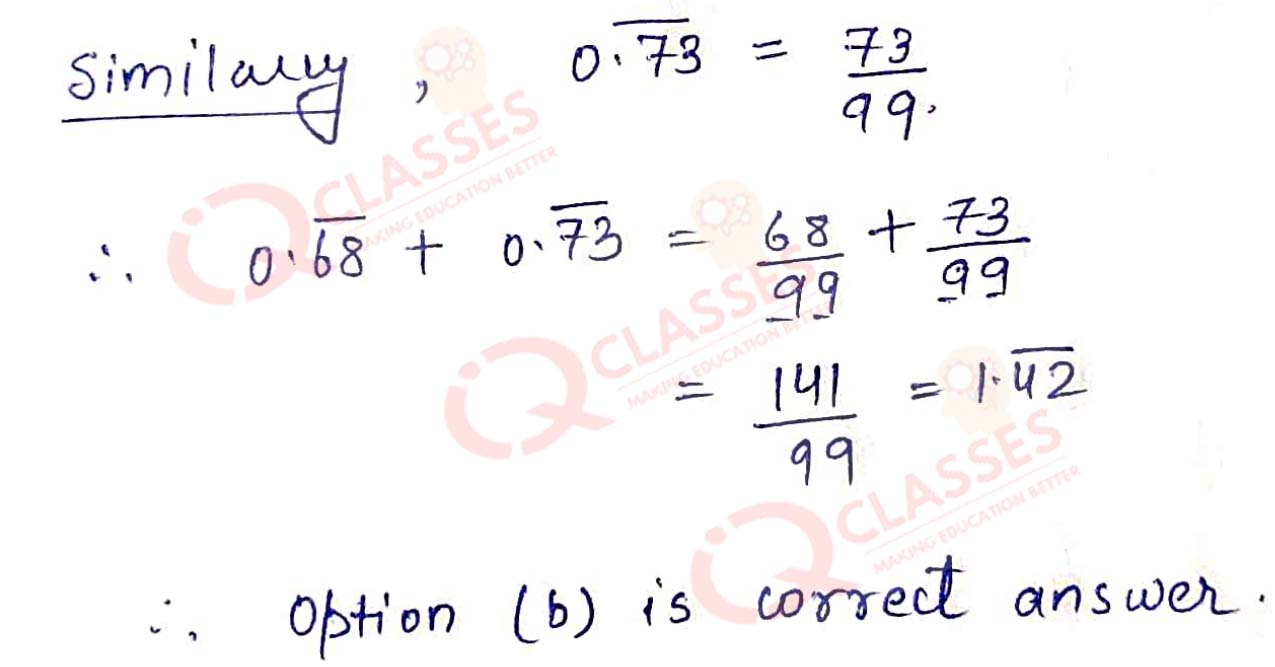
Q15. 0.68 + 0.73 =?

1. 1.41
2. 1.42
3. 0.41
4. None of these

Solution