Class X ICSE Maths Chapter Equation Of Line Exercise 12.1
Welcome to our extensive collection of questions on the topic "Equation of a Line," crafted to help you master this fundamental concept in coordinate geometry. Our question bank covers key aspects such as slope-intercept form, point-slope form, general form, and finding equations of parallel and perpendicular lines. These questions are ideal for students aiming to strengthen their understanding of line equations and their applications in solving real-world problems. Whether you're preparing for exams or simply improving your geometry skills, our resource provides the perfect platform to practice and excel in the "Equation of a Line."
Select Exercise
Q3 Find the equation of a straight line parallel 1 to x-axis which is at a distance
(i) 2 units above it
(ii) 3 units below it.
Q4 Find the equation of a straight line parallel to y-axis which is at a distance of:
(i) 3 units to the right
(ii) 2 units to the left.
Q5 Find the equation of a straight line parallel to y-axis and passing through the point ( - 3, 5).
Q6 Find the equation of the a line whose
(i) slope = 3, y-intercept = - 5
(ii) slope = -2/7, y-intercept = 3
(iii) gradient = √3, y-intercept = -4/3
(iv) inclination = 30°,y-intercept = 2
Q7 Find the slope and y-intercept of the following lines:
(i) x - 2y - 1 = 0
(ii) 4x - 5y - 9 = 0
(iii) 3x +5y + 7 = 0
(iv) x/3 + y/4=1
(v) y - 3 = 0
(vi) x - 3 = 0
Q8 The equation of the line PQ is 3y - 3x + 7 = 0
(i) Write down the slope of the line PQ.
(ii) Calculate the angle that the line PQ makes with the positive direction of x-axis.
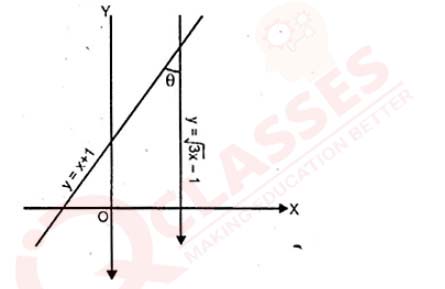
Q9 The given figure represents the line y = x + 1 and y = √3x - 1. Write down the angles which the lines make with the positive direction of the x-axis. Hence determine θ.
Q10 Find the value of p, given that the line y/2=x-p passes through the point ( - 4, 4)
Q12 The graph of the equation y = mx + c passes through the points (1, 4) and ( - 2, - 5). Determine the values of m and c.
Q13 Find the equation of the line passing through the point (2, - 5) and making an intercept of - 3 on the y-axis.
Q14 Find the equation of a straight line passing through ( - 1, 2) and whose slope is 2/5.
Q15 Find the equation of a straight line whose inclination is 60° and which passes through the point (0, - 3).
Q16 Find the gradient of a line passing through the following pairs of points.
(i) (0, - 2), (3, 4)
(ii) (3, - 7), ( - 1, 8)
Q17 The coordinates of two points E and F are (0, 4) and (3, 7) respectively. Find :
(i) The gradient of EF
(ii) The equation of EF
(iii) The coordinates of the point where the line EF intersects the x-axis.
Q19 Find the equation of the line passing through the points P (5, 1) and Q (1, - 1). Hence, show that the points P, Q and R (11, 4) are collinear.
Q20 Find the value of ‘a’ for which the following points A (a, 3), B (2,1) and C (5, a) are collinear. Hence find the equation of the line.
Q21 Use a graph paper for this question. The graph of a linear equation in x and y, passes through A ( - 1, - 1) and B (2, 5). From your graph, find the values of h and k, if the line passes through (h, 4) and (½, k).
Q22 ABCD is a parallelogram where A (x, y), B (5, 8), C (4, 7) and D (2, - 4). Find
(i) the coordinates of A
(ii) the equation of the diagonal BD.
Q23 In ∆ABC, A (3, 5), B (7, 8) and C (1, - 10). Find the equation of the median through A.
Q24 Find the equation of a line passing through the point ( - 2, 3) and having x-intercept 4 units.
Q26 A(2,5),B(−1,2) and C(5,8) are the vertices of a triangle ABC, 'M' is a point on AB such that AM : MB =1:2. Find the co-ordinates of 'M'. Hence find the equation of the line passing through the points C and M.
Q27 Find the equation of the line passing through the point (1, 4) and intersecting the line x - 2y - 11 = 0 on the y-axis.
Q28 Find the equation of the straight line containing the point (3, 2) and making positive equal intercepts on axes.
Q29 Three vertices of a parallelogram ABCD taken in order are A (3, 6), B (5, 10) and C (3, 2) find:
(i) the coordinates of the fourth vertex D.
(ii) length of diagonal BD.
(iii) equation of side AB of the parallelogram ABCD.
Q30 A and B arc two points on the x-axis and y-axis respectively. P (2, - 3) is the mid point of AB. Find the
(i) the co-ordinates of A and B.
(ii) the slope of the line AB.
(iii) the equation of the line AB.
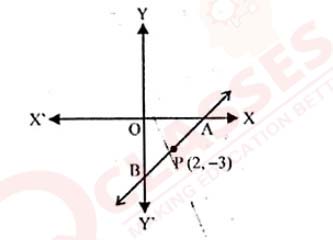
Q31 M and N are two points on the X-axis and Y-axis respectively. P(3,2) divides the line segment MN in the ratio 2:3. Find
(i) The coordinate of M and N
(ii)the slope of the line MN.
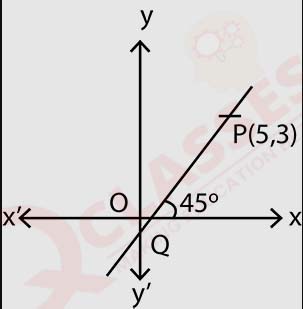
Q32 The line through P(5,3) intersects y-axis at Q.
(i) Write the slope of the line.
(ii) Write the equation of the line.
(iii) Find the co-ordinates of Q.
Q33 (i) Write down the co-ordinates of the point P that divides the line joining A(4,1) and B(17,10) in the ratio 1:2.
(ii) Calculate the distance OP where O is the origin.
(iii) In what ratio does the y-axis divide the line AB?
Q34 Find the equation of the diagonals of a rectangle whose sides are x=−1,x=2,y=−2 and y=6
Q35 Find the equation of a straight line passing through the origin and through the point of intersection of the lines 5x + 1y - 3 and 2x - 3y = 7


Add a comment