Class 12 CBSE Maths Specimen Question 2023
Maximum Marks: 80
Time Allowed: Three hours
This Question paper contains - five sections A, B, C, D and E. Each section is compulsory. However, there
are internal choices in some questions.
Section A has 18 MCQ’s and 02 Assertion-Reason based questions of 1 mark each.
Section B has 5 Very Short Answer (VSA)-type questions of 2 marks each.
Section C has 6 Short Answer (SA)-type questions of 3 marks each.
Section D has 4 Long Answer (LA)-type questions of 5 marks each.
Section E has 3 source based/case based/passage based/integrated units of
assessment (4 marks each) with sub parts.
class 12 CBSE Maths Specimen Question Paper 2024
SECTION A
Question 1
If A =[aij] is a square matrix of order 2 such that
,then
A2 is
If A and B are invertible square matrices of the same order, then which of the following is not correct?
- |AB-1|=|A|/|B|
- |(AB)-1|=1/|A||B|
- (AB)-1=B-1A-1
- (A+B)-1=B-1+A-1
If the area of the triangle with vertices (-3 ,0) , (3,0) and (0, k) is 9 sq units, then the value/s of
k will
- 9
- ± 3
- -9
- 6
If
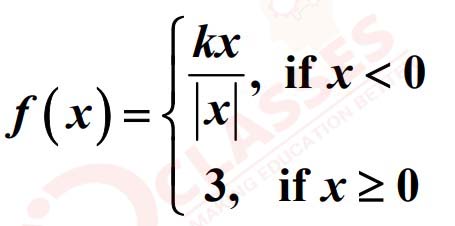,is
continuous at x = 0 , then the value of k is
(a) −3
(b) 0
(c) 3
(d) any real number
The lines
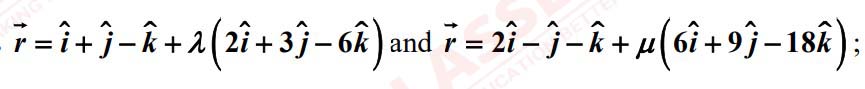,(where
λ and &meu; are scalars) are:
(a) coincident
(b) skew
(c) intersecting
(d) parallel
The degree of the differential equation
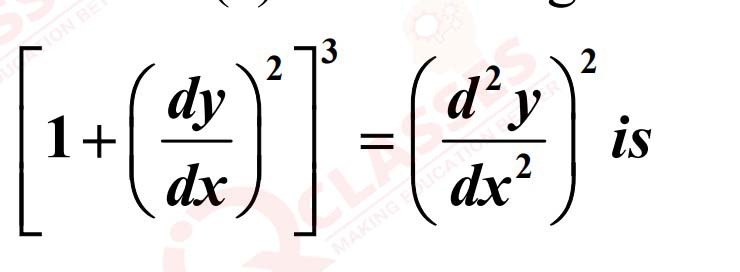
(a) 4
(b) 3
(c) 2/3
(d) Not defined
The corner points of the bounded feasible region determined by a system of linear constraints are (0, 3)
, (1,1) and (3,0) . Let Z=px+qy , where p,q > 0 . The condition on p and q so that the
minimum of Z occurs at (3,0) and (1,1) is
(a) p=2q
(b) p=q/2
(c) p=3q
(d) p=q
ABCD is a rhombus whose diagonals intersect at E. Then EA->+ EB->+ EC-> ED-> equals to
(a) 0->
(b) AD ->
(c) 2BD ->
(d) 2AD->
For any integer n, the value of
(a) -1
(b) 0
(c) 1
(d) 2
The value of |A| , if
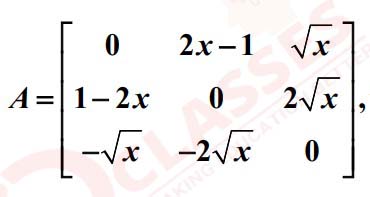where x
∈ R+,is
(a) (2x+1)2
(b) 0
(c) (2x+1)3
(d) (2x-1)2
The feasible region corresponding to the linear constraints of a Linear Programming Problem is given
below.
Which of the following is not a constraint to the given Linear Programming Problem?
(a) x +y≥ 2
(b) x+2y≤ 10
(c) x-y ≥ 1
(d) x-y≤ 1
If
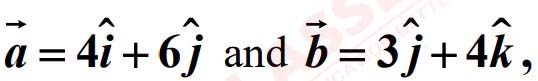, then
the vector form of the component of a-> along b-> is
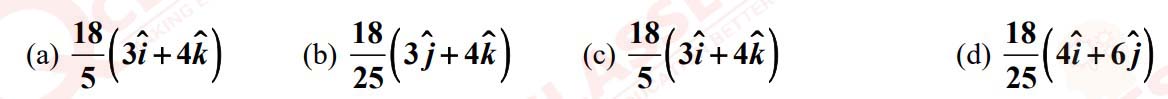
Given that A is a square matrix of order 3 and |A|=-2,then |adj(2A)| is equal to
(a) -26
(b) +4
(c) -28
(d) 28
A problem in Mathematics is given to three students whose chances of solving it are 1/2,1/3,1/4
respectively. If the events of their solving the problem are independent then the probability that the
problem will be solved, is
(a) 1/4
(b) 1/3
(c) 1/2
(d) 3/4
The general solution of the differential equation ydx xdy x y – 0; (Given x,y>0), is of the form
(a) xy=c
(b) x=c y2
(c) y =cx
(d) y=cx2;
(Where ' ' c is an arbitrary positive constant of integration)
The value of λ for which two vectors
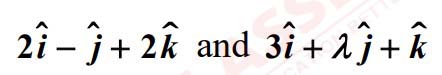are
perpendicular is
(a) 2
(b) 4
(c) 6
(d) 8
The set of all points where the function f(x) =x+ |x| is differentiable, is
(a) (0,∞)
(b) (-∞,0)
(c) (-∞,0)∪ (0,∞)
(d) (-∞,∞)
If the direction cosines of a line are < 1/c,1/c,1/c> then
(a) 0 < c < 1
(b) c > 2
(c) c=±√ 2
(d) c = ±√ 3
TIn the following questions, a statement of Assertion (A) is followed by a
statement of Reason (R).
Choose the correct answer out of the following choices.
(a) Both (A) and (R) are true and (R) is the correct explanation of (A).
(b) Both (A) and (R) are true but (R) is not the correct explanation of (A).
(c) (A) is true but (R) is false.
(d) (A) is false but (R) is true.
Let f(x) be a polynomial function of degree 6 such that
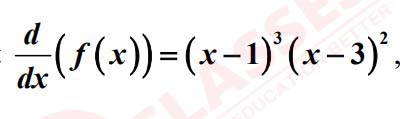
ASSERTION (A): f (xdy) has a minimum at x = 1.
REASON (R): When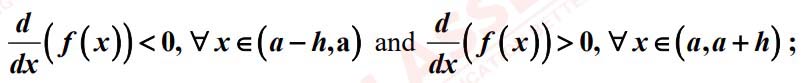 
where
'h' is an infinitesimally small positive quantity, then f (x) has a minimum at x=a,
provided f(x) is continuous at x=a .
ASSERTION (A): The relation f:{1,2,3,4} -> {x,y,z,p} defined by f={(1,x),(2,y),(3,z)} is a
bijective function.
REASON (R): The function f:{1,2,3} -> {x,y,z,p} such that f={(1,x),(2,y),(3,z)} is one-one.
SECTION B
[This section comprises of very short answer type questions (VSA) of 2 marks each}
Question 21
Find the value of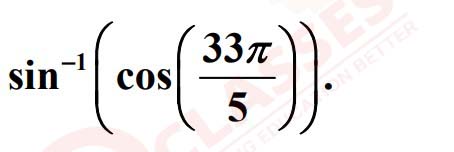 
OR
Find the domain of sin-1(x2-4)
Find the interval/s in which the function f:R->R defined by f(x)=xex,is increasing
If
,, then
find the maximum value of f(x)
OR
Find the maximum profit that a company can make, if the profit function is given by
P(x)=72+42x-x2,where x is the number of units and P is the profit in rupees.
Evaluate:
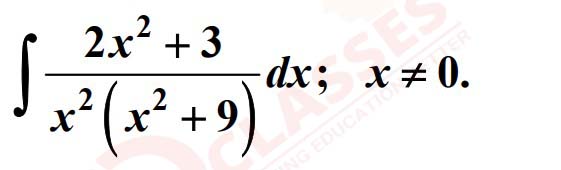
Check whether the function f : R->R defined by f(x)=x3+x has any critical point/s or not ? If yes, then find the point's.
SECTION C
[This section comprises of short answer type questions (SA) of 3 marks each]
Question 26
Find:
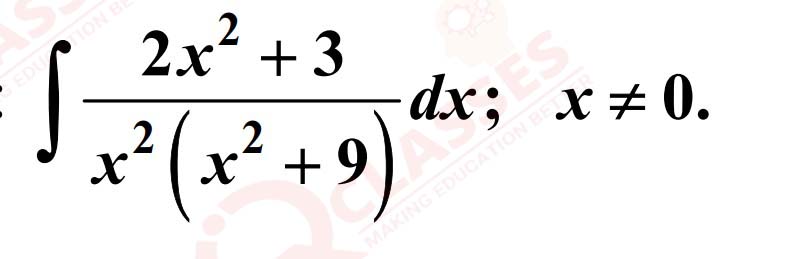
The random variable X has a probability distribution P X of the following form, where 'k' is some
real number:
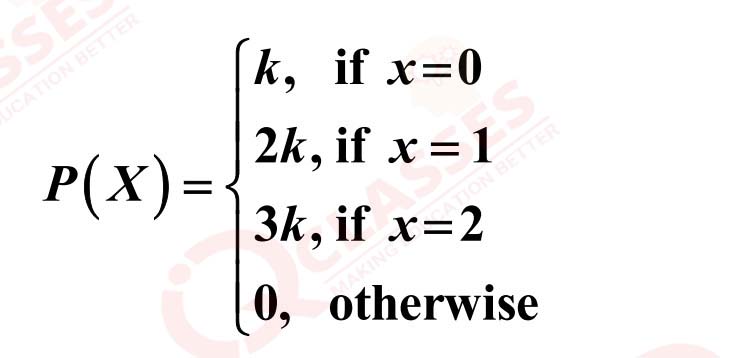
(i) Determine the value of k.
(ii) Find P (X < 2) .
(iii) Find P(X> 2)
Find:
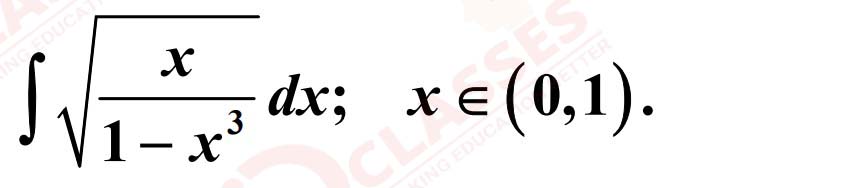
OR
Evaluate:
Solve the differential equation:
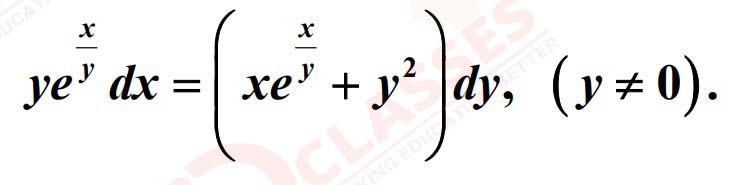
OR
Solve the differential equation:
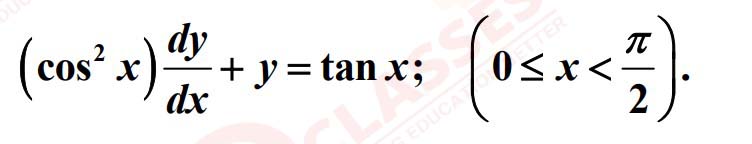
Solve the following Linear Programming Problem graphically:
Minimize:z=x+2y ,
subject to the constraints: x+ 2y≥ 100, 2x-y≤0, 2x-y≤200,x,y≥0
OR
Solve the following Linear Programming Problem graphically:
Maximize: z=-x +2y ,
subject to the constraints: x≥3, x +y≥5, x+2y≥6, y≥0
If (a+bx)ey/x=x then prove that
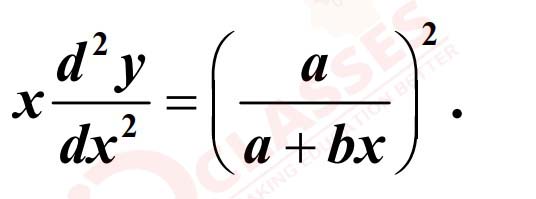
SECTION D
[This section comprises of long answer type questions (LA) of 5 marks each]
Question 32
Make a rough sketch of the region {(x,y):0 ≤y ≤ x2+1,0 ≤ y ≤x+1,0≤ x≤ 2} and find the area of the region, using the method of integration
Let N be the set of all natural numbers and R be a relation on N x N defined by
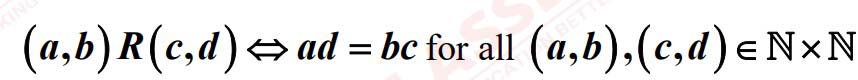.Show
that R is an equivalence relation on N xN. Also, find the equivalence class of (2,6),i.e., [(2,6)]
OR
Show that the function f: R->{x ∈ R: -1 < x< 1} defined by f(x)=x/1+|x|,x ∈ R is one-one and onto
function.
Using the matrix method, solve the following system of linear equations :
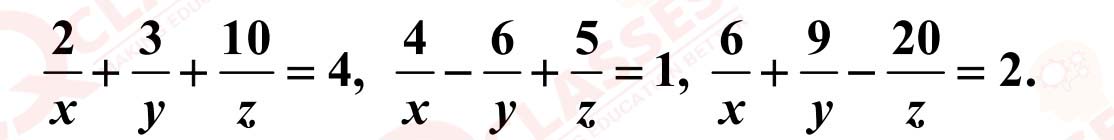
Find the coordinates of the image of the point (1,6,3) with respect to the line
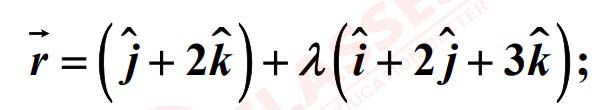
where 'λ' is a scalar. Also, find the distance of the image from the
y-axis
OR
An aeroplane is flying along the line 
where 'λ' is a scalar and another aeroplane is flying
along the line 
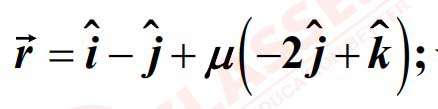where 'µ'is a scalar. At what points on the lines should they reach, so
that the distance between them is the shortest? Find the shortest possible distance between them.
SECTION E
[This section comprises of 3 case- study/passage based questions of 4 marks each with sub parts.
The first two case study questions have three sub parts (i), (ii), (iii) of marks 1,1,2 respectively.
The third case study question has two sub parts of 2 marks each.)
Question 36
Read the following passage and answer the questions given below:
In an Office three employees Jayant, Sonia and Oliver process incoming copies of a certain form. Jayant
processes 50%of the forms, Sonia processes 20% and Oliver the remaining 30% of the forms. Jayant
has an error rate of 0.06 , Sonia has an error rate of 0.04 and Oliver has an error rate of 0.03 .
Based on the above information, answer the following questions.
(i) Find the probability that Sonia processed the form and committed an error.
(ii) Find the total probability of committing an error in processing the form.
(iii) The manager of the Company wants to do a quality check. During inspection, he selects a form at
random from the days output of processed form. If the form selected at random has an error, find the
probability that the form is not processed by Jayant.
OR
(iii) Let E be the event of committing an error in processing the form and let
E1,E2 and E3 be the events that Jayant, Sonia and Oliver processed the
form. Find the value of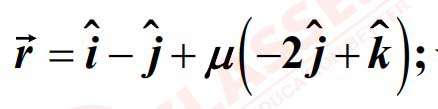
Read the following passage and answer the questions given below:
Teams A B C went for playing a tug of war game. Teams A B C, , have attached a rope to a metal ring and
is trying to pull the ring into their own area.
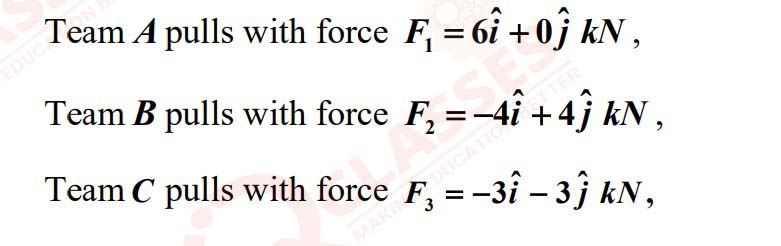
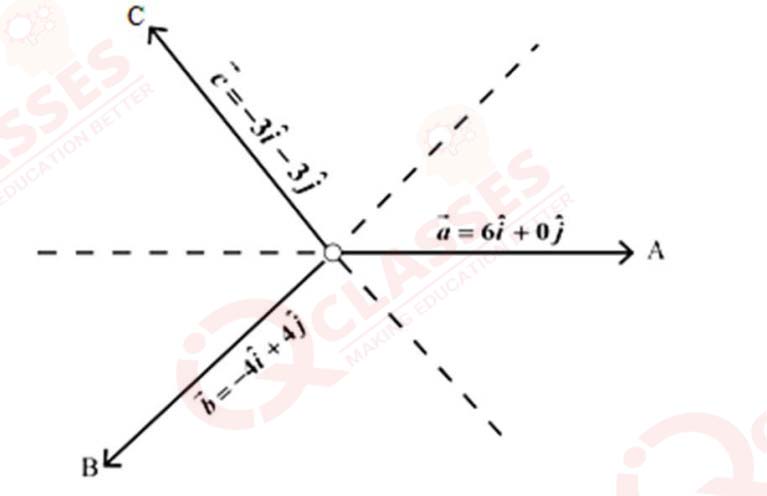
(i) What is the magnitude of the force of Team A ?
(ii) Which team will win the game?
(iii) Find the magnitude of the resultant force exerted by the teams
OR
(iii) In what direction is the ring getting pulled?
Read the following passage and answer the questions given below:
The relation between the height of the plant ('y' in cm) with respect to its exposure to the sunlight is
governed by the following equation y=4x-1/2 x2, , where 'x' is the number of days exposed to
the sunlight, for x ≤ 3.
(i) Find the rate of growth of the plant with respect to the number of days exposed to the sunlight.
(ii) Does the rate of growth of the plant increase or decrease in the first three days?
What will be the height of the plant after 2 days?
Add a comment