Class 12 Maths CBSE Linear Programming Board Questions
Here we provide Class 12 Maths important notes,board questions and predicted questions with Answers for chapter Linear Programming. These important notes,board questions and predicted questions are based on CBSE board curriculum and correspond to the most recent Class 12 Maths syllabus. By practising these Class 12 materials, students will be able to quickly review all of the ideas covered in the chapter and prepare for the Class 12 Board examinations.
2016
Q1
A manufacturer produces two products A and B. Both the products are processed on
two different machines. The available capacity of first machine is 12 hours and that of
second machine is 9 hours per day. Each unit of product A requires 3 hours on both
machines and each unit of product B requires 2 hours on first machine and I hour on
second machine. Each unit of product A is sold at ₹7 profit and that of B at a profit of
₹4. Find the production level per day for maximum profit graphically.
solutions

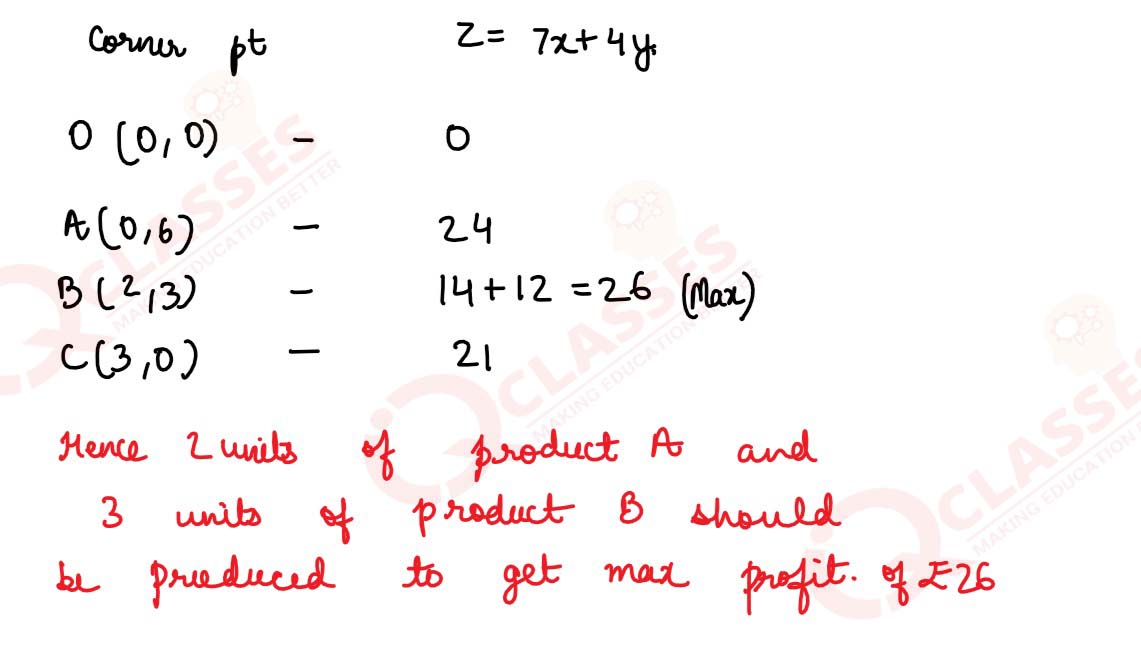
solutions


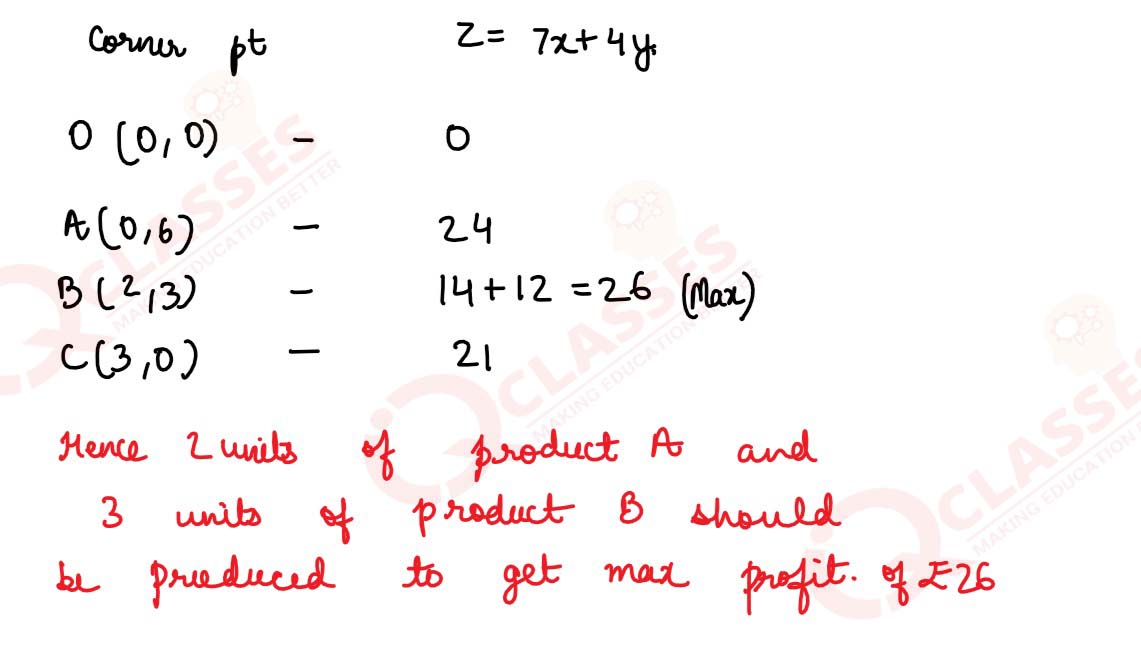
2017
Q1
Two tailors, A and B, earn 300 and 400 per day respectively. A can
stitch 6 shirts and 4 pairs of trousers while B can stitch 10 shirts and
4 pairs of trousers per day. To find how many days should each of them
work and if it is desired to produce at least 60 shirts and 32 pairs of
trousers at a minimum labour cost, formulate this as an LPP.
solutions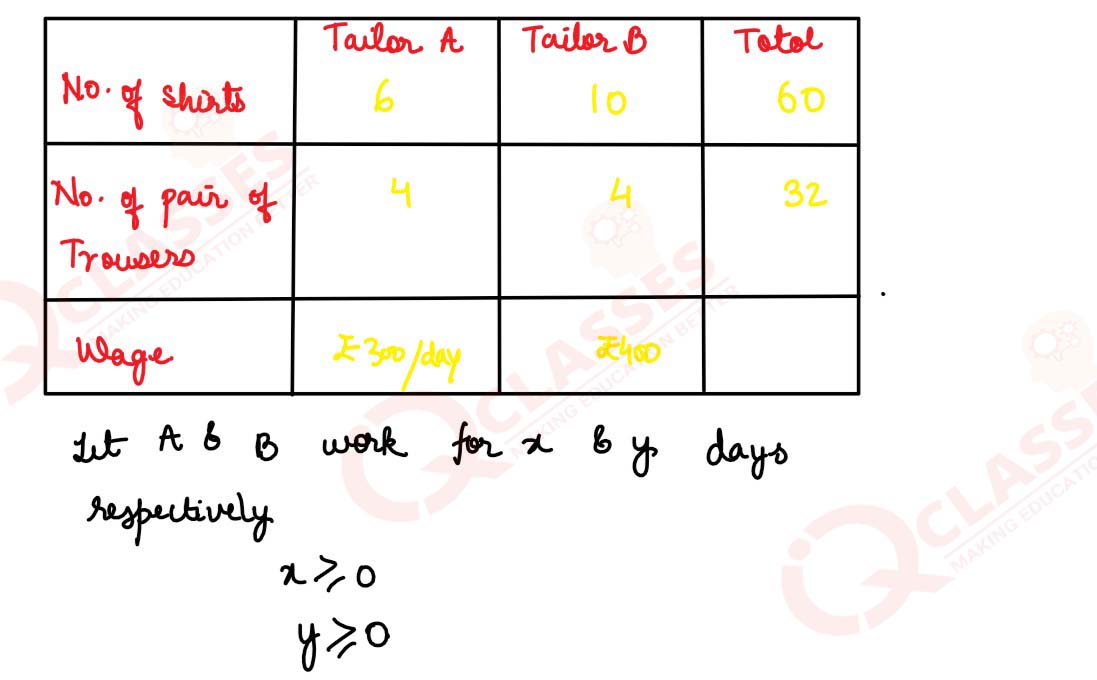

solutions


Q2
Maximise Z = x + 2y
subject to the constraints
x + 2y ≥ 100
2x - y ≤ 0
2x + y ≤ 200
x,y ≥ 0
Solve the above LPP graphically.
solutions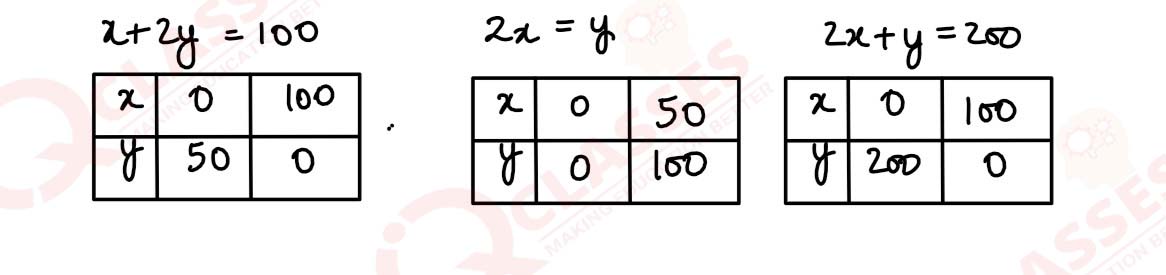


subject to the constraints
x + 2y ≥ 100
2x - y ≤ 0
2x + y ≤ 200
x,y ≥ 0
Solve the above LPP graphically.
solutions



2018
Q1
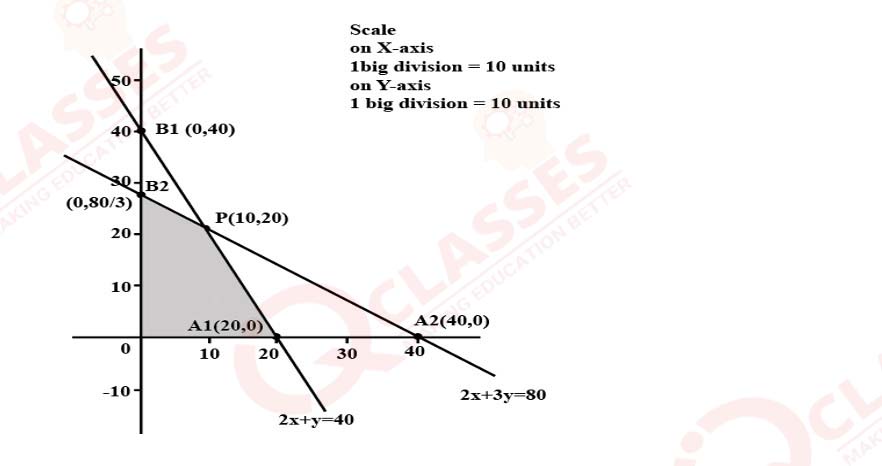
A manufacturer has employed 5 skilled men and 10 semi-skilled men and makes two
models A and B of an article. The making of one item of model A requires 2 hours
work by a skilled man and 2 hours work by a semi-skilled man. One item of model B
requires 1 hour by a skilled man and 3 hours by a semi-skilled man. No man is
expected to work more than 8 hours per day. The manufacturer's profit on an item of
model A is ₹15 and on an item of model B is ₹10. How many of items of each model
should be made per day in order to maximize daily profit ? Formulate the above LPP
and solve it graphically and find the maximum profit.
solutions

solutions



2019
Q1
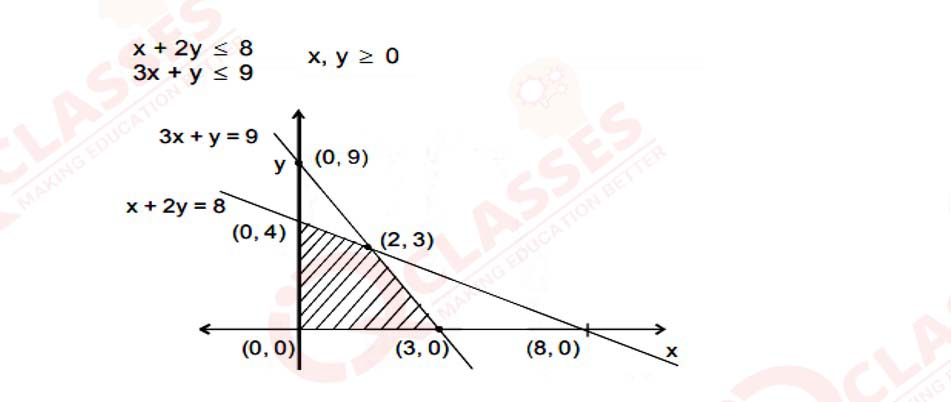
A company produces two types of goods, A and B, that require gold and silver. Each
unit of type A requires 3 g of silver and 1 g of gold while that of B requires 1 g of
silver and 2 g of gold. The company can use atmost 9 g of silver and 8 g of gold. If
each unit of type A brings a profit of ₹40 and that of type B ₹50, find the number of
units of each type that the company should produce to maximize the profit. Formulate
and solve graphically the LPP and find the maximum profit.
solutions

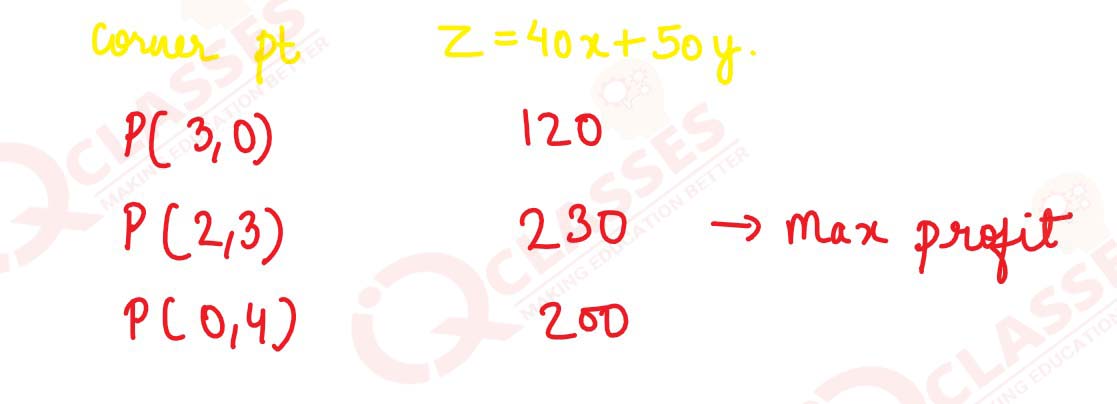
solutions



2020
Q1
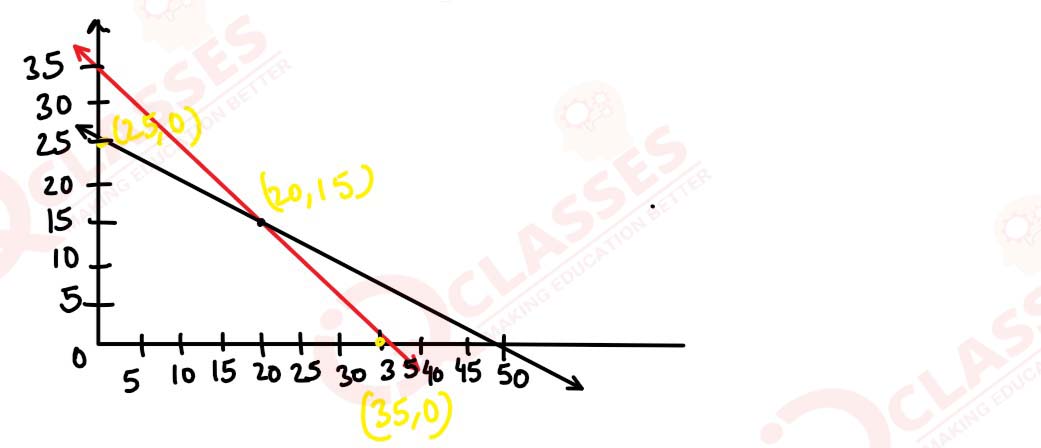
A furniture trader deals in only two items — chairs and tables. He has
₹50,000 to invest and a space to store at most 35 items. A chair costs
him ₹1,000 and a table costs him ₹2,000. The trader earns a profit of
₹150 and ₹250 on a chair and table, respectively. Formulate the above
problem as an LPP to maximise the profit and solve it graphically.
solutions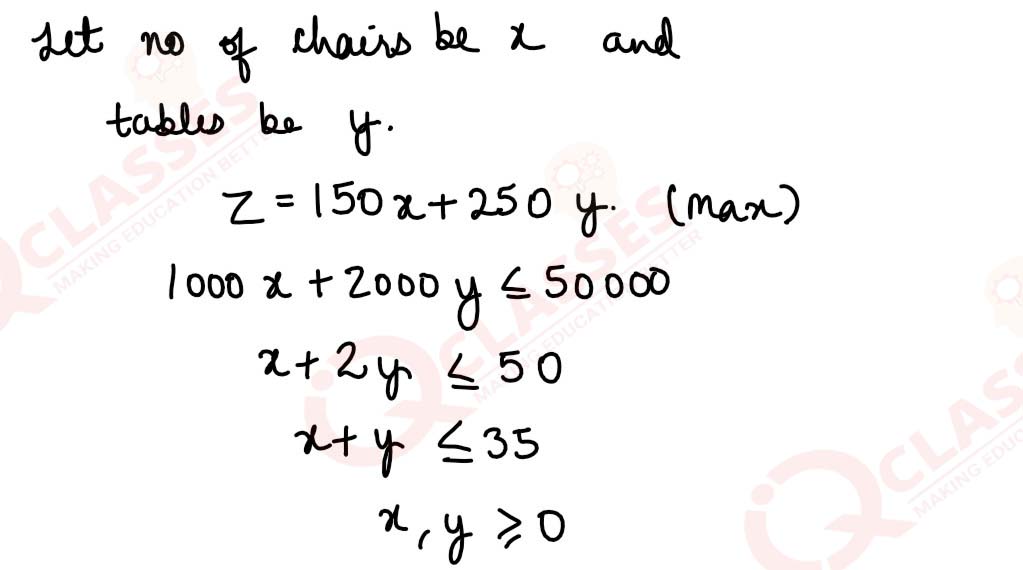

solutions
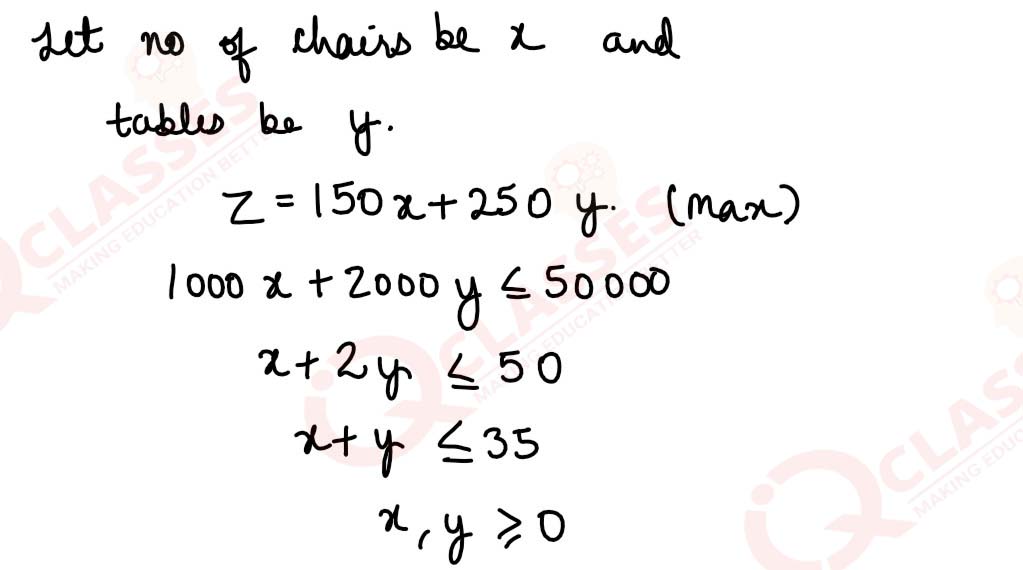


Q2
The corner points of the feasible region determined by the system of
linear inequalities are (0, 0), (4, 0), (2, 4) and (0, 5). If the maximum value
of z = ax + by, where a, b > 0 occurs at both (2, 4) and (4, 0), then
(A) a = 2b
(B) 2a=b
(C) a=b
(D) 3a=b
solutions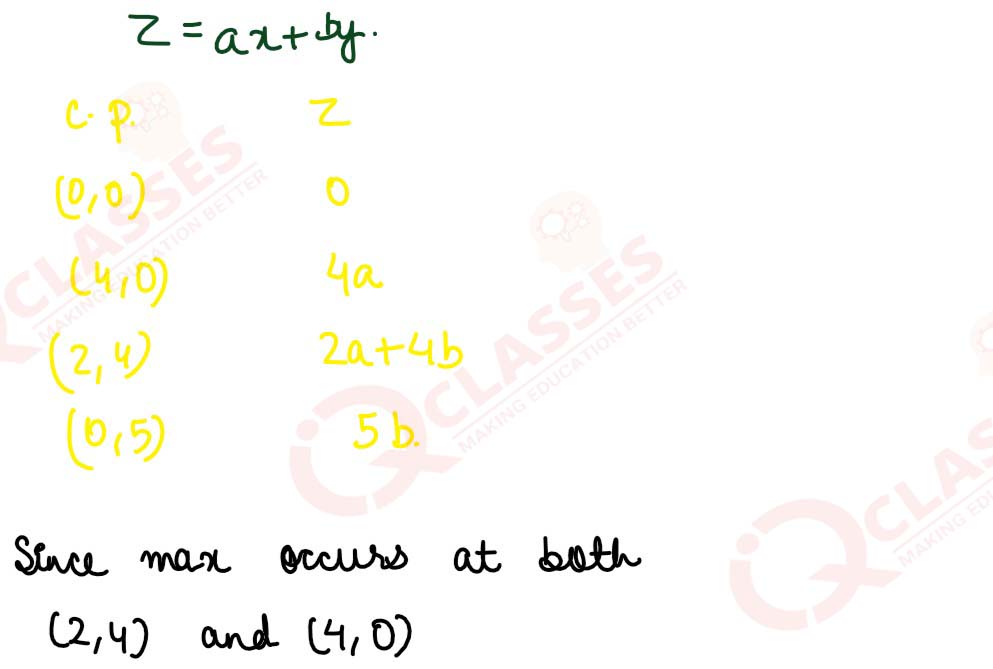

(A) a = 2b
(B) 2a=b
(C) a=b
(D) 3a=b
solutions
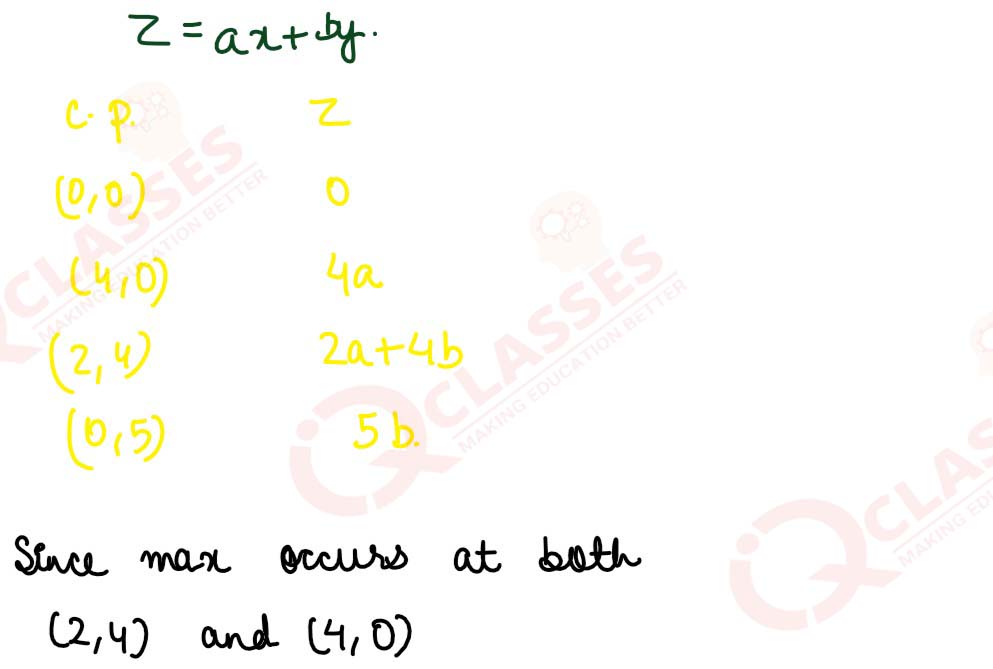


Reach Us
SERVICES
- ACADEMIC
- ON-LINE PREPARATION
- FOUNDATION & CRASH COURSES
CONTACT
B-54, Krishna Bhawan, Parag Narain Road,
Near Butler Palace Colony Lucknow
Contact:+918081967119

Add a comment