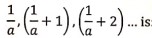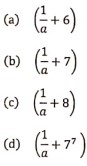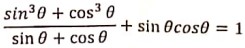Maximum Marks: 80
Time allowed: Two and half hours
Answers to this Paper must be written on the paper provided separately.
You will not be allowed to write during first 15 minutes.
This time is to be spent in reading the question paper.
The time given at the head of this Paper is the time allowed for writing the answers.
Attempt all questions from Section A and any four questions from Section B.
All working, including rough work, must be clearly shown, and must be done on the same sheet as
the rest of the answer.
Omission of essential working will result in loss of marks.
The intended marks for questions or parts of questions are given in brackets [ ]
Mathematical tables are provided.
Section-A
(Attempt all questions from this Section.)
Question 1
Choose the correct answers to the questions from the given options.
(Do not copy the questions, write the correct answers only.)
(i) For an Intra-state sale, the CGST paid by a dealer to the Central government ts
Rs 120. If the marked price of the article is Rs 2000, the rate of GST is:
(a) 6%
(b) 10%
(c) 12%
(d) 16.67%
Solution
View Solution
(ii) What must be subtracted from the polynomial x3 + x2 - 2x + 1, so that the result is
exactly divisible by (x - 3)?
(a) -31
(b) -30
(c) 30
(d) 31
Solution
View Solution
(iii) The roots of the quadratic equation px2 - qx + r = 0 are real and equal if:
(a) p2 = 4qr
(b) q2 = 4qr
(c) -q2 = 4qr
(d) p2 > 4qr
Solution
View Solution
(iv) If matrix A = 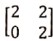 and A2 =
and A2 = 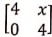 then the value of x is:
then the value of x is:
(a) 2
(b) 4
(c) 8
(d) 10
Solution
View Solution
(v) The median of the following observations arranged in ascending order is 64.
Find the value of x:
27, 31, 46, 52, x, x+4, 71, 79, 85. 90
(a) 60
(b) 61
(c) 62
(d) 66
Solution
View Solution
(vi) Points A (x, y), B (3, -2) and C (4, -5) are collinear, The value of y in terms of x is:
(a) 3x-11
(b) 11-3x
(c) 3x-7
(d) 7-3x
Solution
View Solution
(vii) The given table shows the distance covered and the time taken by a train moving
at a uniform speed along a straight track.

The values of x and y are:
(a) x= 4, y = 150
(b) x =3,y = 100
(c) x = 4, y = 100
(d) x=3,y= 150
Solution
View Solution
(viii) The 7" term of the given Arithmetic Progression (A.P.):
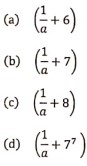
Solution
View Solution
(ix) The sum invested to purchase 15 shares of a company of nominal value Rs 75 available
at a discount of 20% is:
(a) Rs 60
(b) Rs 90
(c) Rs 1350
(d) Rs 900
Solution
View Solution
(x) The circumcentre of a triangle is the point which is:
(a) at equal distance from the three sides of the triangle.
(b) at equal distance from the three vertices of the triangle.
(c) the point of intersection of the three medians
(d) the point of intersection of the three altitudes of the triangle.
Solution
View Solution
(xi) Statement 1: sin2 θ + cos2 θ = 1
Statement 2: cosec2 θ + cot2 θ = 1
Which of the following is valid ?
(a) only 1
(b) only 2
(c) both 1 and 2
(d) neither 1 nor 2
Solution
View Solution
(xii) In the given diagram, PS and PT are the tangents to the circle. SQ || PT and
∠SPT = 80°. The value of ∠QST is:
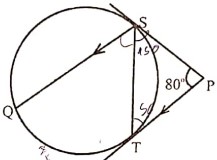
(a) 140°
(b) 90°
(c) 80°
(d) 50°
Solution
View Solution
(xiii) Assertion (A): A die is thrown once and the probability of getting an even
number is 2/3.
Reason (R): The sample space for even numbers on a die is {2, 4, 6}
(a) A is true, R is false.
(b) A is false, R is true.
(c) Both A and R are true.
(d) Both A and R and false.
Solution
View Solution
(xiv) A rectangular sheet of paper of size 11 cm x 7 cm Is first rotated about the side
11 cm and then about the side 7 cm to form a cylinder, as shown in the diagram.
The ratio of their curved surface areas is:
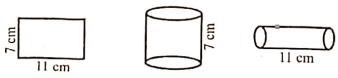
(a) 1:1
(b) 7:11
(c) 11:7
(d) 11π/7 : 7π/11
Solution
View Solution
(xv) In the given diagram, ▵ABC ~ ▵PQR. If AD and PS are bisectors of ∠BAC and
∠OPR respectively then:

(a) ▵ABC ~ ▵PQS
(b) ▵ABD ~ ▵PQS
(c) ▵ABD ~ ▵PSR
(d) ▵ABC~ ▵PSR
Solution
View Solution
Question 2
(i) A =

Find the values of x and y, if AB = C.
(ii) A solid metallic cylinder is cut into two identical halves along its height (as shown [4
in the diagram). The diameter of the cylinder is 7 em and the hei ght is 10 cm. Find:
(a) The total surface area (both the halves).
(b) The total cost of painting the two halves at the rate of Rs 30 per cm2 [Use π=22/7]

(iii) 15, 30, 60, 120... are in G.P. (Geometric Progression).
(a) Find the nth term of this G.P. in terms of n.
(b) How many terms of the above G.P. will give the sum 945?
Solution
View Solution
Question 3
(i) Factorize: sin3θ + cos3θ
Hence, prove the following identity:
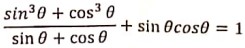
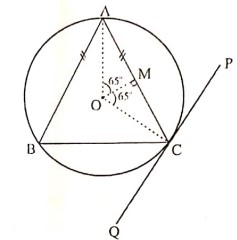
(ii) In the given diagram, O is the centre of the circle. PR and PT are two tangents
drawn from the external point P and touching the circle at Q and S respectively. MN is a diameter of circle . Given ∠PQM = 42o and ∠PSM = 25o

Find :
(a) ∠ OQM
(b) ∠ QNS
(c) ∠ QOS
(d) ∠ QMS
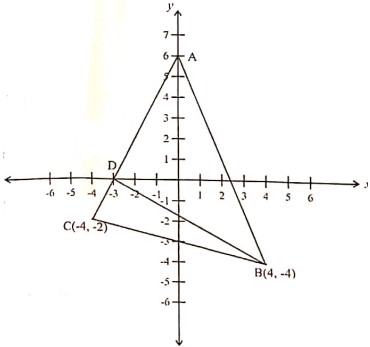
(iii) Use graph sheet for this question. Take 2 cm = 1 unit along the axes.
(a) Plot A(O, 3), B(2, 1) and C(4, -1).
(b) Reflect point B and C in y-axis and name their images as B' and C'
respectively. Plot and write coordinates of the points B' and C'.
(c) Reflect point A in the line BB' and name its images as A'.
(d) Plot and write coordinates of point A'.
(c) Join the points ABA'B' and give the geometrical name of the closed figure so
formed.
Solution
View Solution
Section-B
(Attempt any four questions from this Section.)
Question 4
(i) Suresh has a recurring deposit account in a bank. He deposits Rs 2000 per month and the [3
bank pays interest at the rate of 8% per annum. If he gets Rs 1040 as interest at the time of
maturity, find in years total time for which the account was held.
(ii) The following table gives the duration of movies in minutes.
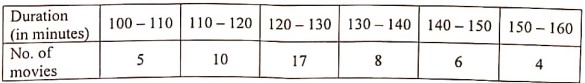
Using step - deviation method, find the mean duration of the movies.
(iii) If

(a) Find (a+b)/(a-b)
(b) Hence using properties of proportion, find a: b,
Solution
View Solution
Question 5
(i) The given graph with a histogram represents the number of plants of different heigh
grown in a school campus. Study the graph carefully and answer the following questions
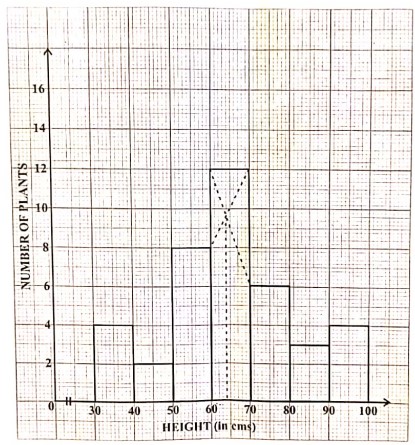
(a) Make a frequency table with respect to the class boundaries and their corresponding
frequencies.
(b) State the modal class.
(c) Identify and note down the mode of the distribution.
(d) Find the number of plants whose height range is between 80 cm to 90 cm.
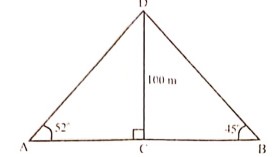
(ii) The angle of elevation of the top of a 100 m high tree from two points A and B on the opposite side of the tree are 52° and 45° respectively.Find the distance AB to the nearest meter.
Solution
View Solution
Question 6
(i) Solve the following quadratic equation for x and give your answer correct to three significant figures: 2x2 - 10x + 5 = 0 (Use mathematical tables if necessary)
(ii) The nth term of an Arithmetic Progression (A.P.) is given by the relation Tn = 6(7 - n).
Find:
(a) its first term and common difference
(b) sum of its first 25 terms
(iii) In the given diagram ▵ADB and ▵ACB are two right angled triangles with
∠ADB = ∠BCA = 90°. If AB = 10 cm, AD = 6 cm, BC = 2.4 cm and DP = 4.5 cm
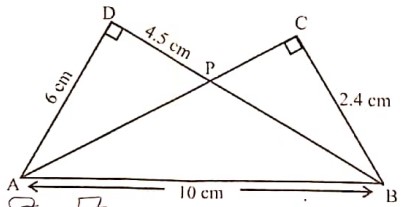
(a) Prove that ▵APD ~ ▵BPC
(b) Find the length of BD and PB
(c) Hence, find the length of PA
(d) Find area ▵APD : area ▵BPC
Solution
View Solution
Question 7
Question 8
(i) The polynomial 3x3 + 8x2 - 15x + k has (x - 1) as a factor. Find the value of k, Hence
factorize the resulting polynomial completely,
(ii) The following letters A, D, M, N, O, S, U, Y of the English alphabet are written on separate
cards and put in a box. The cards are well shuffled and one card is drawn at random. What
is the probability that the card drawn is a letter of the word,
(a) MONDAY?
(b) which does not appear in MONDAY?
(c) which appears both in SUNDAY and MONDAY?
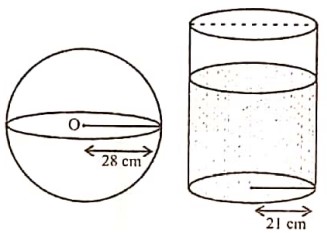
(iii) Oil is stored in a spherical vessel occupying 3/4 of its full capacity. Radius of this spherical
vessel is 28 cm. This oil is then poured into a cylindrical vessel with a radius of 21 cm.
Find the height of the oil in the cylindrical vessel (correct to the nearest cm). [Take π = 22/7]
Solution
View Solution
Question 9
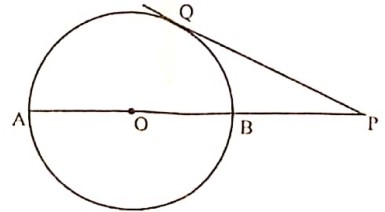
(i) The figure shows a circle of radius 9 cm with O as the centre. The diameter AB produced
meets the tangent PQ at P. If PA = 24 cm, find the length of tangent PQ,
(ii) Mr. Gupta invested Rs 33000 in buying 100 shares of a company at 10% premium. The
dividend declared by the company is 12%. Find:
(a)
the number of shares purchased by him.
(b) his annual dividend.
(iii) A lite insurance agent found the following data for distribution of ages of 100 policy
holders:
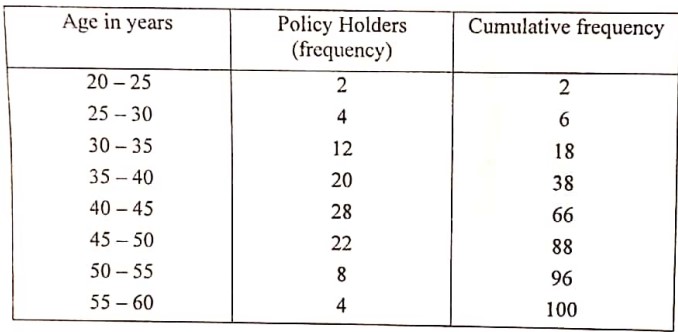
On a graph sheet draw an ogive using the given data. Take 2 cm = 5 years along one axis
and 2 cm = 10 policy holders along the other axis. Use your graph to find:
(a) The median age.
(b) Number of policy holders whose age is above 52 years.
Solution
View Solution
Question 10
(i) Rohan bought the following eatables for his friends :
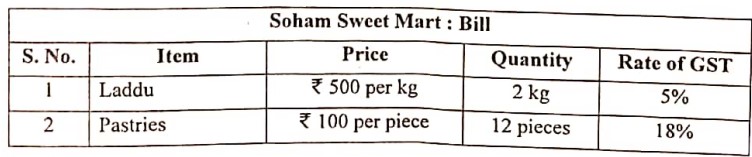
Calculate :
(a) Total GST paid.
(b) Total bill amount including GST.
(ii) (a) If the lines kx - y + 4 = 0 and 2y = 6x + 7 are perpendicular to each other, find the
value of k.
(b) Find the equation of a line parallel to 2y = 6x + 7 and passing through (-1, 1)
(iii) Use ruler and compass to answer this question. Construct ∠ABC = 90°, where
AB =6cm, BC =8 cm.
(a) Construct the locus of points equidistant from B and C.
(b) Construct the locus of points equidistant from A and B.
(c) Mark the point which satisfies both the conditions (a) and (b) as O. Construct the
locus of points keeping a fixed distance OA from the fixed point O.
(d) Construct the locus of points which are equidistant from BA and BC.
Solution
View Solution
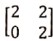 and A2 =
and A2 = 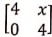 then the value of x is:
then the value of x is: